Pre-Calculus : GEOMETRIC SEQUENCE
Summary
TLDRThis lesson delves into geometric sequences and series, explaining how to determine the common ratio and calculate terms using the nth term formula. It introduces the concept of the geometric mean and its calculation between two terms. The lesson further explores the geometric series, providing formulas for the sum of a finite series and an infinite series, highlighting their applications in various fields. Through practical examples, students learn to apply these concepts, enhancing their understanding of this essential mathematical topic.
Takeaways
- 😀 A geometric sequence consists of terms formed by multiplying the previous term by a constant called the common ratio.
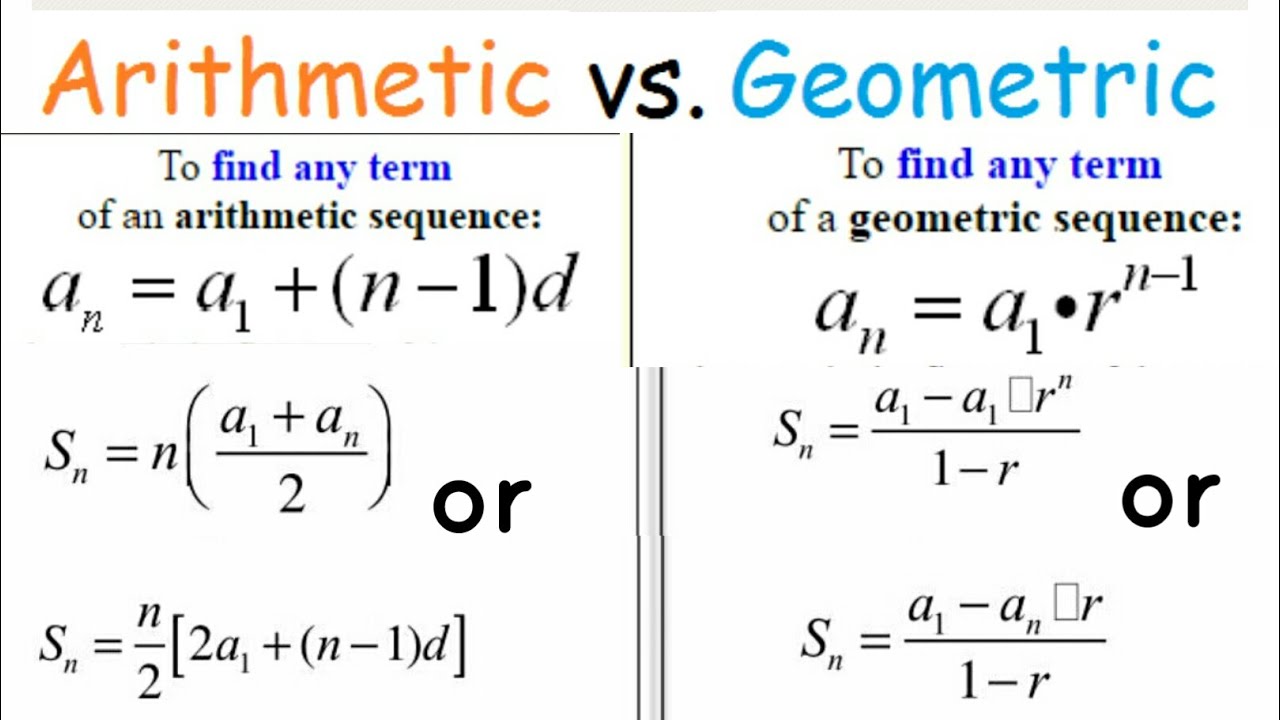
- 📏 The formula for the nth term of a geometric sequence is a_n = a_1 × r^(n-1), where a_1 is the first term and r is the common ratio.
- ➗ The common ratio (r) can be determined by dividing any term in the sequence by its preceding term.
- 📊 The geometric mean between two numbers can be calculated using the formula: √(a × b).
- 🔢 In a geometric series, the sum of the first n terms can be found using the formula S_n = a_1 × (r^n - 1) / (r - 1).
- 💡 For infinite geometric series, where |r| < 1, the sum can be calculated using S_∞ = a_1 / (1 - r).
- 🧮 An example of a geometric sequence is 1, 3, 9, 27, which has a common ratio of 3.
- 🔍 The geometric mean can also be applied to find means between other terms in a geometric sequence.
- 🏗️ The concept of geometric series is applicable in real-world situations, such as calculating distances traveled by objects in motion, like a pendulum.
- 📚 Understanding geometric sequences and series is essential for solving various mathematical problems and applications in different fields.
Q & A
What defines a geometric sequence?
-A geometric sequence is defined as a sequence in which each succeeding term is obtained by multiplying the previous term by a fixed number known as the common ratio.
How is the common ratio calculated in a geometric sequence?
-The common ratio can be calculated by dividing any term in the sequence by the previous term, such as dividing the second term by the first term.
What is the formula for finding the nth term of a geometric sequence?
-The formula for the nth term of a geometric sequence is given by a_n = a_1 * r^(n-1), where a_n is the nth term, a_1 is the first term, r is the common ratio, and n is the term number.
What is a geometric mean?
-The geometric mean is a term that lies between two terms in a geometric sequence, calculated as the square root of the product of the two terms.
How can the geometric mean be calculated using the terms 2 and 8?
-To find the geometric mean of 2 and 8, you multiply the two terms (2 * 8 = 16) and then take the square root of the result, giving you 4.
What is a geometric series?
-A geometric series is the sum of the terms in a geometric sequence.
What is the formula for the sum of the first n terms of a geometric sequence?
-The formula for the sum of the first n terms (S_n) of a geometric sequence is S_n = a_1 * (r^n - 1) / (r - 1), where a_1 is the first term, r is the common ratio, and n is the number of terms.
What is the infinite geometric series, and how is it calculated?
-An infinite geometric series is the sum of the terms of a geometric sequence that continues indefinitely. It can be calculated using the formula S_infinity = a_1 / (1 - r), where a_1 is the first term and r is the common ratio (|r| < 1).
In the example given, what was the common ratio for the pendulum swings?
-In the example of the pendulum swings, the common ratio was two-thirds (2/3) of the length of the previous swing.
What was the total distance traveled by the pendulum before coming to rest?
-The total distance traveled by the pendulum before coming to rest can be calculated as an infinite geometric series, resulting in a sum of 30 decimeters.
Outlines

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraMindmap

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraKeywords

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraHighlights

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraTranscripts

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahora5.0 / 5 (0 votes)