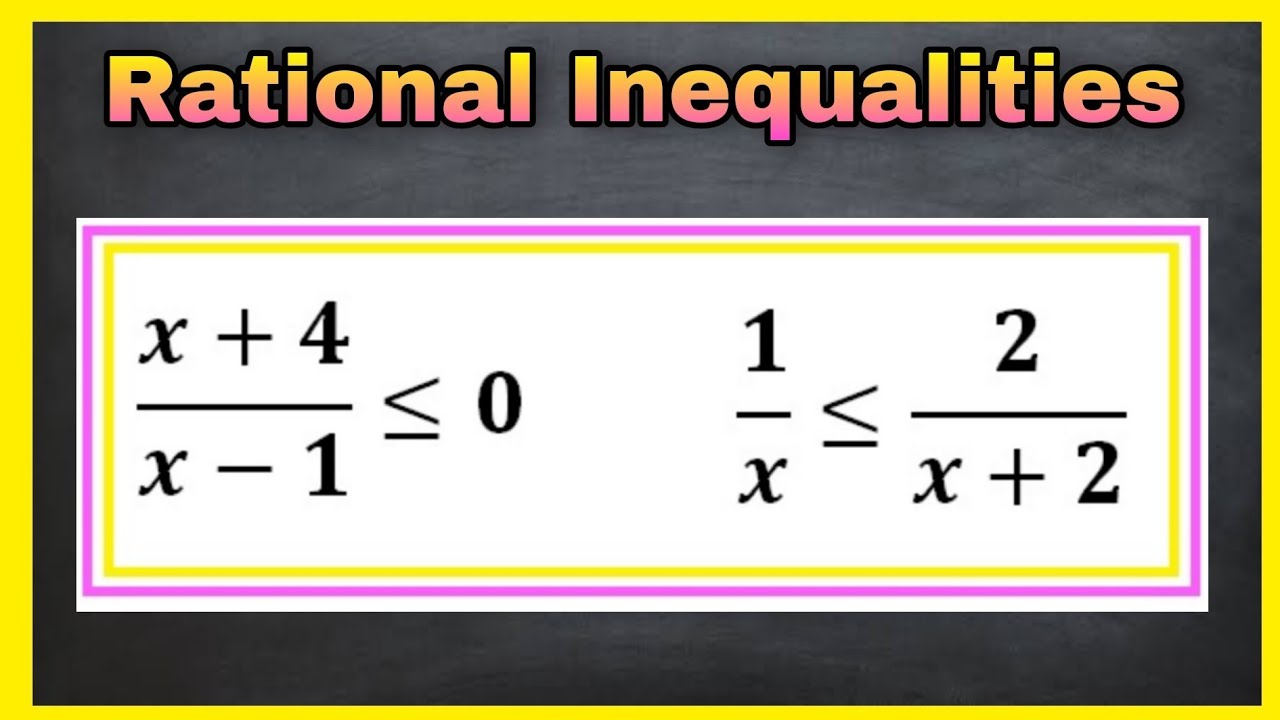Solving a Rational Inequality - Example 1
Summary
TLDRThis educational video script outlines the process of solving rational inequalities, which are fractions involving polynomials. The presenter emphasizes converting the left side to a single fraction and setting the numerator and denominator to zero to find critical values. These values are plotted on a number line, and intervals are tested to determine where the inequality holds. The example given involves the inequality (2x-4)/(6x+18) ≤ 0, with solutions found between -3 and 2, excluding -3 due to an undefined expression.
Takeaways
- 📉 The process starts by writing the left side of the rational inequality as a single fraction, making one side zero.
- 📝 The numerator and denominator of the rational inequality need to be set equal to zero and solved separately.
- 🧮 Once the solutions for the numerator and denominator are found, the next step is to plot them on a number line.
- ⛔ Numbers that make the denominator zero are undefined and cannot be solutions (open circles on the number line).
- ✅ Solutions for the numerator, like x = 2, can be valid if the expression evaluates correctly (closed circles on the number line).
- 🔢 Test points are selected from different intervals of the number line to check which values satisfy the inequality.
- 📉 When a test point results in a positive fraction, it doesn't satisfy the inequality since it must be less than or equal to zero.
- ✅ Test points that produce negative results in the inequality satisfy the condition (e.g., x = 0 gives a negative result).
- 🔎 Numbers between negative 3 and 2 satisfy the inequality, and these are the valid solutions.
- ✏️ The final solution is expressed in interval notation as (-3, 2], including 2 but excluding -3.
Q & A
What is the first step when solving a rational inequality?
-The first step is to express one side of the inequality as a single fraction and set the other side to zero. This simplifies the inequality for further calculations.
What does it mean when the script refers to a 'rational inequality'?
-A rational inequality is an inequality involving a fraction where both the numerator and the denominator are polynomials. The goal is to determine where the expression is greater than or less than zero.
How do you handle the numerator and denominator in a rational inequality?
-You set the numerator equal to zero to find critical points and solve it. Similarly, set the denominator equal to zero, as this will help identify points where the expression is undefined (i.e., vertical asymptotes).
Why do we need to plot critical points on a number line?
-Critical points, where the numerator or denominator equals zero, divide the number line into intervals. These intervals help test the behavior of the rational function within each range to determine where the inequality holds.
What happens when you plug a value into the denominator and get zero?
-If plugging a value into the denominator results in zero, the expression is undefined at that point. Therefore, the inequality cannot be satisfied, and this point is excluded from the solution.
Why is x = 2 a solution in this inequality?
-When x = 2 is plugged into the numerator, the result is zero. Zero divided by any non-zero number is zero, and since the inequality is 'less than or equal to zero,' x = 2 satisfies the inequality.
How do you determine which intervals satisfy the inequality?
-After plotting the critical points on the number line, you test points from each interval by plugging them into the original inequality. If the inequality holds true, that interval is part of the solution.
What does it mean when a tested point gives a negative value in the inequality?
-If a tested point gives a negative value, and the inequality requires the expression to be less than or equal to zero, this means the inequality is satisfied for that interval.
Why does the solution include numbers between -3 and 2?
-Testing points within the interval between -3 and 2 showed that the inequality was satisfied in this range. Outside of this interval, the inequality does not hold.
What is the final answer to the inequality in interval notation?
-The solution is the interval (-3, 2], where -3 is excluded (since the expression is undefined) and 2 is included (since it satisfies the inequality).
Outlines

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraMindmap

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraKeywords

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraHighlights

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraTranscripts

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahora5.0 / 5 (0 votes)