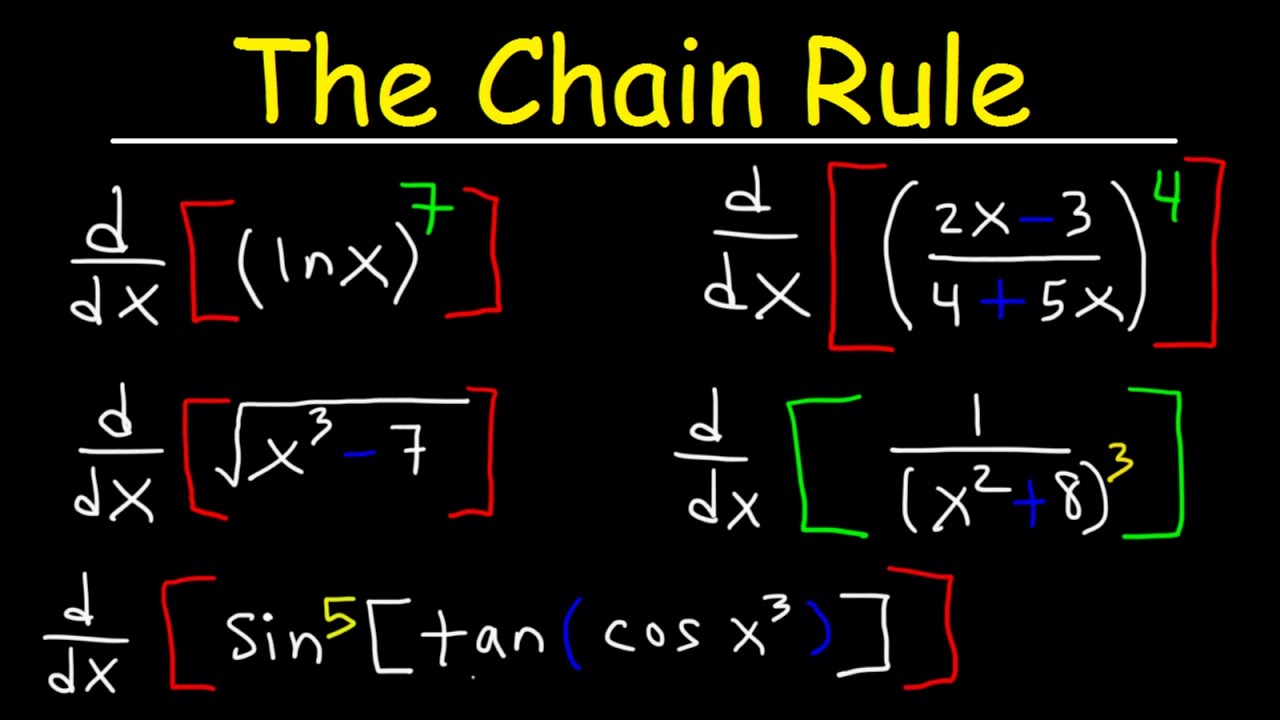
The Chain Rule for Finding Derivatives | Chain Rule | Basic Calculus

Summary

This educational video provides a clear explanation of how to use the chain rule to find derivatives of composite functions. It walks through six examples, demonstrating the process of identifying the outer and inner functions, applying the chain rule, and simplifying the results. The examples range from basic to more complex functions, such as those involving powers and roots, offering viewers a comprehensive understanding of derivative calculations.

Takeaways

• 📚 The video is a tutorial on using the chain rule to find derivatives of composite functions.
• 🔍 The chain rule formula is given as the derivative of h(x) = g(f(x)) equals the derivative of g at f(x) times the derivative of f(x).
• 📝 The concept of 'inner' and 'outer' functions is introduced to help understand the composite function structure.
• 🔢 The first example demonstrates finding the derivative of a function involving a cubic term and a quadratic term inside a parenthesis.
• 📉 The second example shows the derivative of a function with a square root and a negative exponent, illustrating the power rule.
• 📈 The third example involves a function with a term raised to a fractional exponent, emphasizing the chain rule's application.
• 📌 The fourth example explains the derivative of a function with a square root, highlighting the use of the chain rule with exponents.
• 🔑 The fifth example covers the derivative of a cube root function, showing the simplification process using the chain rule.
• 📐 The sixth and final example in the script deals with the derivative of a function containing a square root of a quadratic expression.
• 👨‍🏫 The presenter, Prof D, encourages viewers to ask questions or seek clarifications in the comments section if needed.
• 👋 The video concludes with a sign-off from Prof D, indicating the end of the tutorial.

Q & A

What is the main topic of the video?

The main topic of the video is the application of the chain rule in calculus to find the derivative of composite functions.

What is the chain rule in derivatives?

The chain rule states that the derivative of a composite function h(x) = g(f(x)) is h'(x) = g'(f(x)) · f'(x), where g'(x) is the derivative of the outer function and f'(x) is the derivative of the inner function.

What is the first example given in the video to demonstrate the chain rule?

The first example is to find the derivative of f(x) = (5x^2 - 4x + 2)^3.

How is the derivative of the first example simplified using the chain rule?

The derivative is simplified by applying the chain rule to the outer function and the power rule to the inner function, resulting in 30x(5x^2 - 4x + 2)^2 - 12(5x^2 - 4x + 2).

What is the second example in the video, and how is the derivative found?

The second example is f(x) = (3x^2 - 4)^{1/2}. The derivative is found by applying the chain rule with the outer function being the square root and the inner function being 3x^2 - 4, resulting in 3x / √(3x^2 - 4).

What is the third example provided, and what is its derivative?

The third example is f(x) = 5x^4 - 7^{2/3}. The derivative is found by applying the chain rule to both terms, resulting in 40x^3 / 3(5x^4 - 7)^{1/3}.

In the fourth example, what is the function and its derivative?

The fourth example is f(x) = √(5x - 2). The derivative is 5 / 2√(5x - 2).

What is the function and its derivative in the fifth example?

The fifth example is f(x) = ∛(3x + 5). The derivative is 1 / 3(3x + 5)^{2/3}.

What is the sixth example in the video, and how is its derivative simplified?

The sixth example is f(x) = √(15x^2 - 6x + 2). The derivative is simplified by applying the chain rule and the power rule, resulting in (15x - 3) / 2√(15x^2 - 6x + 2).

What does the video suggest to do if viewers have questions or need clarifications?

The video suggests that viewers should leave their questions or requests for clarifications in the comment section below.