Geometric Series and Geometric Sequences - Basic Introduction
Summary
TLDRThis educational video script explores the concepts of geometric sequences and series, distinguishing them from arithmetic ones by their common ratio versus common difference. It explains how to calculate the nth term and the partial sum of a geometric series, highlighting the formulae involved. The script also discusses the arithmetic and geometric means, provides examples of writing equations between terms, and covers the sum of infinite geometric series, emphasizing the convergence criteria. Practice problems are included to reinforce the concepts.
Takeaways
- 📚 The difference between a geometric sequence and a series is that a geometric sequence is a list of numbers with a common ratio between terms, while a geometric series is the sum of the numbers in a geometric sequence.
- 🔢 In a geometric sequence, each term is found by multiplying the previous term by a common ratio, whereas in an arithmetic sequence, each term is found by adding a common difference to the previous term.
- 🧮 The formula to find the nth term of a geometric sequence or series is \( a_n = a_1 \times r^{(n-1)} \), where \( a_1 \) is the first term and \( r \) is the common ratio.
- 📈 The partial sum formula for a geometric series is \( S_n = a_1 \times \frac{1 - r^n}{1 - r} \), which calculates the sum of the first \( n \) terms.
- ⚠️ The sum of an infinite geometric series can only be calculated if the series converges, which occurs when the absolute value of the common ratio \( r \) is less than 1.
- 🔁 The sum of an infinite geometric series that converges is given by \( S_{\infty} = \frac{a_1}{1 - r} \), where \( a_1 \) is the first term and \( r \) is the common ratio.
- 🔢 The arithmetic mean (Ma) of two numbers in an arithmetic sequence is the average, while the geometric mean (Mg) of two numbers is the square root of their product.
- 📉 To relate terms within a geometric sequence, multiply the previous term by the common ratio raised to the power of the term's position difference.
- 📝 When identifying a sequence or series, look for a common ratio (geometric) or common difference (arithmetic), and determine if it is finite or infinite based on whether it has an end or continues indefinitely.
- 📊 Practice problems in the script illustrate how to calculate terms of a geometric sequence, write general formulas, and determine the type of sequence or series based on given patterns.
- 🤓 Understanding the properties of geometric sequences and series is essential for solving problems involving series convergence, term calculation, and sum determination.
Q & A
What is the main focus of the video?
-The video focuses on geometric sequences and series, explaining the difference between them, how to identify them, and how to calculate various terms and sums within these sequences and series.
What distinguishes a geometric sequence from an arithmetic sequence?
-A geometric sequence is distinguished by a common ratio between consecutive terms, whereas an arithmetic sequence has a common difference between terms. In a geometric sequence, each term is found by multiplying the previous term by the common ratio.
How is the common ratio of a geometric sequence calculated?
-The common ratio is calculated by dividing any term in the sequence by the previous term. For example, if the sequence is 3, 6, 12, ..., the common ratio is 2, since 6 divided by 3 equals 2.
What is the formula to find the nth term of a geometric sequence or series?
-The formula to find the nth term of a geometric sequence or series is given by \( a_n = a_1 \times r^{(n-1)} \), where \( a_1 \) is the first term, \( r \) is the common ratio, and \( n \) is the term number.
How do you calculate the sum of the first n terms of a geometric series?
-The sum of the first n terms of a geometric series is calculated using the formula \( S_n = a_1 \times \frac{1 - r^n}{1 - r} \), where \( S_n \) is the sum, \( a_1 \) is the first term, \( r \) is the common ratio, and \( n \) is the number of terms.
What is an infinite geometric series and when does it converge?
-An infinite geometric series is a series that continues indefinitely. It converges when the absolute value of the common ratio \( r \) is less than 1, meaning the terms get smaller and smaller, allowing the series to sum to a finite value.
How is the sum of an infinite geometric series calculated?
-The sum of an infinite geometric series is calculated using the formula \( S_{\infty} = \frac{a_1}{1 - r} \), where \( a_1 \) is the first term and \( r \) is the common ratio, provided that the absolute value of \( r \) is less than 1.
What is the difference between the arithmetic mean and the geometric mean of two numbers?
-The arithmetic mean of two numbers is the average, found by adding the numbers and dividing by 2. The geometric mean is the square root of the product of the two numbers, reflecting the middle term in a geometric sequence.
How can you determine if a sequence is arithmetic or geometric by looking at its terms?
-A sequence is arithmetic if there is a common difference between consecutive terms, while it is geometric if there is a common ratio by which you multiply one term to get the next.
What is the relationship between the terms in a geometric sequence defined by a recursive formula?
-In a geometric sequence defined by a recursive formula, each term is found by multiplying the previous term by the common ratio, represented as \( a_n = r \times a_{n-1} \).
Can you provide an example of how to write the first five terms of a geometric sequence given the first term and the common ratio?
-Certainly. If the first term \( a_1 \) is 2 and the common ratio \( r \) is 3, the first five terms would be calculated as follows: \( a_1 = 2 \), \( a_2 = 2 \times 3 = 6 \), \( a_3 = 6 \times 3 = 18 \), \( a_4 = 18 \times 3 = 54 \), and \( a_5 = 54 \times 3 = 162 \).
Outlines

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenMindmap

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenKeywords

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenHighlights

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenTranscripts

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenWeitere ähnliche Videos ansehen
Arithmetic Sequences and Arithmetic Series - Basic Introduction
MATH 10 : DIFFERENTIATING GEOMETRIC SEQUENCE FROM AN ARITHMETIC SEQUENCE (Taglish)
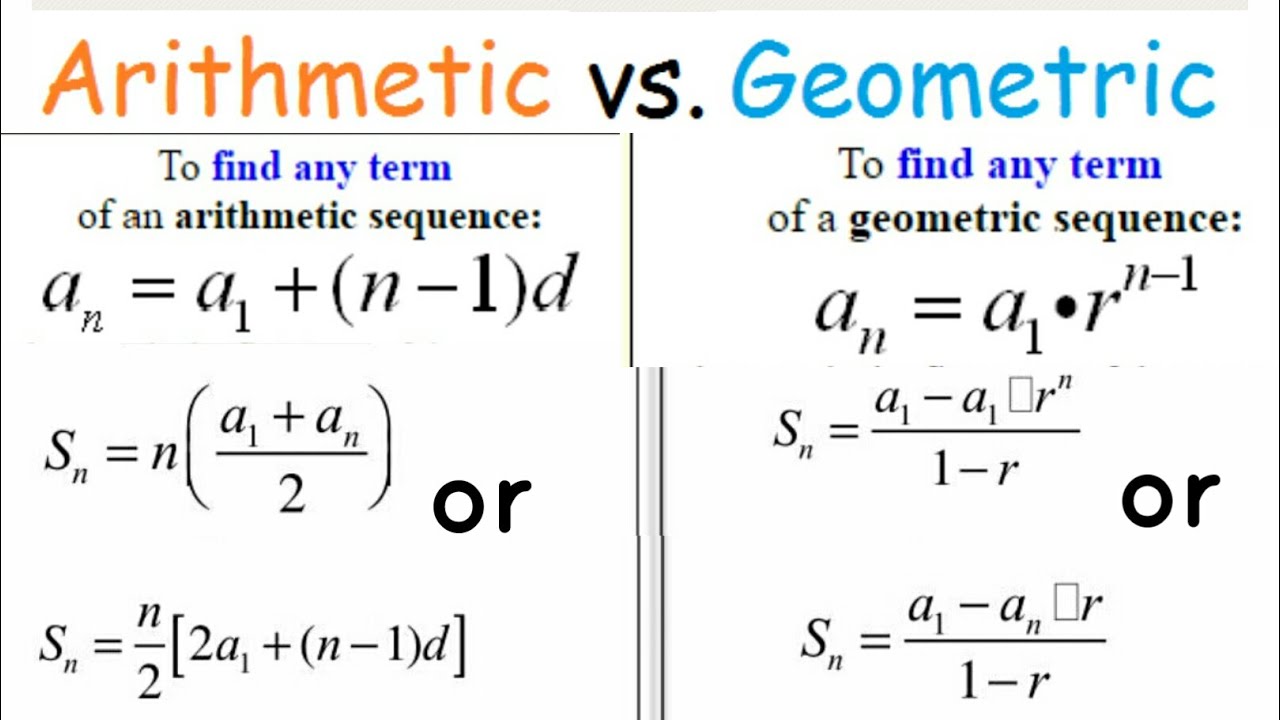
Arithmetic Sequence vs Geometric Sequence

GCSE Maths - Types of Number Sequences - Arithmetic vs Geometric
Introduction to Sequences

Pembahasan BARISAN DAN DERET (Aritmetika & Geometri) KELAS 11 | #MatematikAsik
5.0 / 5 (0 votes)
