Pembahasan Soal-Soal Gerak Parabola || Fisika Kelas X || #OprekMafiki

Summary

This educational video explains the concepts of projectile motion through the analysis of several problems. The presenter covers topics such as the trajectory of a projectile, the maximum range, and how to calculate various elements like initial velocity, time to reach the highest point, and horizontal distance. Using specific examples, the video demonstrates how to apply key formulas and concepts, including the use of sine and cosine for different angles. The solutions to the problems are clearly worked out, helping viewers understand the process of solving parabolic motion problems step by step.

Takeaways

• 😀 The video discusses projectile motion, focusing on parabolic movement.
• 😀 The first problem involves calculating the horizontal distance of a projectile shot at a 15° angle with a velocity of 50 m/s.
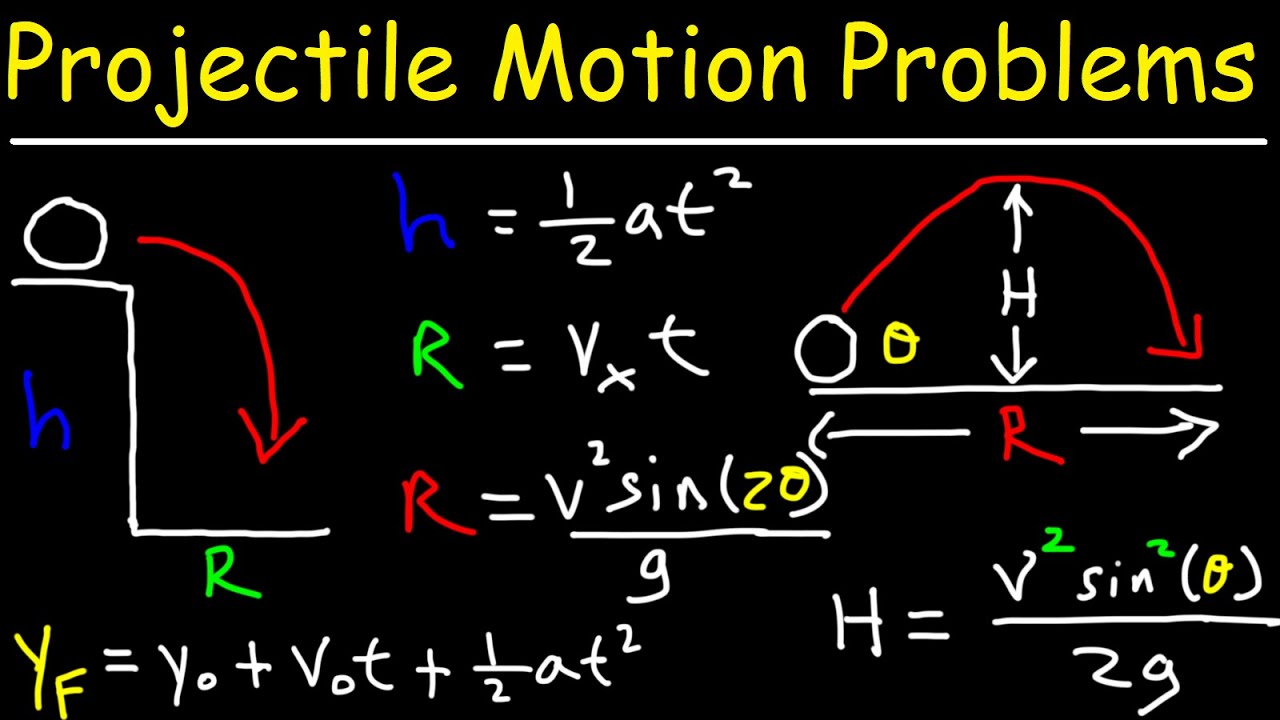
• 😀 The formula used for the horizontal range is X_max = (v^2 * sin(2α)) / g.
• 😀 The result of the first problem is that the horizontal distance covered by the projectile is 125 meters.
• 😀 The second problem involves a stone thrown at a 37° angle that reaches its highest point in 3 seconds.
• 😀 The horizontal distance covered by the stone during the 3-second interval is calculated to be 120 meters.
• 😀 In the third problem, a bullet is fired at a 30° angle, and its position at a certain point is given as (720√3, 0).
• 😀 The problem asks to determine the initial velocity of the projectile, and the formula used is X_max = (v0^2 * sin(2α)) / g.
• 😀 The initial velocity of the bullet is calculated to be 120 m/s.
• 😀 The video emphasizes understanding projectile motion equations and applying them to solve real-world problems related to trajectory and distance.

Q & A

What is the main topic discussed in the video?

The video discusses projectile motion, specifically the concept of parabolic motion and how to solve related problems using basic physics principles.

What is the formula used to calculate the maximum horizontal distance in projectile motion?

The formula used is X_max = (v0^2 * sin(2 * Alfa)) / g, where v0 is the initial velocity, Alfa is the launch angle, and g is the acceleration due to gravity.

In the first example, what is the horizontal distance traveled by the projectile?

The horizontal distance (X_max) is calculated to be 125 meters, based on the initial velocity of 50 m/s and an angle of 15°.

How do we calculate the time taken to reach the highest point of projectile motion?

The time to reach the highest point is calculated using the formula t = (v0 * sin(Alfa)) / g, where v0 is the initial velocity and Alfa is the launch angle.

What is the horizontal distance covered by the stone in the second example?

The horizontal distance covered by the stone is calculated to be 120 meters, using the initial velocity of 50 m/s, an angle of 37°, and the time taken to reach the highest point as 3 seconds.

What does the equation X = (v0^2 * sin(2 * Alfa)) / g represent?

This equation represents the formula to calculate the maximum horizontal distance traveled by a projectile in ideal conditions, where X is the horizontal distance, v0 is the initial velocity, Alfa is the launch angle, and g is the acceleration due to gravity.

How do we determine the initial velocity from a given maximum horizontal distance and launch angle?

To find the initial velocity, we use the formula v0 = sqrt((X * g) / (sin(2 * Alfa))), where X is the maximum horizontal distance, g is the acceleration due to gravity, and Alfa is the launch angle.

In the third example, what is the initial velocity of the projectile?

The initial velocity of the projectile is calculated to be 120 m/s, based on the given maximum horizontal distance (720 * √3) and the launch angle of 30°.

What is the significance of the sin and cos functions in projectile motion calculations?

The sine and cosine functions are used to resolve the velocity vector into horizontal and vertical components. Sin is used for the vertical component (to calculate time or height), and cos is used for the horizontal component (to calculate distance).

What role does gravity play in projectile motion?

Gravity influences the vertical motion of the projectile, causing it to decelerate on its way up, reach a peak, and then accelerate downward. It also affects the time it takes for the projectile to reach its highest point and return to the ground.