History of Calculus: Part 1 - Calculus in a Nutshell

Summary

This video script introduces the concept of calculus as a tool for analyzing curves and functions, which can represent various phenomena in the universe. It outlines the historical quest to 'square' curves and find tangents, leading to calculus's development. The script explains calculus's ability to determine properties like area, length, concavity, and extrema of curves. It simplifies calculus into a 'cutting and stitching' process, distinguishing between differential and integral calculus. The video also touches on the historical evolution of calculus, from its pioneers' intuitive use of infinitesimals to the 19th-century formalization using limits, promising a journey through the history of calculus starting from Ancient Egypt.

Takeaways

• 📚 Calculus was initially developed as a tool to analyze mathematical curves and find their properties.
• 🔍 Calculus helps to determine five key properties of curves: area under the curve, curve length, concavity, tangents, and points of maxima or minima.
• 🎓 The historical quest to 'square the curve' and find tangents were significant catalysts in the discovery of calculus.
• 🌐 Calculus evolved from analyzing curves to analyzing functions, which are more abstract mathematical entities.
• 🤔 Mathematicians were motivated to analyze curves due to their representation of measurable phenomena in the universe.
• 🌟 Calculus is considered one of the most important branches in mathematics because of its applicability to understanding various natural phenomena.
• ⚙️ The core processes of calculus are 'cutting' and 'stitching,' which involve dividing a curve into infinitesimally small pieces and then recombining them.
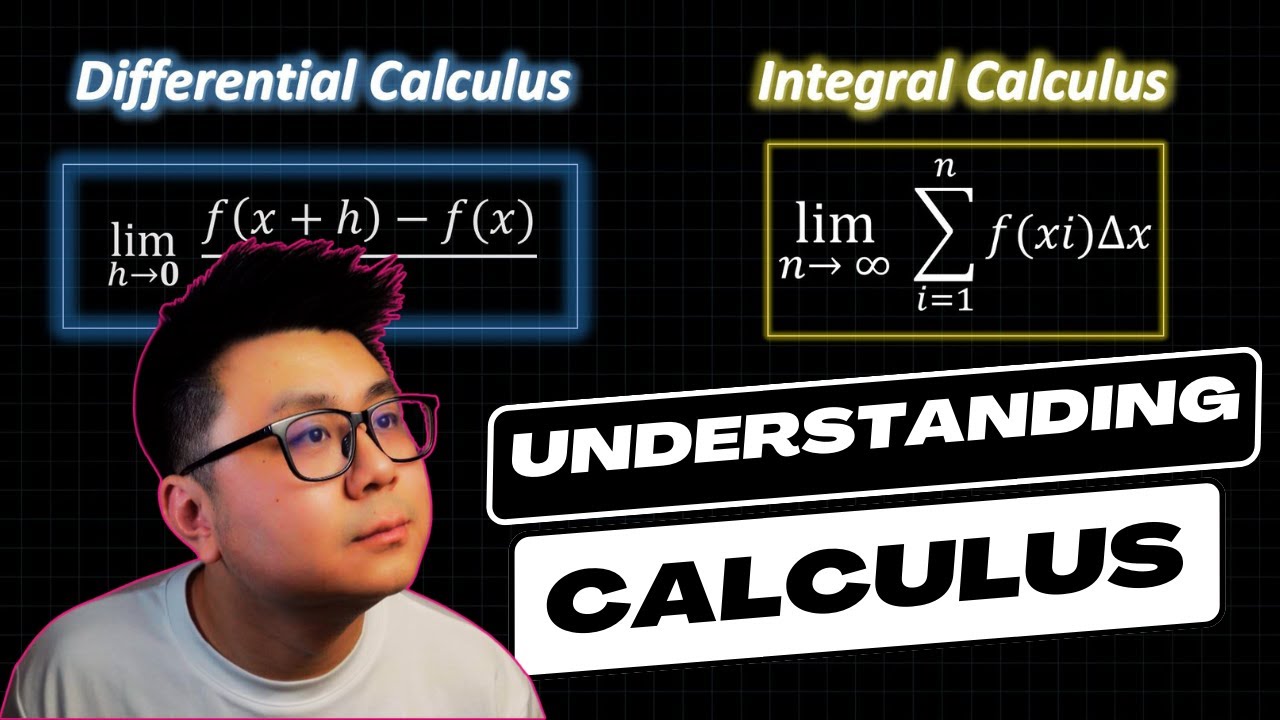
• 🧩 Differential calculus deals with the 'cutting' process, while integral calculus handles the 'stitching' back together.
• 🤓 The concept of infinitesimals, though logically vague, was crucial for the pioneers of calculus and has been replaced by limits in modern formulations for logical rigor.
• 🏛️ Calculus simplifies complex problems that once required extraordinary ingenuity, making it accessible to a broader range of individuals through systematic rules and symbols.

Q & A

What is the primary focus of the video series 'The History of Calculus'?

The primary focus of the video series 'The History of Calculus' is to explore how calculus evolved and to understand it in a more intuitive way by learning it through its historical development.

What are the five properties of curves that calculus helps us find?

The five properties of curves that calculus helps us find are: 1) the area under the curve, 2) the length of the curve, 3) the concavity of the curve, 4) the tangent to the curve, and 5) the points of maxima or minima.

Why were mathematicians historically interested in 'squaring the curve'?

Historically, mathematicians were interested in 'squaring the curve' as it was a way to find the area under a curve, which was a significant challenge and a major catalyst in the discovery of calculus.

How does calculus extend the concept of finding tangents to curves?

Calculus extends the concept of finding tangents to curves by using the process of cutting the curve into infinitely small pieces and extending the piece at a particular point to get the tangent line.

What is the practical motivation behind the development of calculus?

The practical motivation behind the development of calculus was the ability to analyze and find properties of different phenomena in the universe, which could be represented by curves.

What are the two simple processes that calculus uses to analyze curves?

The two simple processes that calculus uses to analyze curves are 'cutting' and 'stitching', which involve dividing the region or curve into infinitely small pieces and then combining them to find the desired properties.

What is the branch of calculus that deals with the 'cutting' process?

The branch of calculus that deals with the 'cutting' process is called differential calculus.

What is the branch of calculus that deals with the 'stitching' process?

The branch of calculus that deals with the 'stitching' process is called integral calculus.

How did the concept of infinitesimals contribute to the development of calculus?

The concept of infinitesimals, although vague and illogical, contributed to the development of calculus by allowing mathematicians to work with infinitely small pieces of curves or areas, which led to correct results despite the lack of a solid logical foundation at the time.

What was the significant change in the understanding of calculus in the 19th century?

In the 19th century, mathematicians tackled the problem of infinitesimals by eliminating them and introducing limits, which put calculus on a stronger logical foundation but made it more difficult to understand.

How does calculus simplify the process of analyzing shapes and curves compared to earlier methods?

Calculus simplifies the process of analyzing shapes and curves by providing a systematic method of cutting and stitching, which reduces problems that once required significant ingenuity and creativity into a set of rules and symbols that anyone can apply.

Outlines

00:00

📚 Introduction to Calculus

This paragraph introduces the concept of calculus and its historical development. Calculus began as a tool for analyzing mathematical curves, helping to determine properties such as area under the curve, curve length, concavity, tangent lines, and extrema (maxima and minima). The paragraph emphasizes the practical applications of calculus in understanding various phenomena in the universe, which can be represented by curves. The historical quest to 'square the curve' and find tangents were significant in the discovery of calculus. The video also touches on the evolution of calculus from analyzing curves to more abstract functions and the transition from using infinitesimals to limits for a more solid logical foundation.

05:01

🔍 How Calculus Works

This paragraph delves into the mechanics of how calculus operates, focusing on the processes of 'cutting' and 'stitching.' It explains that calculus breaks down a curve into infinitely small pieces to calculate properties like area and length, then combines these pieces to find the total. The paragraph distinguishes between differential calculus, which deals with the cutting process, and integral calculus, which involves stitching the pieces back together. It also addresses the historical and philosophical questions surrounding the concept of infinitesimals, which were eventually replaced by limits in the 19th century to provide a more rigorous foundation for calculus. The paragraph concludes by highlighting the power of calculus to simplify complex problems and its evolution from ancient methods to a systematic discipline.

Keywords

💡Calculus

Calculus is a branch of mathematics that studies how things change. In the video, calculus is described as originating as a tool to analyze and find properties of mathematical curves, such as areas, lengths, concavity, tangents, and extrema. It is central to the video's theme as it sets the stage for understanding the historical development and practical applications of calculus.

💡Curves

In the context of the video, curves refer to graphical representations of functions or equations. The script uses curves to illustrate the properties that calculus can help find, such as the area under a curve, the length of a curve, and the tangent at a point on a curve. Curves are a visual and intuitive way to introduce the concepts of calculus.

💡Area under the curve

The area under a curve is a fundamental concept in calculus, historically known as 'squaring the curve.' The video explains that calculus can be used to find this area, which is a measure of the space enclosed by the curve and the x-axis. This concept is crucial for understanding integral calculus, one of the two main branches of calculus.

💡Length of the curve

Calculus can also determine the length of a curve, a concept historically referred to as 'rectifying the curve.' The video uses this property to demonstrate how calculus extends beyond simple geometric shapes to more complex curves, emphasizing the versatility of calculus in analyzing shapes and forms.

💡Concavity

Concavity refers to the curvature of a curve, indicating whether it bends upwards or downwards. The video explains that calculus can determine the concavity, which is important for understanding the behavior of the curve and its potential applications in various fields.

💡Tangent

A tangent, in the historical context provided by the video, is a line that touches the curve at exactly one point. Calculus is used to find the equation of the tangent line, which is essential for analyzing local properties of the curve and has wide applications in physics and engineering.

💡Maxima and Minima

Maxima and minima are points on a curve where it reaches its highest or lowest point, respectively. The video discusses how calculus can identify these points, which are critical in optimization problems and understanding the behavior of various phenomena modeled by curves.

💡Differential Calculus

Differential calculus is one of the two main branches of calculus, focusing on the 'cutting' process. As explained in the video, it deals with the rates of change and the slopes of curves, which is essential for finding tangents and understanding how quantities change.

💡Integral Calculus

Integral calculus is the other main branch of calculus, focusing on the 'stitching' process. The video describes how it is used to find the total area under a curve by integrating the areas of infinitely small pieces, which is fundamental for calculating quantities like distances and volumes.

💡Infinitesimals

Infinitesimals are infinitely small quantities that were used by the pioneers of calculus to perform calculations before the concept of limits was formalized. The video acknowledges the historical use of infinitesimals and their role in the development of calculus, despite their logical ambiguities.

💡Limits

Limits are a fundamental concept in calculus that provides a rigorous foundation for the manipulation of infinitesimals. The video mentions that limits were introduced in the 19th century to resolve the logical issues with infinitesimals, making calculus more rigorous but also more complex.

Highlights

Learning calculus through its historical evolution is more intuitive than the current teaching methods.

Calculus was originally developed as a tool to analyze mathematical curves.

One of the first properties calculus helps find is the area under a curve, historically known as squaring the curve.

Calculus can also determine the length of a curve, known as rectifying the curve.

Concavity of a curve, indicating whether it bends upwards or downwards, is another property calculus can reveal.

Calculus is used to find the tangent to a curve, defined as the line touching the curve at a single point.

Maxima and minima points of a curve can be identified using calculus.

Calculus evolved from analyzing curves to more abstract mathematical objects like functions.

Mathematicians were motivated to analyze curves because many natural phenomena can be represented by them.

Calculus is considered one of the most important branches in mathematics due to its applicability to real-world phenomena.

Calculus operates on two processes: cutting and stitching, used to analyze and understand properties of curves.

Differential calculus deals with the cutting process, while integral calculus focuses on stitching pieces back together.

The concept of infinitesimals was a key part of early calculus but was later replaced by limits for a more logical foundation.

The historical approach to calculus, using cutting and stitching, predates calculus itself and was used by ancient mathematicians.

Calculus provides a systematic method for what once required individual ingenuity, such as Archimedes' work on the sphere.

Today, calculus allows students to solve problems that once only the greatest mathematicians could tackle.

Calculus is a powerful tool for analyzing curves and functions, and by extension, various phenomena in the universe.

Transcripts

Learning calculus the way it evolved is generally

a lot easier and much more intuitive than the way it is currently taught.

And this will be the topic of this series:

The History of Calculus.

But first we want to develop a general  understanding of calculus, that is:

What is calculus?

Why do we need it?

And how does it work?

And this will be the topic of this video:

Calculus in a Nutshell.

So let's start with the first question:

What is calculus?

Calculus originated as a tool to analyze and find properties

of different mathematical curves.

Let's take this curve as an example:

Calculus helps us find many of its properties

and I'll talk about five of those:

The first property that calculus helps  us find

is the area under the curve.

Historically this was known as squaring the curve

or finding a square with an equivalent area.

It was mathematicians' quest to square different curves

that led to the discovery of calculus.

The second property that calculus helps  us find

is the length of the curve.

This was known historically  as rectifying the curve or  

finding a straight line with an equivalent length.

The third property that calculus  helps us find

is the concavity of the curve.

Is it bent downwards?

Is it bent upwards?

Is it just a straight line?

And if you're wondering, isn't it obvious to which way the curve is bent!

Remember that these curves are usually given in an equation format

and their concavities are not immediately obvious.

The fourth property that calculus helps us find

is the tangent to the curve.

Historically the tangent was defined as

the line that touches the curve at one point only.

This definition has been modified since then,

but to keep things simple we will stick with it for the time being.

Together with squaring curves,

finding tangents was a major catalyst in the discovery of calculus.

And the fifth property that calculus helps us find

is the point of maxima or the highest point of the curve,

and if the curve is bent downwards then the point of minima

or the lowest point of the curve.

I like to think of calculus as a machine  with a dial.

We feed in the curve we want to study,

set the dial to the property we  want to get,

and it gives us the answer.

Over the years calculus evolved from analyzing curves into analyzing functions

which are more generic and abstract mathematical objects.

But since curves are more visual, and since they were

the objects that were being studied when calculus was discovered,

we will focus on them for now.

The second question is why?

Why were mathematicians interested in analyzing curves to start off with?

Now in general mathematicians are interested in mathematics

for intellectual purposes.

They are driven by a sense of

intellectual challenge,

intellectual beauty,

regardless whether or not their work has any practical applications.

But in the case of calculus there was also a major practical motivation.

See, it was discovered that many, if not all, measurable phenomena in the universe

could be represented by curves.

So for example the speed of a pendulum

can be represented by this curve.

The distance covered by a falling object

can be represented by this curve.

The growth of bacteria in an enclosed environment

can be represented by this curve.

And the list goes on!

So when we have a tool like calculus that can

analyze and find properties of different curves,

we have a tool that can analyze and find  properties

of different phenomena in the universe.

And this was a major motivation for analyzing curves

and subsequently discovering calculus,

and the major reason why  calculus today is considered  

one of the most important branches in mathematics.

The third question is how?

How does calculus work?

What's happening under the hood of our machine?

Calculus uses two simple  processes:

Cutting and stitching.

For example when we want to find the  area of the region under the curve,  

calculus cuts the region into infinitely small pieces,

finds the area, or more accurately, the equation of the area, of these pieces

and then stitches them back together to get the total area.

Similarly, when we want to  find the length of the curve,  

calculus cuts the curve into infinitely  small pieces,

finds the equation of the length of these pieces

and then stitches them back together to get the total length.

For some properties we only need the  cutting process without the stitching.

For example when we want to find the  tangent to the curve at a particular point,  

we use calculus to cut the  curve into infinitely small pieces 

and then extend the piece  at that point to get the tangent.

And when we want to find the point of maxima

we use calculus to cut the curve into infinitely small pieces

and then look for the piece which is  horizontal

and that would be the point of maxima.

And if the curve is bent downwards  then that would be the point of minima.

Calculus’ branch that deals with  cutting is called differential calculus.

And its branch that deals with stitching is called integral calculus.

Where we integrate the pieces back together to form a whole.

But now we want to address a  vagueness in our terminology:  

What do we mean by an infinitely small  piece?

Or what is known as the infinitesimal!

Is it bigger than zero?

If so can't we just cut it in half and get a smaller piece?

And then cut that piece in half and get even a smaller one

and keep doing so indefinitely!

Is it equal to zero?

If so, isn’t stitching zeros together results in nothing but zero?!

Pioneers of calculus in the 17th and 18th century

were aware of this vague and illogical nature of infinitesimals

but they couldn't resist using them as they gave correct results.

It wasn't until the 19th century

that mathematicians tackled the problem of infinitesimals

and to do so they eliminated infinitesimals altogether

and they introduced limits instead.

And while they succeeded in putting  calculus on a stronger logical foundation,  

they also made it much more  difficult to understand.  

And this ended up being the curriculums  that we currently study around the world.

But it is a lot easier to follow  the natural evolution of calculus.  

So for the time being

we're going to adopt the attitude of the pioneers of calculus

and pretend that we can indeed cut something into infinitely small pieces

and then stitch them back  together to get different results.

This method of solving problems by cutting and stitching

predates calculus by thousands of years.

Ancient mathematicians used it to

find areas and volumes of different shapes.

But the main difference between  calculus and its predecessors  

is that calculus makes this process  of cutting and stitching

systematic.

See, with earlier methods

different shapes needed to be cut and stitched back differently.

And this usually required  a lot of ingenuity and creativity.

For example Archimedes, one of the  best mathematicians of all time,  

used this cutting and stitching method to find the volume of the sphere.

He worked out that the volume of the sphere

is two-thirds the volume of the cylinder that surrounds it.

He was so proud of this discovery

that he asked for it to be engraved on his tombstone.

Today with calculus, a school  student can find areas,  

volumes and many more properties of  shapes and curves that Archimedes  

didn't even know existed let alone  be able to find their properties!

And such is the power of calculus:

It reduces problems that once required an Archimedes

into a set of rules and symbols that anyone can apply.

And this is calculus in a nutshell:

It's a systematic way

of cutting and stitching

to analyze different curves and functions

and in turn analyze different phenomena in the universe.

So let's go back to where it  all started:

Ancient Egypt.

See you in the next video.

Coming Soon