Intervalo de Confiança para a Média Populacional (μ) com variância populacional conhecida
Summary
TLDRThis video provides a comprehensive guide on calculating confidence intervals for the population mean when the population variance is known. It explains the concept of confidence intervals, the necessary assumptions, and the formula used to calculate them. Through detailed examples, the video demonstrates how to compute the interval for various scenarios, such as estimating cholesterol levels and salaries. Viewers are guided through step-by-step calculations and are shown how different factors, like sample size and confidence level, affect the margin of error and the resulting interval. It’s an essential resource for anyone learning statistical estimation techniques.
Takeaways
- 😀 Confidence intervals (CIs) are used to estimate the population mean based on sample data.
- 😀 The sample mean is a point estimate, but we must account for sampling error by adding a margin of error.
- 😀 The margin of error depends on the sample size (n), the population standard deviation (σ), and the confidence level.
- 😀 A confidence interval provides a range of values within which the true population mean (μ) is likely to fall.
- 😀 To calculate a confidence interval, we need to use the formula: μ = [x̄ - Z * (σ/√n), x̄ + Z * (σ/√n)].
- 😀 The Z-value for the confidence interval depends on the desired confidence level (e.g., Z=1.96 for 95% confidence).
- 😀 The sample should be randomly selected, and the population must either be normally distributed or large enough for the Central Limit Theorem to apply.
- 😀 As the sample size (n) increases, the margin of error decreases, leading to a more precise estimate of the population mean.
- 😀 Larger population standard deviations (σ) increase the margin of error and make the interval wider.
- 😀 A higher confidence level (e.g., 99%) increases the margin of error because it requires a larger Z-value.
- 😀 In practical examples, you can apply these principles to specific problems, such as estimating cholesterol levels or employee salaries based on sample data.
Q & A
What is the purpose of calculating a confidence interval for a population mean?
-The purpose of calculating a confidence interval for a population mean is to estimate the true population mean based on a sample, while considering the margin of error. This interval provides a range of values that the population mean is likely to fall within, offering a more accurate estimate than a point estimate alone.
What assumptions are made when calculating a confidence interval for the population mean?
-The key assumptions when calculating a confidence interval for the population mean include: (1) the sample is randomly selected, and (2) the population follows a normal distribution, although the Central Limit Theorem allows this to be relaxed for large sample sizes.
Why can’t we simply use the sample mean as the population mean?
-The sample mean is just an estimate of the population mean and can be affected by sampling error. It is not necessarily equal to the true population mean. Therefore, we need to account for the margin of error and use a confidence interval to estimate the population mean more accurately.
What is the relationship between the sample size and the margin of error in a confidence interval?
-The margin of error decreases as the sample size increases. This is because a larger sample provides more reliable information about the population, reducing variability in the estimate of the population mean.
How does the population standard deviation affect the margin of error?
-The margin of error increases as the population standard deviation increases. A higher standard deviation means more variability in the data, which leads to a larger margin of error and a wider confidence interval.
What does the 'z' value represent in the formula for a confidence interval?
-The 'z' value corresponds to the critical value for a standard normal distribution at a given confidence level. It is used to determine the range within which the population mean is likely to fall, based on the desired confidence level.
How do you calculate the confidence interval for a population mean when the population variance is known?
-When the population variance is known, the confidence interval is calculated using the formula: x̄ ± (z * σ / √n), where x̄ is the sample mean, z is the z-value corresponding to the desired confidence level, σ is the population standard deviation, and n is the sample size.
What does the expression 1 - α represent in the context of confidence intervals?
-The expression 1 - α represents the confidence level. For example, if α = 0.05, then 1 - α = 0.95, which corresponds to a 95% confidence level. This indicates that there is a 95% chance that the true population mean lies within the calculated confidence interval.
How does increasing the confidence level affect the confidence interval?
-Increasing the confidence level (e.g., from 90% to 95%) results in a wider confidence interval. This is because a higher confidence level requires a larger margin of error to ensure that the true population mean is within the interval with greater certainty.
What does a 95% confidence level mean in terms of probability?
-A 95% confidence level means that if the same sampling procedure were repeated multiple times, approximately 95% of the confidence intervals generated would contain the true population mean. It reflects the likelihood that the interval contains the true value.
Outlines

هذا القسم متوفر فقط للمشتركين. يرجى الترقية للوصول إلى هذه الميزة.
قم بالترقية الآنMindmap

هذا القسم متوفر فقط للمشتركين. يرجى الترقية للوصول إلى هذه الميزة.
قم بالترقية الآنKeywords

هذا القسم متوفر فقط للمشتركين. يرجى الترقية للوصول إلى هذه الميزة.
قم بالترقية الآنHighlights

هذا القسم متوفر فقط للمشتركين. يرجى الترقية للوصول إلى هذه الميزة.
قم بالترقية الآنTranscripts

هذا القسم متوفر فقط للمشتركين. يرجى الترقية للوصول إلى هذه الميزة.
قم بالترقية الآنتصفح المزيد من مقاطع الفيديو ذات الصلة

Confidence Interval [Simply explained]

Intervalo de Confiança para a MÉDIA (μ) com variância populacional desconhecida
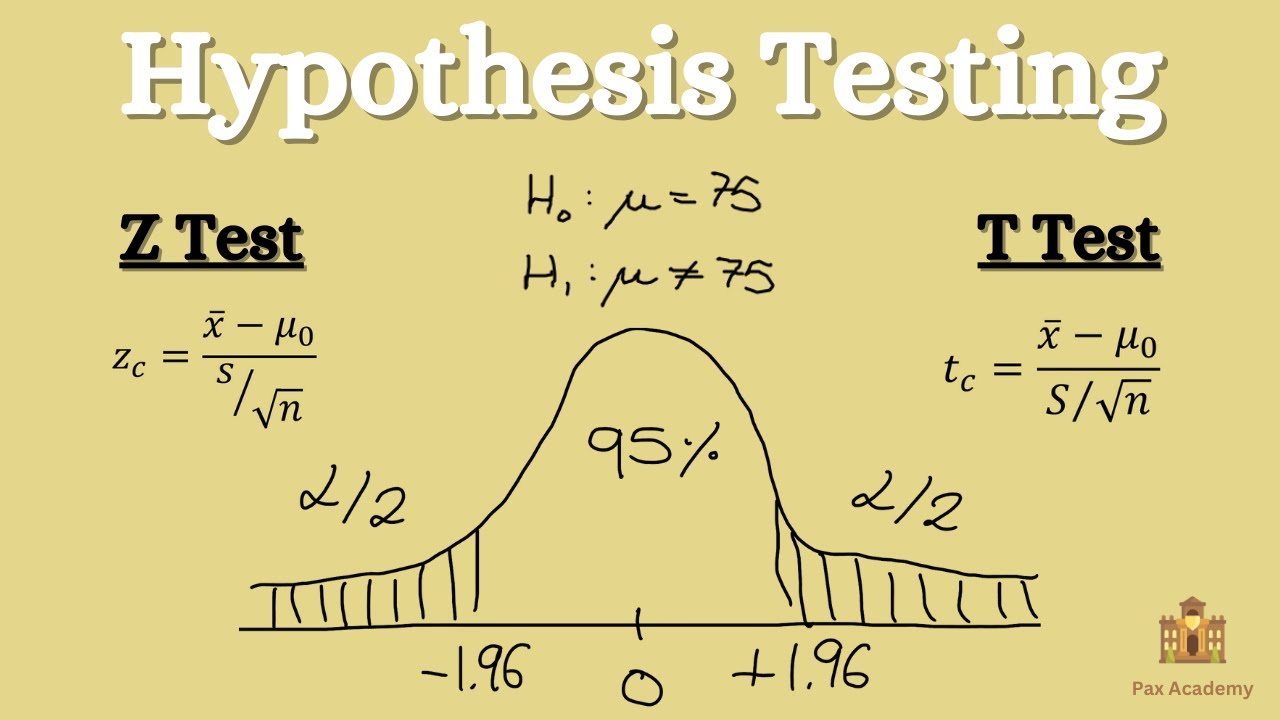
Hypothesis Testing - Z test & T test

Hypothesis Test vs. Confidence Interval | Statistics Tutorial #15 | MarinStatsLectures
Descriptive Statistics vs Inferential Statistics | Measure of Central Tendency | Types of Statistics

05 One-Sample t-Tests in SPSS – SPSS for Beginners
5.0 / 5 (0 votes)
