Lecture 3 Part 1 - Crystal Structure - 2 (Unit Cell, Lattice, Crystal)
Summary
TLDRThis lecture delves into the concept of unit cells in crystallography, explaining how they can fill space without voids. It clarifies the difference between a lattice—a periodic arrangement of points—and a crystal, which includes atoms or molecules at these points. The talk covers the parameters needed to define unit cells in 1D, 2D, and 3D, highlighting the importance of choosing the smallest unit cell with the highest symmetry. The discussion also touches on primitive and non-primitive unit cells, emphasizing that the term 'unit cell' refers to the basic repeating unit, not necessarily a unit of size one.
Takeaways
- 📐 The concept of a unit cell is fundamental in understanding lattice structures, where an infinite number of possible unit cells can fill the entire space.
- 🔍 Unit cells can have various geometries, but the most common in 2D are parallelograms, while in 3D, parallelepipeds are typical.
- 📏 In 2D, a unit cell is defined by two vectors, 'a' and 'b', and the angle 'alpha' between them, which can lead to different shapes like squares or rectangles depending on their values.
- 🚫 A triangular unit cell cannot fill space by translation alone without leaving voids, and thus is not a qualified unit cell for space filling.
- 🧊 In 1D, the unit cell is simply a line, which can be repeated to construct the entire lattice.
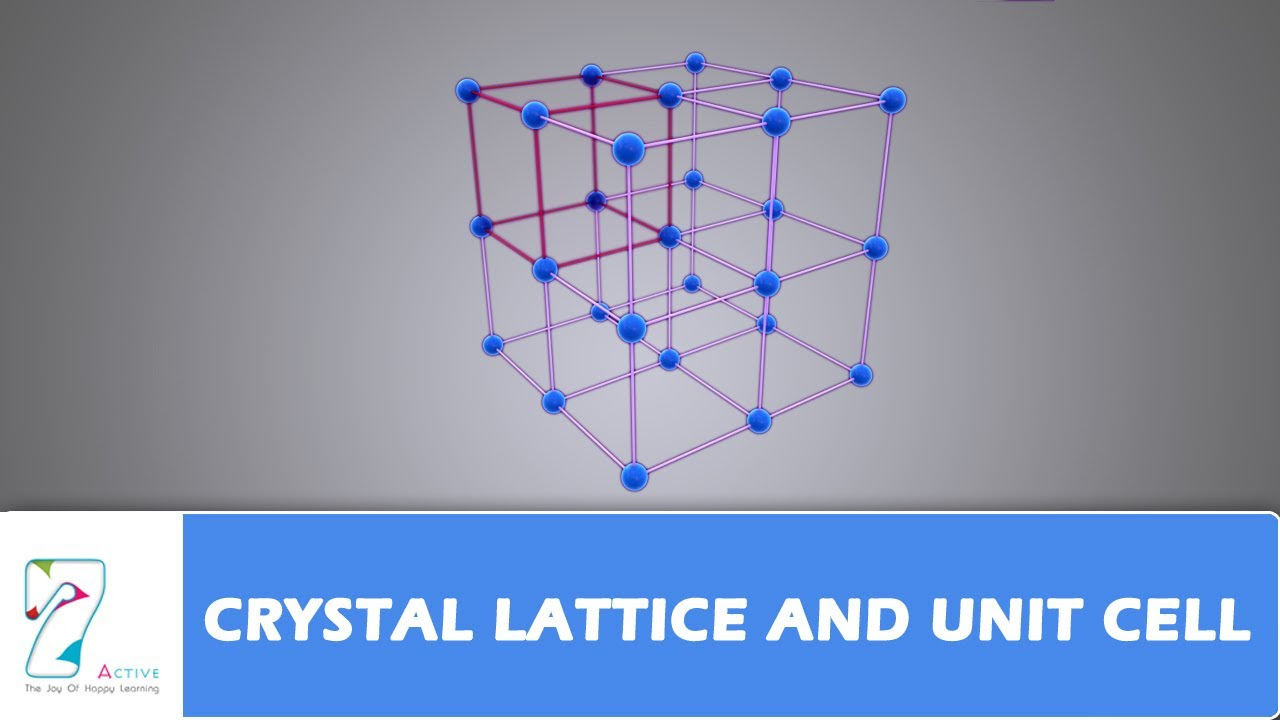
- 💎 The difference between a lattice and a crystal is that a lattice is an arrangement of points, while a crystal is a lattice with atoms, ions, or molecules occupying these points.
- 🔑 The term 'primitive unit cell' refers to the smallest unit cell with the highest symmetry, typically containing one lattice point or one motif.
- 🔄 While the guideline is to choose the smallest possible unit cell, it's possible to choose any number of unit cells to fill the space.
- 📈 The parameters required to define a unit cell in 3D are a, b, c, alpha, beta, and gamma, which are six lattice parameters.
- 🔢 The size of the unit cell is not necessarily 'unit' in measurement; it refers to the basic repeating unit in the structure.
- 💠 The concept of a crystal is essentially a lattice plus a motif or basis, which defines what sits on the lattice points and how to repeat them to construct the crystal structure.
Q & A
What is the concept of a unit cell in the context of a lattice?
-A unit cell is the smallest repeating unit in a lattice that can be used to fill the entire space without leaving any gaps. It is defined by its geometry and can be translated along the lattice vectors to construct the lattice.
Can a unit cell be any shape, such as a triangle or a parallelogram?
-In 2D, a unit cell can be a parallelogram. While a triangle might seem like a candidate, it cannot fill the entire space by translation alone without leaving voids, hence it is not a qualified unit cell for filling space.
What are the parameters required to describe a unit cell in 2D?
-In 2D, the parameters required to describe a unit cell are two vectors, one in the x direction (denoted as 'a') and another in the y direction (denoted as 'b'), along with the angle between them (alpha).
What is the significance of the angle alpha in a 2D unit cell?
-The angle alpha is the included angle between the two vectors a and b. It, along with the lengths of a and b, determines the shape and orientation of the unit cell.
What is the difference between a primitive and a non-primitive unit cell?
-A primitive unit cell has one lattice point per unit cell, effectively containing one atom, molecule, or ion if it's a crystal. A non-primitive unit cell has more than one lattice point per unit cell, meaning it contains multiple atoms, molecules, or ions.
Why is a parallelogram considered a qualified unit cell for a 2D lattice?
-A parallelogram is considered a qualified unit cell for a 2D lattice because it can be translated along the x and y directions without leaving any voids, thus filling the entire space.
What is the simplest form of a unit cell in 1D?
-In 1D, the simplest form of a unit cell is a line segment, which represents the basic unit being repeated along the lattice.
What is the difference between a lattice and a crystal?
-A lattice is a translationally periodic arrangement of points in space, while a crystal is a lattice where these points are occupied by atoms, ions, or molecules, forming a solid material with a periodic structure.
How many lattice parameters are required to define a unit cell in 3D?
-In 3D, six lattice parameters are required to define a unit cell: three edge lengths (a, b, c) and three angles (alpha, beta, gamma).
What does the term 'motif' or 'basis' refer to in the context of a crystal?
-In the context of a crystal, 'motif' or 'basis' refers to the arrangement of atoms, ions, or molecules that occupy the lattice points, defining the crystal's structure.
Why is the size of a unit cell not necessarily related to the number '1'?
-The size of a unit cell is not necessarily related to the number '1' because the term 'unit cell' refers to the basic unit that is repeated in the lattice to construct the entire structure, rather than its size being a unit length.
Outlines

此内容仅限付费用户访问。 请升级后访问。
立即升级Mindmap

此内容仅限付费用户访问。 请升级后访问。
立即升级Keywords

此内容仅限付费用户访问。 请升级后访问。
立即升级Highlights

此内容仅限付费用户访问。 请升级后访问。
立即升级Transcripts

此内容仅限付费用户访问。 请升级后访问。
立即升级浏览更多相关视频

noc19-cy16 Lecture 06-Solid state Chemistry-Week 2 Lecture-1 Unit Cell
CRYSTAL LATTICE AND UNIT CELL

Aula 10 – Estruturas Cristalinas Cúbicas de Face Centrada, Corpo Centrado e Hexagonal Compacta.

ICT Mentorship Core Content - Month 04 - Liquidity Voids

2A Conceitos básicos de simetria e cristalografia

Constructing an Ewald Sphere
5.0 / 5 (0 votes)
