Statistika NonParametrik : Uji Runtun (Run Test) - PART 1
Summary
TLDRThis video provides an introduction to nonparametric statistics, focusing on the runs test to analyze randomness in a sample. The runs test evaluates whether a sequence of data points (such as gender in a sample) follows a random pattern or displays a structured trend. The process involves calculating the number of runs and comparing it to expected values to determine randomness. Critical values, significance levels, and probability formulas are discussed to interpret results and make decisions about the randomness of a sample. The video highlights the importance of nonparametric methods in statistical analysis when distribution assumptions cannot be made.
Takeaways
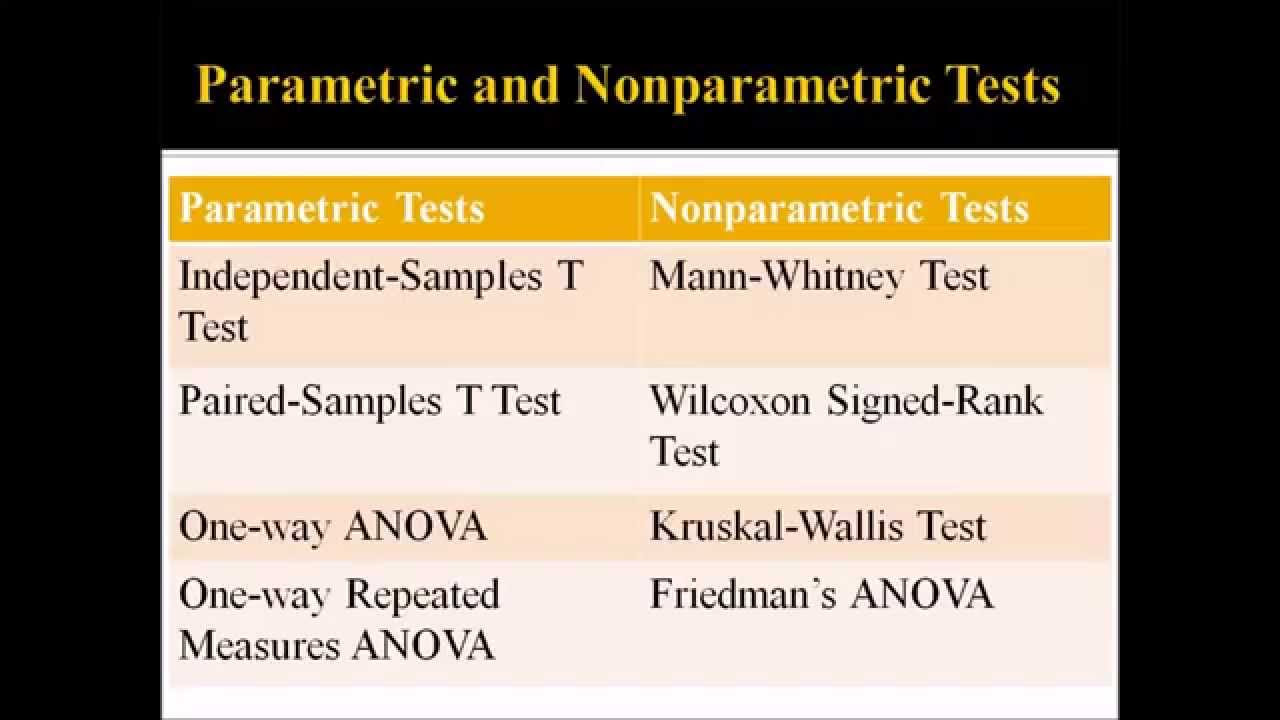
- 😀 Non-parametric statistics do not rely on specific distribution assumptions, making them versatile for various data types.
- 😀 The run test is a non-parametric method used to examine the randomness of a sample by identifying consecutive similar values.
- 😀 In a sample, a 'run' refers to a sequence of consecutive identical values, like consecutive males or females in a gender-based sample.
- 😀 The primary goal of the run test is to determine if the observed sample follows a random pattern or if there is a structured non-random order.
- 😀 A sample is considered random if the runs are distributed within certain critical bounds; otherwise, the hypothesis of randomness is rejected.
- 😀 Critical values for the run test (RB and RA) are calculated using formulas or tables based on the significance level (usually α = 5%).
- 😀 The process for hypothesis testing in the run test includes calculating the number of runs, then comparing it to the critical values derived from the alpha level.
- 😀 The significance level (alpha) is crucial in determining whether the observed runs fall within the acceptable range for randomness.
- 😀 If the observed number of runs falls between the critical values, the sample is considered random; if it falls outside, the sample is non-random.
- 😀 The lecture emphasized that, depending on the sample size and number of categories (e.g., males and females), the smallest group should be used to calculate critical values.
- 😀 In practice, using a table of critical values for the run test can simplify the process and eliminate the need for complex formula-based calculations.
Q & A
What is nonparametric statistics?
-Nonparametric statistics refers to methods that do not assume a specific distribution for the sample data. Unlike parametric tests, which assume normal distribution or other specific forms, nonparametric tests are used when the data doesn't meet those assumptions.
What is the primary focus of the run test (Uji Runtun)?
-The run test is primarily used to determine whether a sequence of observations (such as gender, color, etc.) is randomly distributed. It helps to identify if the sample is random or if there is a pattern suggesting non-randomness.
What is a 'run' in the context of the run test?
-A 'run' is a sequence of consecutive identical values within the dataset. For example, if a sample contains 6 men and 6 women, a run might occur when two consecutive individuals in the sample are both men or both women.
How does the run test determine if a sample is random?
-The run test calculates the number of runs (consecutive identical values) in the sample and compares it with critical values. If the number of runs is too small or too large, the sample may be considered non-random. If the number of runs falls between the critical values, the sample is considered random.
What are critical values in the run test?
-Critical values in the run test are predefined values that determine whether the sample's number of runs indicates randomness or non-randomness. These values are based on the sample size and the significance level (α), typically set at 5%.
How are critical values calculated in the run test?
-Critical values can be calculated using a statistical formula based on the number of observations in each category (e.g., men and women in a gender-based sample). Alternatively, critical values can be looked up in a table of run values for a given significance level (α).
What happens if the observed number of runs is outside the critical values?
-If the observed number of runs falls outside the critical values, the null hypothesis (that the sample is random) is rejected, indicating that the sample may not be random and suggesting a non-random sequence.
What does it mean when the observed number of runs falls between the critical values?
-When the observed number of runs falls between the critical values, the sample is considered random, meaning there is no evidence to suggest a non-random pattern in the sequence of observations.
What is the significance level (α) used in the run test, and how does it affect the critical values?
-The significance level (α) is commonly set at 5%, which means that the test is looking for patterns in the data that occur less than 5% of the time by chance. The significance level affects the critical values, determining the cutoffs for whether the number of runs is considered too extreme to be random.
What is the role of N1 and N2 in the run test?
-N1 and N2 represent the number of observations in each category (for example, N1 could be the number of men and N2 could be the number of women in a gender-based sample). These values are important for calculating the critical values of the run test and determining the significance of the observed runs.
Outlines

此内容仅限付费用户访问。 请升级后访问。
立即升级Mindmap

此内容仅限付费用户访问。 请升级后访问。
立即升级Keywords

此内容仅限付费用户访问。 请升级后访问。
立即升级Highlights

此内容仅限付费用户访问。 请升级后访问。
立即升级Transcripts

此内容仅限付费用户访问。 请升级后访问。
立即升级浏览更多相关视频
5.0 / 5 (0 votes)