KALKULUS | TURUNAN | Aturan Rantai (Chain Rules)
Summary
TLDRIn this video, the chain rule in calculus is explained, focusing on differentiating composite functions. The script covers various types of composite functions, such as those with powers, trigonometric functions, and higher-order compositions. Examples demonstrate how to apply the chain rule for different cases, including differentiating functions like sin(3x), cos(2x), and more. The video also highlights the importance of taking derivatives of inner functions when using the chain rule, offering clear steps to simplify complex expressions. It aims to equip viewers with the skills to apply the chain rule confidently in various calculus problems.
Takeaways
- 😀 The chain rule is used to differentiate compositions of functions.
- 😀 A composition of functions, such as f(x) = sin(3x), involves applying one function to the result of another.
- 😀 Differentiating a composition is more complex than directly differentiating simple trigonometric functions.
- 😀 The chain rule involves taking the derivative of the outer function and multiplying by the derivative of the inner function.
- 😀 The first type of chain rule involves functions like y = (u(x))^n, where n is a constant exponent. The derivative involves multiplying by n and reducing the exponent by 1.
- 😀 Example 1: For y = (2x - 3)^10, the derivative is found using the chain rule, with a multiplication factor and the derivative of the inner function.
- 😀 Example 2: For y = (2x^3 - 2x^2 + 1)^50, the chain rule is again used, yielding a derivative with a large multiplication factor and the derivative of the inner function.
- 😀 In trigonometric functions, such as sin(x) or cos(x), the chain rule applies when the argument involves another function of x.
- 😀 Example 3: For y = sin(3x), the derivative is cos(3x) multiplied by 3, the derivative of the inner function 3x.
- 😀 Example 4: For y = cos(2x), the derivative is -sin(2x) multiplied by 2, the derivative of the inner function 2x.
- 😀 For higher powers or complex compositions of trigonometric functions, the chain rule can still be applied to obtain correct derivatives.
Q & A
What is the chain rule used for in calculus?
-The chain rule is used to differentiate composite functions, which are functions formed by combining two or more functions. It helps in calculating the derivative of functions where one function is nested inside another.
How do we differentiate a composite function like f(x) = sin(3x)?
-To differentiate f(x) = sin(3x), we apply the chain rule. The outer function is sin(x), and the inner function is 3x. The derivative of sin(x) is cos(x), and the derivative of 3x is 3. So, the derivative is 3 * cos(3x).
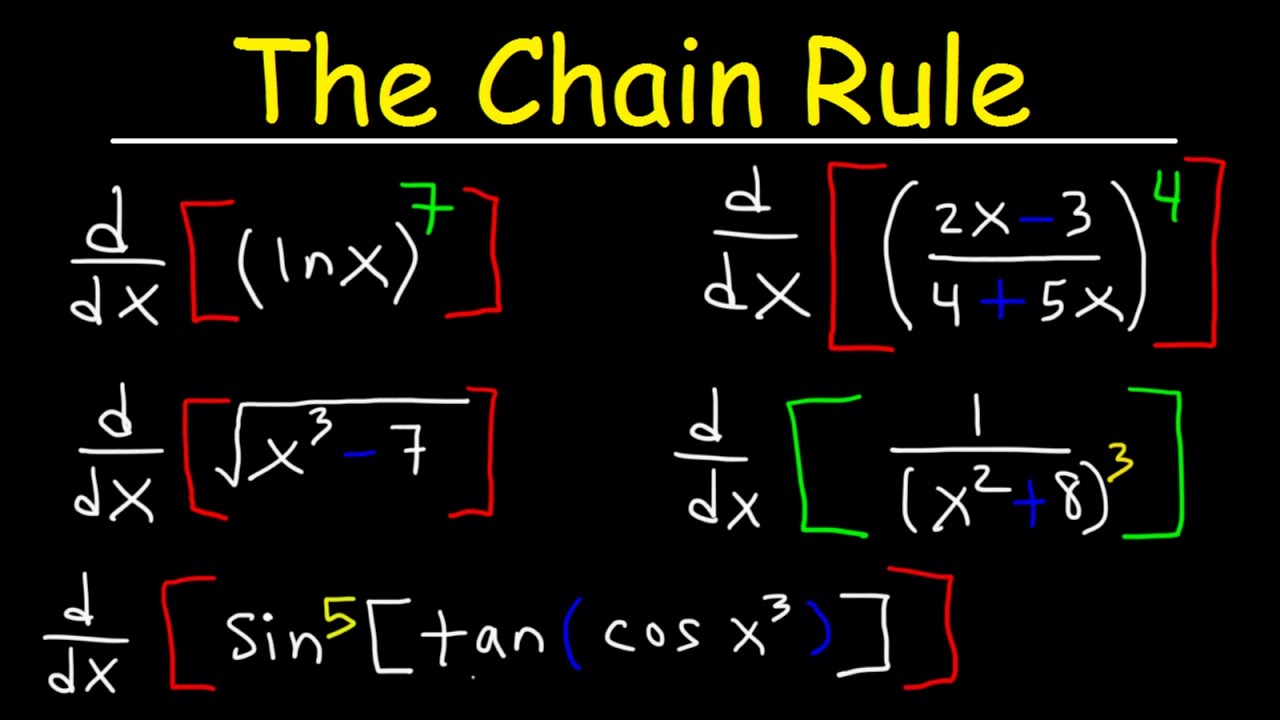
What is the process for differentiating a function of the form y = u(x)^n?
-For a function y = u(x)^n, where u(x) is a function of x and n is a constant, the derivative is given by the chain rule: y' = n * u(x)^(n-1) * u'(x), where u'(x) is the derivative of u(x) with respect to x.
Can you explain the differentiation of y = (2x - 3)^10?
-For y = (2x - 3)^10, the derivative is calculated using the chain rule. The outer function is (u(x))^10, and the inner function is 2x - 3. First, differentiate the outer function, which gives 10 * (2x - 3)^9, then multiply by the derivative of the inner function, which is 2. So, the result is 20 * (2x - 3)^9.
What is the derivative of a trigonometric function like sin(3x)?
-For the function y = sin(3x), the derivative is found using the chain rule. The derivative of sin(x) is cos(x), and the derivative of 3x is 3. Thus, the derivative is 3 * cos(3x).
How do you differentiate y = cos(2x)?
-For y = cos(2x), the derivative is the negative sine of 2x, as the derivative of cos(x) is -sin(x). Then, multiply by the derivative of the inner function, which is 2. So, the result is -2 * sin(2x).
What is the derivative of y = tan(3x^2)?
-For y = tan(3x^2), the derivative is calculated using the chain rule. The derivative of tan(x) is sec^2(x), so the derivative of tan(3x^2) is sec^2(3x^2) times the derivative of 3x^2, which is 6x. Therefore, the derivative is 6x * sec^2(3x^2).
How do you differentiate y = sin(cos(x^2))?
-For y = sin(cos(x^2)), the derivative is computed using the chain rule. First, the derivative of sin(x) is cos(x), so we differentiate the outer function and get cos(cos(x^2)). Then, we differentiate the inner function cos(x^2), which gives -sin(x^2) * 2x. Thus, the derivative is -2x * sin(x^2) * cos(cos(x^2)).
How do we differentiate a function like y = sin(x^3 + 5)?
-For y = sin(x^3 + 5), we apply the chain rule. The derivative of sin(x) is cos(x), so we differentiate the outer function to get cos(x^3 + 5). Then, we differentiate the inner function x^3 + 5, which gives 3x^2. Therefore, the derivative is 3x^2 * cos(x^3 + 5).
What does it mean to apply the chain rule for trigonometric functions with powers, like y = sin^3(4x)?
-When differentiating a function like y = sin^3(4x), we apply the chain rule in two stages. First, differentiate the outer function, which is sin^3(u), resulting in 3 * sin^2(u). Then, differentiate the inner function, sin(4x), using the chain rule again to get 4 * cos(4x). The result is 12 * cos(4x) * sin^2(4x).
Outlines

此内容仅限付费用户访问。 请升级后访问。
立即升级Mindmap

此内容仅限付费用户访问。 请升级后访问。
立即升级Keywords

此内容仅限付费用户访问。 请升级后访问。
立即升级Highlights

此内容仅限付费用户访问。 请升级后访问。
立即升级Transcripts

此内容仅限付费用户访问。 请升级后访问。
立即升级5.0 / 5 (0 votes)