Perbandingan Trigonometri
Summary
TLDRThis educational video covers trigonometric ratios and their application in the Cartesian coordinate system, focusing on the relationships of sine, cosine, tangent, and their reciprocals in all four quadrants. The script explains the definitions and signs of these functions in Quadrants I-IV, illustrating how to compute values for various angles (including special angles) using identities. Key concepts such as angle sum and difference formulas, as well as the behavior of trigonometric functions in different quadrants, are explored in detail. The video provides useful examples and tips for solving trigonometric problems involving angles beyond 90°.
Takeaways
- 😀 The lesson covers trigonometric comparisons in the context of a Cartesian plane, focusing on understanding the relationship between the X and Y axes in different quadrants.
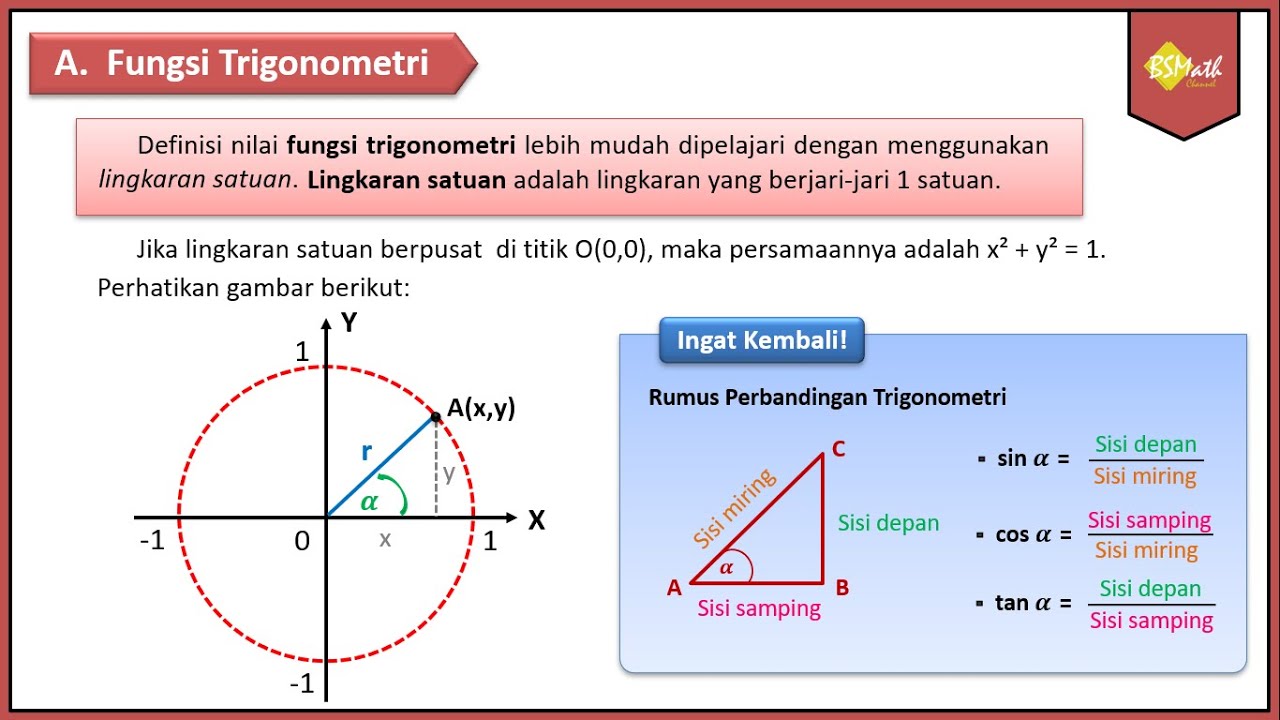
- 😀 In the first quadrant, points are represented by positive coordinates (x, y), with the Pythagorean theorem used to relate x, y, and the radius (r) as x² + y² = r².
- 😀 The reflection of the point in different quadrants follows a pattern: Quadrant I has coordinates (x, y), Quadrant II has (-x, y), Quadrant III has (-x, -y), and Quadrant IV has (x, -y).
- 😀 In trigonometry, the relationships between the sides of a triangle and the angle (alpha) are described: the opposite side (front), adjacent side (side), and hypotenuse (r).
- 😀 Basic trigonometric ratios for the first quadrant include: Sin(Alpha) = opposite/hypotenuse, Cos(Alpha) = adjacent/hypotenuse, and Tan(Alpha) = opposite/adjacent.
- 😀 Reciprocal trigonometric functions are introduced: Cosecant (CSC) = 1/Sin(Alpha), Secant (Sec) = 1/Cos(Alpha), and Cotangent (Cot) = 1/Tan(Alpha).
- 😀 In Quadrant II, trigonometric functions like Sin(Alpha) remain positive, while Cos(Alpha) and Tan(Alpha) become negative due to the position of the point on the unit circle.
- 😀 In Quadrant III, both Sin(Alpha) and Cos(Alpha) are negative, while Tan(Alpha) becomes positive as both the opposite and adjacent sides are negative.
- 😀 In Quadrant IV, Sin(Alpha) and Tan(Alpha) are negative, while Cos(Alpha) is positive, following the same principles as the other quadrants.
- 😀 Special angles (like 30°, 45°, and 60°) are used to calculate trigonometric values across different quadrants using formulas such as Sin(180 - Alpha), Cos(180 - Alpha), and Tan(180 - Alpha) for angles beyond 90°.
Q & A
What is the purpose of dividing the Cartesian coordinate system into four quadrants?
-The Cartesian coordinate system is divided into four quadrants to help understand the signs of the coordinates (x, y) and to define the trigonometric ratios for different angles. Each quadrant has specific rules for determining the signs of sine, cosine, and tangent functions.
How do you define the trigonometric ratios for an angle in the first quadrant?
-In the first quadrant, the coordinates of a point P(x, y) are used to define the trigonometric ratios. The radius r is calculated using the Pythagorean theorem: r = √(x² + y²). The sine, cosine, and tangent ratios are then defined as Sin(α) = y/r, Cos(α) = x/r, and Tan(α) = y/x.
What happens to the signs of the trigonometric functions in the second quadrant?
-In the second quadrant, the sine function remains positive, while the cosine function becomes negative. The tangent function is also negative, as it is the ratio of sine to cosine.
How are the trigonometric functions in the third quadrant different from those in the first quadrant?
-In the third quadrant, both sine and cosine are negative because the x and y coordinates are negative in this quadrant. However, the tangent function becomes positive, as it is the ratio of sine to cosine (negative divided by negative equals positive).
What are the reciprocal functions of sine, cosine, and tangent, and how are they calculated?
-The reciprocal functions of sine, cosine, and tangent are cosecant (csc), secant (sec), and cotangent (cot), respectively. They are calculated as follows: csc(α) = r/y, sec(α) = r/x, and cot(α) = x/y.
How does the sign of cosine change in the second quadrant compared to the first quadrant?
-In the second quadrant, the cosine function becomes negative. This is because the x-coordinate is negative in this quadrant, while the y-coordinate is positive. In the first quadrant, both the x and y coordinates are positive, so cosine is positive.
What identity is used to calculate the sine and cosine of angles greater than 90° but less than 180°?
-The identity used for angles between 90° and 180° is Sin(180° - α) = Sin(α) and Cos(180° - α) = -Cos(α). This helps to determine the sine and cosine values in the second quadrant.
How do you calculate the sine, cosine, and tangent of angles in the third quadrant?
-In the third quadrant, the sine and cosine functions are negative, and the tangent function is positive. The formulas are: Sin(α) = -y/r, Cos(α) = -x/r, and Tan(α) = y/x.
What is the rule for determining trigonometric values for angles between 180° and 270°?
-For angles between 180° and 270°, the sine and cosine functions are both negative, but the tangent function remains positive. The general identities are Sin(180° + α) = -Sin(α), Cos(180° + α) = -Cos(α), and Tan(180° + α) = Tan(α).
How are trigonometric functions calculated for angles between 270° and 360°?
-For angles between 270° and 360° (fourth quadrant), sine becomes negative, cosine is positive, and tangent is negative. The identities are Sin(360° - α) = -Sin(α), Cos(360° - α) = Cos(α), and Tan(360° - α) = -Tan(α).
Outlines

此内容仅限付费用户访问。 请升级后访问。
立即升级Mindmap

此内容仅限付费用户访问。 请升级后访问。
立即升级Keywords

此内容仅限付费用户访问。 请升级后访问。
立即升级Highlights

此内容仅限付费用户访问。 请升级后访问。
立即升级Transcripts

此内容仅限付费用户访问。 请升级后访问。
立即升级浏览更多相关视频

TRIGONOMETRI #3 (perbandingan trigonometri di berbagai kuadran)

Cara Mudah Menentukan Nilai Trigonometri Sudut Istimewa Semua Kuadran

Identitas Trigonometri: Identitas Kebalikan, Perbandingan dan Pythagoras - SMA Kelas 10
Matematika SMA - Trigonometri (1) - Pengenalan Trigonometri, Perbandingan Trigonometri (A)
Tanda Fungsi Trigonometri Tiap Kuadran | Matematika Tingkat Lanjut SMA Kelas XI Kurikulum Merdeka

TRIGONOMETRI - Ukuran Sudut dan Perbandingan Trigonometri
5.0 / 5 (0 votes)
