Cara Mudah Menentukan Nilai Trigonometri Sudut Istimewa Semua Kuadran

Summary

In this video, the presenter explains an easy method to determine the trigonometric values for special angles in all four quadrants. By understanding basic concepts such as the division of the 360° circle into quadrants and which trigonometric functions are positive in each quadrant, viewers can easily calculate sine, cosine, tangent, and their reciprocals. The video also provides a memorable trick to help recall the positive functions in each quadrant and offers step-by-step examples of determining trigonometric values for angles in the second, third, and fourth quadrants. This approach eliminates the need for memorizing all values.

Takeaways

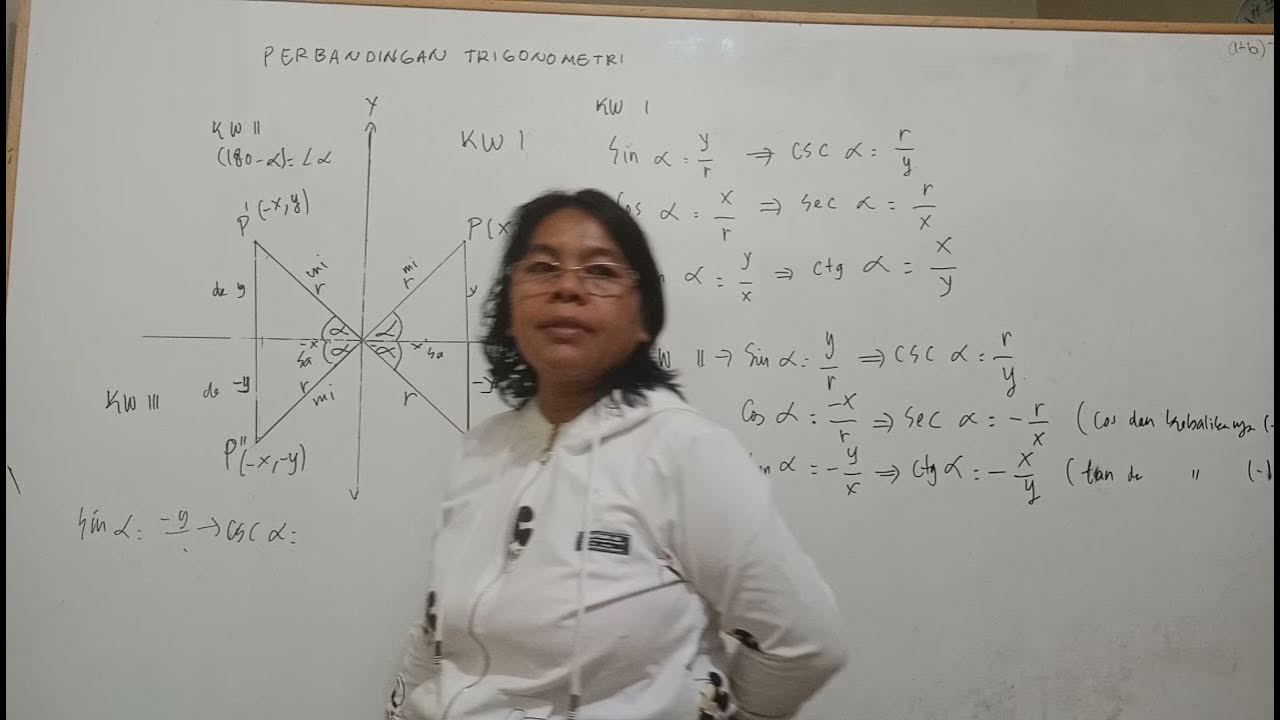
• 😀 The 360° circle is divided into four quadrants, each with specific trigonometric function rules.
• 😀 In Quadrant 1 (0° to 90°), all trigonometric functions (sin, cos, tan, sec, cosecant, cotangent) are positive.
• 😀 In Quadrant 2 (90° to 180°), only sin and cosecant are positive.
• 😀 In Quadrant 3 (180° to 270°), tan and cotangent are positive.
• 😀 In Quadrant 4 (270° to 360°), only cos and sec are positive.
• 😀 The phrase 'semanis Shinta tanpa kosmetik' is a memory aid for remembering the signs of trigonometric functions in each quadrant.
• 😀 Special angles in Quadrant 1 include 0°, 30°, 45°, 60°, and 90°.
• 😀 The sine, cosine, and tangent values for these special angles in Quadrant 1 are clearly explained (e.g., sin 30° = 1/2, cos 45° = √2/2, etc.).
• 😀 To determine the trigonometric value in other quadrants, first identify the quadrant, then reference the corresponding special angle from Quadrant 1.
• 😀 Example calculations show how to determine the values for angles like cos 150°, cos 225°, and sin 240° using the quadrant method.
• 😀 The script emphasizes that memorizing trigonometric values in Quadrant 1 is essential for calculating values in all other quadrants.

Q & A

What is the purpose of the video?

The video aims to teach viewers an easy way to determine the trigonometric values of special angles across all four quadrants, without having to memorize every value.

How is the circle divided into quadrants?

A full circle of 360° is divided into four quadrants: Quadrant 1 (0° to 90°), Quadrant 2 (90° to 180°), Quadrant 3 (180° to 270°), and Quadrant 4 (270° to 360°).

What is the mnemonic to remember the signs of trigonometric functions in each quadrant?

The mnemonic is 'Semanis Shinta Tanpa Kosmetik'. 'Semanis' means all functions are positive in Quadrant 1, 'Shinta' means sin is positive in Quadrant 2, 'Tanpa' indicates tan is positive in Quadrant 3, and 'Kosmetik' means cos is positive in Quadrant 4.

What are the special angles in Quadrant 1, and how are their sine values determined?

The special angles in Quadrant 1 are 0°, 30°, 45°, 60°, and 90°. Their sine values are: sin 0° = 0, sin 30° = 1/2, sin 45° = √2/2, sin 60° = √3/2, and sin 90° = 1.

How are cosine values related to sine values in Quadrant 1?

Cosine values are the reciprocals of sine values. For example, cos 0° = 1, cos 30° = √3/2, cos 45° = √2/2, cos 60° = 1/2, and cos 90° = 0.

What is the relationship between tangent and other trigonometric functions in Quadrant 1?

Tangent is the ratio of sine to cosine. For example, tan 30° = 1/√3, tan 45° = 1, and tan 60° = √3.

How are trigonometric values for angles in Quadrants 2, 3, and 4 determined?

To find trigonometric values in Quadrants 2, 3, and 4, you first determine the reference angle (the angle’s distance from the nearest x-axis), then apply the appropriate sign based on the quadrant's mnemonic.

How would you calculate cos 150°?

Cos 150° is in Quadrant 2, where cosine is negative. The reference angle is 30°, so cos 150° = -cos 30° = -√3/2.

What is the significance of reference angles in trigonometry?

Reference angles are the acute angles formed with the x-axis in each quadrant. They allow for easier computation of trigonometric values by relating the angle in the quadrant to an angle in Quadrant 1.

What happens when an angle exceeds 360° or is less than 0°?

When an angle exceeds 360° or is less than 0°, you subtract or add full circles (multiples of 360°) to bring the angle within the range of 0° to 360°. For example, 570° becomes 210°, and 396° becomes 30°.