Random variables | Probability and Statistics | Khan Academy
Summary
TLDRIn this video, the concept of random variables is introduced, highlighting their role in mapping outcomes of random processes (like flipping a coin or rolling dice) to numbers. Unlike traditional algebraic variables, random variables can take on multiple values with different probabilities, simplifying complex probability calculations. The video demonstrates how to define random variables, use mathematical notation for easier calculations, and emphasizes their importance in probability theory. The speaker illustrates this with examples like defining a random variable for coin flips or the sum of dice rolls, with an introduction to the types of random variables to be explored in the next video.
Takeaways
- 😀 Random variables map the outcomes of random processes to numerical values.
- 😀 A random variable is not like traditional variables from algebra, which represent fixed quantities.
- 😀 In the coin flip example, the random variable X is defined as 1 for heads and 0 for tails.
- 😀 Random variables can be defined in many ways, such as mapping 100 to heads and 703 to tails, depending on the context.
- 😀 The process of quantifying random outcomes allows for more advanced mathematical operations.
- 😀 Random variables enable the use of cleaner probability notation, like P(Y ≤ 30), instead of lengthy expressions.
- 😀 By defining random variables, you can calculate the probability of complex events more efficiently.
- 😀 Traditional variables in algebra are fixed, while random variables can take many values with different probabilities.
- 😀 Probabilities are used to describe the behavior of random variables, such as the likelihood of Y being even.
- 😀 The next steps in studying random variables involve exploring the different types and their properties.
Q & A
What is a random variable?
-A random variable is a way to map the outcomes of a random process to numerical values. For example, when flipping a coin, a random variable might assign a value of 1 for heads and 0 for tails.
How are random variables different from traditional algebraic variables?
-Traditional algebraic variables, like x or y, can be directly assigned specific values or solved for. In contrast, random variables take on different values based on the outcomes of random processes, and these values are associated with probabilities rather than being fixed.
Can random variables take on any value?
-Random variables can take on many different values, but these values are associated with probabilities. The values are not directly assigned or solved for like in algebra, but are determined by the outcomes of random events.
What is the purpose of defining random variables?
-Defining random variables helps quantify the outcomes of random processes, making it easier to apply mathematical operations and compute probabilities. It simplifies the analysis of random events.
What is an example of defining a random variable for a coin flip?
-An example would be defining a random variable X as follows: X = 1 if the coin lands heads, and X = 0 if the coin lands tails.
How could you define a random variable for the sum of multiple dice rolls?
-You could define a random variable Y to represent the sum of the upward faces after rolling multiple dice. For example, if rolling 7 dice, Y would be the sum of the values shown on the top face of each die.
Why is the notation for random variables simpler than writing out full descriptions of random events?
-Using random variables allows for shorter and clearer notation. Instead of writing out the entire description of a random event, we can use a variable like Y and write something simpler, such as P(Y ≤ 30), to describe the probability of a sum being less than or equal to 30.
Can random variables only be used with simple processes like coin flips?
-No, random variables can be used for a wide range of random processes, such as rolling dice, measuring rainfall, or any event where outcomes can be quantified and associated with probabilities.
How does defining random variables help in probability calculations?
-By defining random variables, you can use mathematical notation to express complex probabilistic events more simply. It allows you to focus on probabilities of values or properties of random events without needing to restate the entire scenario each time.
What happens when a random variable takes on multiple values? How are they handled?
-When a random variable takes on multiple values, each value has an associated probability. Instead of assigning a single value, we calculate the likelihood of each possible value occurring, which allows for a more comprehensive analysis of the random process.
Outlines

此内容仅限付费用户访问。 请升级后访问。
立即升级Mindmap

此内容仅限付费用户访问。 请升级后访问。
立即升级Keywords

此内容仅限付费用户访问。 请升级后访问。
立即升级Highlights

此内容仅限付费用户访问。 请升级后访问。
立即升级Transcripts

此内容仅限付费用户访问。 请升级后访问。
立即升级浏览更多相关视频
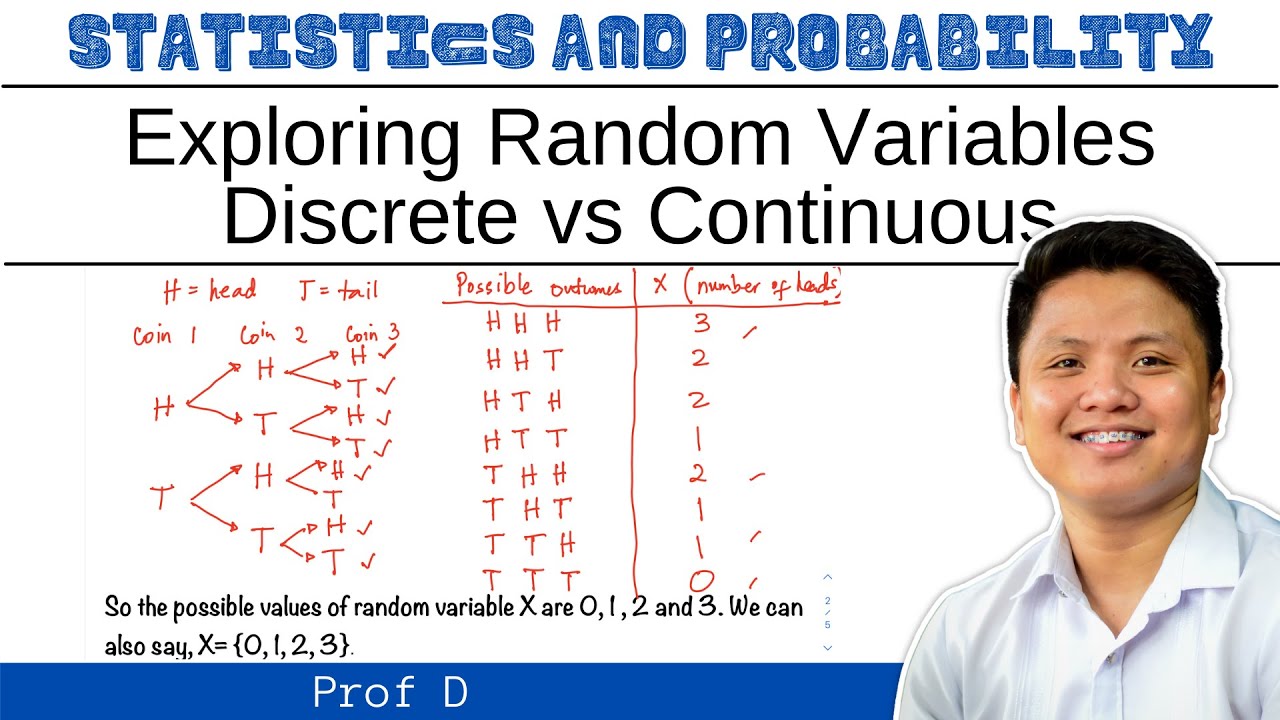
EXPLORING RANDOM VARIABLES | DISCRETE AND CONTINUOUS | PROF D
Random Variables - Grade 11 (Statistics and Probability) @MathTeacherGon
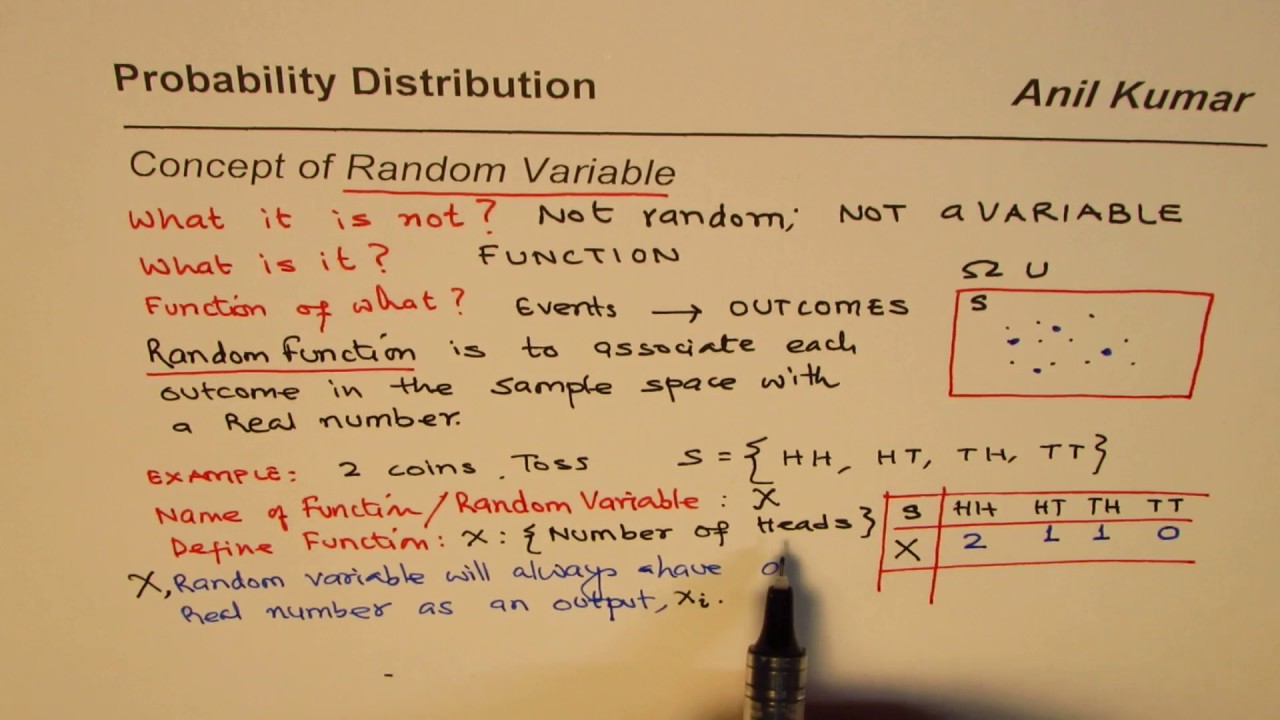
Introduction to Random Variables Probability Distribution

Aula 08 - Parte 1

Distribusi Binomial • Part 1: Variabel Acak

Experimento Aleatorio, Espacio Muestral, Evento o Suceso y Probabilidades
5.0 / 5 (0 votes)
