Quantum Mechanics and the Schrödinger Equation
Summary
TLDRIn this video, Professor Dave explains the Schrödinger equation and its relevance to quantum mechanics. He begins by discussing wave-particle duality and how matter, including electrons, exhibits wave-like properties. Focusing on the electron as a circular standing wave, he explains how quantized energy levels arise. The video also covers how Schrödinger's equation describes the wave function of quantum systems and introduces the concept of probability amplitude proposed by Max Born. Finally, it touches on various interpretations of quantum mechanics, like the Copenhagen and many-worlds interpretations, without endorsing a particular view.
Takeaways
- 🔬 The Schrodinger equation is crucial for describing quantum systems, just as F = ma applies to Newtonian systems.
- 💡 Energy is quantized, and light has both wave and particle characteristics, which also applies to matter, including electrons.
- 🌀 An electron in an atom can be seen as a circular standing wave, leading to quantized energy levels due to the need for an integer number of wavelengths.
- ⚛️ The wave nature of the electron explains why it can only occupy specific energy levels in an atom.
- 📈 When an electron absorbs energy, it moves to a higher energy level by increasing the number of wavelengths in its standing wave.
- 🔗 Orbital overlap, driven by the constructive interference of standing waves, explains covalent bonding.
- 🧮 The Schrodinger equation calculates the wave function, which describes the system's state in quantum mechanics.
- 📊 Max Born proposed that the wave function represents a probability amplitude, with the square of its magnitude indicating the probability of an electron's position.
- 🎯 The wave function's probabilistic nature means we can't predict exact outcomes for individual electrons, only the likelihood of certain behaviors.
- 🌌 There are various interpretations of quantum mechanics, such as the Copenhagen interpretation and the many-worlds interpretation, but no consensus on which is correct.
Q & A
What is the key significance of the Schrodinger equation in quantum mechanics?
-The Schrodinger equation describes the wave function of quantum systems, much like F = ma describes classical systems. It allows us to calculate the probability amplitude of finding a particle in a particular state.
How does the concept of wave-particle duality extend to matter?
-Wave-particle duality, initially applied to light, was extended to matter by de Broglie. This means that all matter, from tiny electrons to large objects, exhibits both wave-like and particle-like properties.
Why is the wavelength of larger objects, such as humans or stars, negligible?
-An object's wavelength is inversely proportional to its mass. For larger objects, like humans or stars, the wavelength becomes so tiny that it has no observable effects, making it negligible.
How does the concept of a standing wave apply to an electron in an atom?
-An electron in an atom can be viewed as a circular standing wave surrounding the nucleus. This explains why energy levels are quantized since only standing waves with an integer number of wavelengths can exist.
What happens when an electron absorbs energy from a photon?
-When an electron absorbs energy from a photon, it gets promoted to a higher energy state, corresponding to an increase in the number of wavelengths in its standing wave.
How does the wave behavior of electrons explain covalent bonding?
-Covalent bonding is explained by the constructive interference of standing electron waves, allowing orbital overlap, which results in the sharing of electrons between atoms.
What is the role of the Hamiltonian operator in the Schrodinger equation?
-The Hamiltonian operator in the Schrodinger equation represents the total energy of a system, taking into account all the interactions affecting the state of the particle.
How did Max Born interpret the wave function in quantum mechanics?
-Max Born proposed that the wave function should be interpreted as a probability amplitude, where the square of the wave function’s magnitude represents the probability of finding a particle in a particular location.
What does the double slit experiment reveal about the nature of electrons?
-The double slit experiment shows that the electron's wave function is probabilistic. The diffraction pattern is not the electron itself but rather a representation of the probability of the electron arriving at different locations on a screen.
Why is quantum mechanics viewed as probabilistic, and how does it differ from classical physics?
-Quantum mechanics is probabilistic because the wave function only gives the likelihood of where a particle might be found, not a definite position. This contrasts with classical physics, where systems can be described with certainty, such as the position and velocity of objects.
Outlines

此内容仅限付费用户访问。 请升级后访问。
立即升级Mindmap

此内容仅限付费用户访问。 请升级后访问。
立即升级Keywords

此内容仅限付费用户访问。 请升级后访问。
立即升级Highlights

此内容仅限付费用户访问。 请升级后访问。
立即升级Transcripts

此内容仅限付费用户访问。 请升级后访问。
立即升级浏览更多相关视频
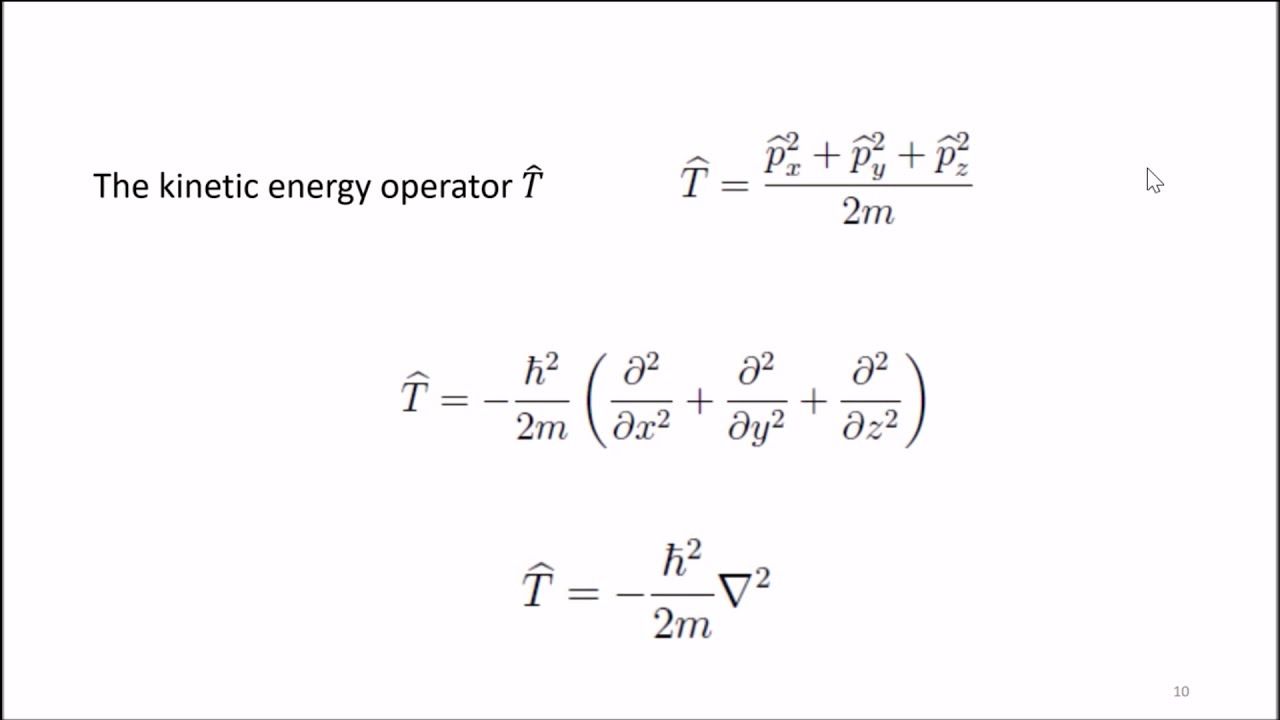
7 Postulates of Quantum Mechanics
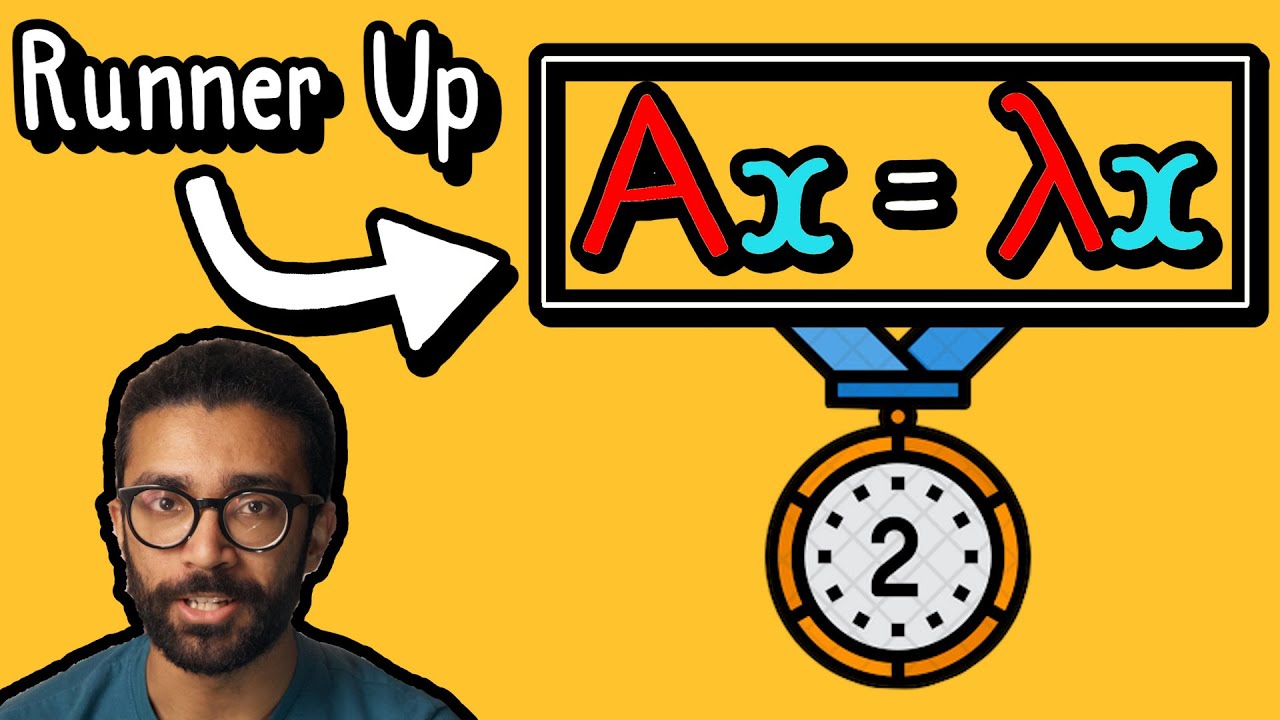
The SECOND Most Important Equation in Quantum Mechanics: Eigenvalue Equation Explained for BEGINNERS
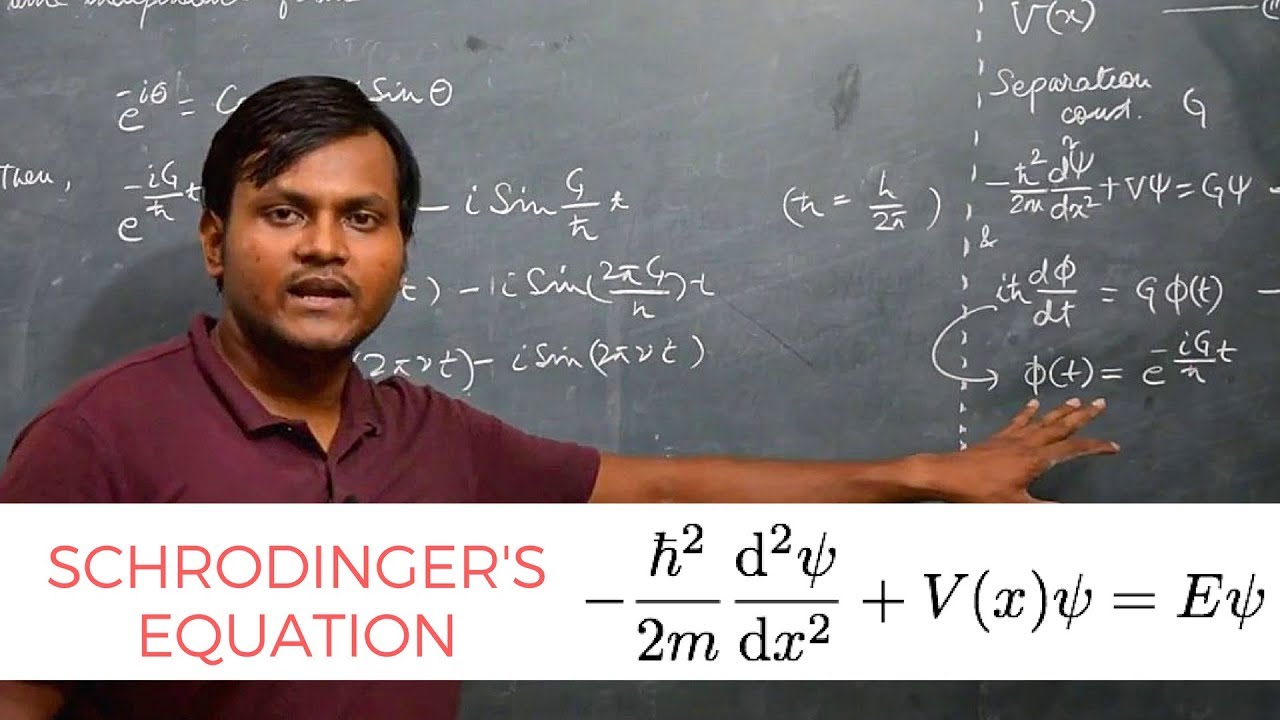
Derive Time Independent SCHRODINGER's EQUATION from Time Dependent one

A Classical Analogy for Schrödinger's Equation

Linearity and nonlinear theories. Schrödinger's equation

What is Quantum Tunneling, Exactly?
5.0 / 5 (0 votes)
