FÁCIL e RÁPIDO | ARCO DUPLO EM 9 MINUTOS
Summary
TLDRThis video covers three key exercises on double-angle identities in trigonometry, aiming to help viewers solve related questions with ease. The instructor walks through concepts like sine, cosine, and tangent of double angles, using examples and triangle diagrams to clarify. Key tips include remembering trigonometric formulas and visualizing problems with triangles. Practical examples are provided, such as calculating sine, cosine, and tangent for given values, as well as solving a specific triangle problem using the tangent identity. The video encourages viewers to take notes and practice for mastery.
Takeaways
- 📚 The video covers three key exercises on double-angle identities in trigonometry, focusing on how to solve problems involving them.
- 👍 The instructor encourages viewers to like and subscribe for more content.
- ✍️ A helpful tip is to write down the formulas and stick them on the wall for easier memorization.
- 📝 The video demonstrates how to find the sine, cosine, and tangent of 2x using the formulas for double angles.
- 🔢 Example: Given sin(x) = 3/5, the video shows how to solve for cos(x) using the Pythagorean theorem, resulting in cos(x) = 4/5.
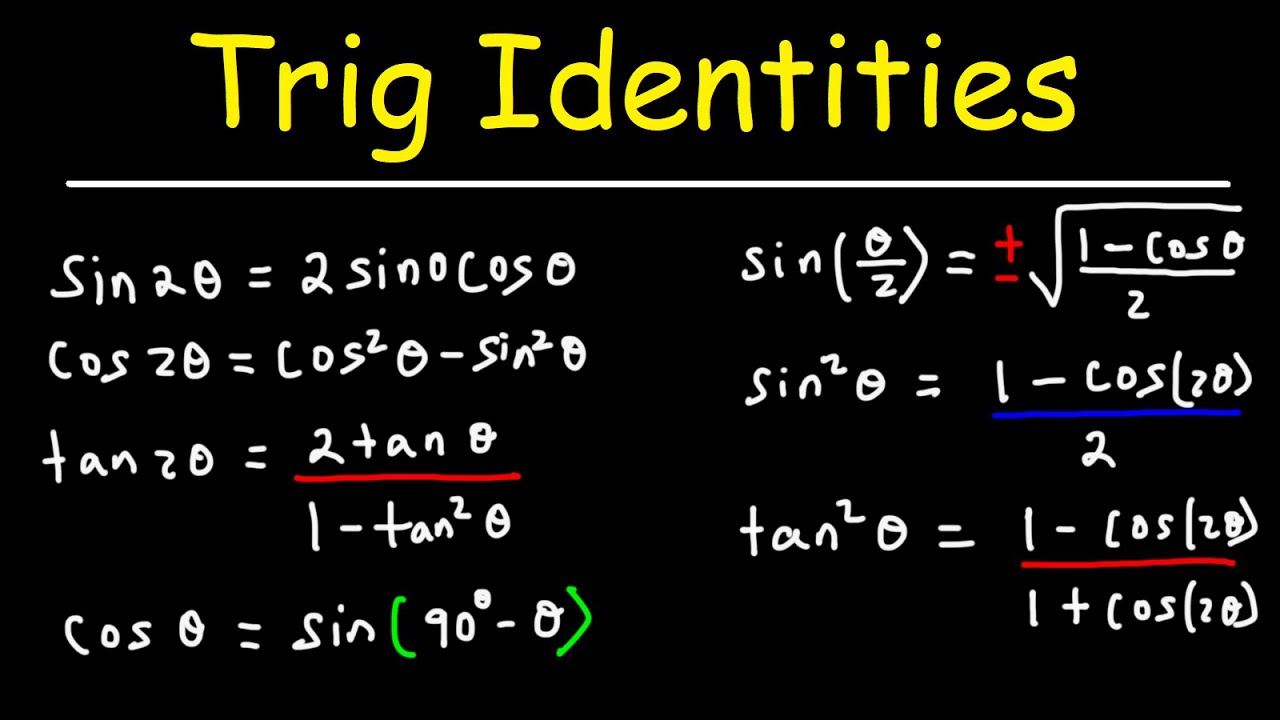
- ✅ The double-angle identity for sine, sin(2x) = 2sin(x)cos(x), is applied to solve example problems.
- 🔗 The video explains the double-angle identity for cosine: cos(2x) = cos²(x) - sin²(x), with step-by-step calculations.
- 🧠 The double-angle identity for tangent, tan(2x) = 2tan(x) / (1 - tan²(x)), is applied with an example using given values for tan(x).
- 🧮 The instructor emphasizes the importance of correctly applying formulas to avoid mistakes in exams.
- 📐 The video ends with a challenging problem involving a right triangle and double-angle identities, encouraging viewers to practice solving it.
Q & A
What mathematical topic is the video primarily discussing?
-The video focuses on solving trigonometric problems involving double-angle formulas for sine, cosine, and tangent.
What is the double-angle formula for sine as presented in the video?
-The double-angle formula for sine is given as sin(2x) = 2 * sin(x) * cos(x).
How is the cosine of an angle derived using a right triangle in the video?
-The cosine of an angle is calculated using the formula cos(x) = adjacent/hypotenuse. For an example triangle, the adjacent side is 4, and the hypotenuse is 5, resulting in cos(x) = 4/5.
What is the process for finding the adjacent side using the Pythagorean theorem in this context?
-The adjacent side is found using the Pythagorean theorem: hypotenuse² = opposite² + adjacent². In the example, if the opposite side is 3 and the hypotenuse is 5, the adjacent side is calculated as 4.
How does the video suggest handling fraction operations when working with trigonometric formulas?
-The video recommends simplifying fractions by finding a common denominator when subtracting or adding fractions. It also explains how to simplify expressions like 16/25 - 9/25 by subtracting the numerators and keeping the common denominator.
What is the double-angle formula for cosine, and how is it applied?
-The double-angle formula for cosine is cos(2x) = cos²(x) - sin²(x). The video applies this by squaring the values of cos(x) and sin(x), then subtracting the results.
How is the double-angle formula for tangent derived and used?
-The double-angle formula for tangent is tan(2x) = 2 * tan(x) / (1 - tan²(x)). The video demonstrates how to use this formula by first calculating tan(x) as opposite/adjacent, then substituting into the formula.
What method does the video suggest to solve for the sine of 2x when given sin(x) + cos(x) = 2/3?
-The video suggests squaring both sides of the equation sin(x) + cos(x) = 2/3, then using the identity sin²(x) + cos²(x) = 1 to simplify the expression. It eventually solves for sin(2x) using the double-angle formula.
What is the significance of the 'SOH CAH TOA' mnemonic in the video?
-'SOH CAH TOA' is used to help remember the basic trigonometric ratios: sine = opposite/hypotenuse, cosine = adjacent/hypotenuse, and tangent = opposite/adjacent. The video uses this mnemonic when solving for sine, cosine, and tangent.
How does the video explain the process of solving a problem involving a triangle with a bisector?
-The video explains that to solve for the unknown side (x) in a triangle with a bisector, you can use the tangent function. It sets up the equation for tan(2α) and uses it to find the value of x through algebraic manipulation and substitution.
Outlines

此内容仅限付费用户访问。 请升级后访问。
立即升级Mindmap

此内容仅限付费用户访问。 请升级后访问。
立即升级Keywords

此内容仅限付费用户访问。 请升级后访问。
立即升级Highlights

此内容仅限付费用户访问。 请升级后访问。
立即升级Transcripts

此内容仅限付费用户访问。 请升级后访问。
立即升级浏览更多相关视频
Trig Identities

Trigonometry | One Shot Revision | Class 10 | Haripriya Mam | Vedantu Telugu

TRIGONOMETRI SUDUT ISTIMEWA

Trigonometry One Shot Concept in 10 Minutes | CBSE Class 10 Maths Chapter 8 | Jhatpat Gyaan

Double Angle Identities & Formulas of Sin, Cos & Tan - Trigonometry
GEOMETRIA PLANA: SOMA DOS ÂNGULOS INTERNOS (α + β) #IF2021
5.0 / 5 (0 votes)
