Simplifying Radicals With Variables, Exponents, Fractions, Cube Roots - Algebra

Summary

This educational video script offers a comprehensive guide on simplifying radicals with variables and exponents. It explains the process of simplifying square roots and higher roots by breaking down the exponents and identifying perfect squares or cubes, using examples like simplifying the square root of 32 and cube root of 16. The script also covers handling expressions with multiple variables and demonstrates the use of absolute values for even indices with odd exponents. It concludes with solving complex radical expressions, emphasizing the importance of rationalizing denominators and simplifying to the most reduced form.

Takeaways

• 📚 The video focuses on simplifying radicals with variables and exponents, providing various methods to achieve this.
• 🔍 Simplifying the square root of a variable raised to an exponent involves breaking down the exponent by the index number, as shown with \( \sqrt{x^5} \) simplified to \( x^2\sqrt{x} \).
• ✂️ Another approach to simplifying radicals is by dividing the exponent by the index to determine how many times the index fits into the exponent and what remains.
• 📉 For radicals with a number, such as \( \sqrt{32} \), the number is broken down into a product of a perfect square and another number, simplifying to \( 4\sqrt{2} \).
• 📶 When simplifying expressions with multiple variables and exponents, such as \( \sqrt{50x^3y^{18}} \), divide the exponents by the index and simplify the radical part separately.
• 💡 The use of absolute values is mentioned for cases with an even index and an odd exponent resulting from the radical simplification, which may be required by some teachers.
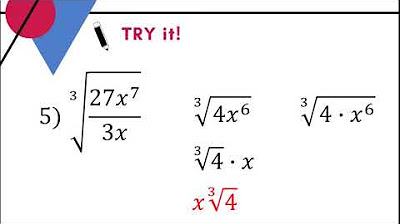
• 📐 The cube root of a variable to a certain power can be simplified by dividing the power by the index, as demonstrated with \( \sqrt[3]{x^5} \) becoming \( x^2 \) inside the radical.
• 🔑 Simplifying complex expressions with multiple variables and exponents in the numerator and denominator involves simplifying each part and then combining them, considering the division of exponents.
• 📘 Rationalizing the denominator is necessary when the denominator contains a radical, as shown in the final example where the denominator is multiplied by the radical's conjugate.
• 🔄 The process of simplifying radicals involves breaking down the expression into simpler components, simplifying each part, and then recombining them.
• 🎓 The video concludes by reinforcing the method of simplifying radicals with variables and exponents, emphasizing the importance of understanding the process for various mathematical problems.

Q & A

What is the main focus of the video?

The video focuses on how to simplify radicals with variables and exponents.

How can you simplify the square root of x to the fifth power?

You can simplify it by writing x to the power of 5/2, which results in x squared times the square root of x.

What is the method to determine how many times the index number goes into the exponent when simplifying radicals?

You divide the exponent by the index number to see how many times it goes evenly, and then determine the remainder to simplify the radical.

How is the square root of 32 simplified in the video?

It is simplified by breaking down 32 into 16 and 2, where the square root of 16 is 4, resulting in 4 times the square root of 2.

What is the purpose of using absolute values when simplifying radicals with even indices and odd exponents?

Absolute values are used to ensure the result is non-negative, as the outcome of an even index radical with an odd exponent can be negative.

How can you simplify the square root of 50 when it includes variables with exponents?

You break down 50 into 25 and 2, and since the square root of 25 is 5, the expression simplifies to 5 times the square root of 2.

What is the process for simplifying the cube root of x to the fifth, y to the ninth, and z to the fourteenth?

You determine how many times 3 goes into each exponent (5, 9, 14), simplify accordingly, and then place the remaining exponents inside the radical.

How does the video simplify the cube root of 16 with variables to higher powers?

The video breaks down 16 into 8 and 2, simplifies the cube root of 8 to 2, and leaves the 2 inside the radical, resulting in 2 times the cube root of 2.

What is the final step in simplifying the complex radical expression involving square roots and cube roots of numbers and variables?

The final step is to rationalize the denominator by multiplying the numerator and denominator by the square root of the number in the denominator.

How does the video handle the division of variables with exponents in a radical?

The video subtracts the exponents of the variables when they are divided within a radical, placing the result with the appropriate variable.

Outlines

00:00

📚 Simplifying Radicals with Variables and Exponents

This paragraph introduces the concept of simplifying radicals that include variables raised to various exponents. It explains the process of simplifying the square root of x to the fifth power by breaking it down into x squared times the square root of x. The method involves determining how many times the index number (in this case, 2) fits into the exponent, simplifying the expression accordingly. The paragraph also discusses an alternative approach to simplification and provides examples with different exponents, illustrating how to handle cases with no remainder and those with a remainder, ultimately simplifying the radicals to their most reduced form.

05:02

🔍 Advanced Radical Simplification Techniques

The second paragraph delves into more complex examples of radical simplification, including handling perfect squares and non-perfect squares, as well as variables with exponents. It demonstrates how to break down numbers into components that include perfect squares to simplify the radical expression. The paragraph also addresses the use of absolute values in cases where an even index results in an odd exponent after simplification, as per some educational requirements. Examples are given to show the step-by-step simplification process, including dividing exponents and rationalizing denominators, to arrive at the final simplified form of the expression.

10:03

📘 Final Thoughts on Radical Simplification

The final paragraph wraps up the video script by summarizing the process of simplifying radicals with variables and exponents. It emphasizes the importance of understanding how to break down radicals into their simplest form, whether dealing with perfect cubes or other exponents. The paragraph concludes with a reminder that the need for absolute values depends on the index and the resulting exponent after simplification. It ends on a positive note, encouraging viewers to apply these techniques to further problems and wishing them well, thus closing the educational segment on radical simplification.

Keywords

💡Radicals

Radicals are mathematical expressions involving roots, such as square roots or cube roots, which are used to find the quantity that, when raised to a certain power, gives the original number. In the video, radicals are the central theme, with the focus on simplifying them with variables and exponents, for example, simplifying the square root of x to the fifth power.

💡Simplify

To simplify in mathematics means to reduce a complex expression to a more straightforward form. The video script provides various methods to simplify radicals, such as breaking down the radicand (the number under the radical sign) into factors that can be easily rooted.

💡Variables

Variables are symbols, often letters, used to represent unknown or changeable quantities in mathematics. The script discusses simplifying radicals that contain variables raised to different powers, showing how to handle expressions like the square root of 'x to the fifth'.

💡Exponents

Exponents are used to denote repeated multiplication of the same number, indicating how many times a base number is multiplied by itself. The video explains how to simplify radicals with variables that have exponents, such as simplifying the square root of 'x^5' to 'x^2 * sqrt(x)'.

💡Index Number

The index number in a radical indicates the root's degree, such as a square root (index 2) or cube root (index 3). The script explains how the index number affects the simplification process, as seen when determining how many times the index divides into the exponent.

💡Perfect Square

A perfect square is a number that can be expressed as the square of an integer. In the context of the video, breaking down a number into a perfect square and another number simplifies the process of taking the square root, as demonstrated with the square root of 32 being simplified to 4 * sqrt(2).

💡Absolute Value

Absolute value denotes the distance of a number from zero on a number line, disregarding its sign. The script mentions using absolute value in certain cases when simplifying radicals with an even index and an odd exponent to ensure the result is non-negative.

💡Rationalize the Denominator

Rationalizing the denominator is the process of eliminating radicals from the denominator of a fraction to make it a rational number. The video gives an example where the denominator contains a radical, and it is multiplied by a form of one over the same radical to eliminate it.

💡Cube Root

A cube root is a radical that finds the number that, when multiplied by itself three times, gives the original number. The script discusses how to simplify cube roots, such as simplifying the cube root of 'x to the fifth' by determining how many times 3 divides into 5.

💡Divide Exponents

When dividing expressions with the same base, the exponents are divided. The script explains this concept in the context of simplifying complex radical expressions, such as dividing 'x to the seventh' by 'x to the third' results in 'x to the fourth'.

💡Subtract Exponents

Subtracting exponents is a process used in simplifying expressions under radicals, where the exponent outside the radical is reduced by the exponent inside. The video demonstrates this by showing how to simplify 'y to the ninth' divided by 'y to the third' to 'y to the sixth'.

Highlights

Focus on simplifying radicals with variables and exponents.

Simplification of square root of x to the fifth by breaking it down into x squared times the square root of x.

Method of simplifying radicals by determining how many times the index number fits into the exponent.

Example of simplifying square root of x to the seventh by dividing the exponent by the index.

Explanation of simplifying square root of x to the eighth, which results in no remainder when divided by two.

Demonstration of simplifying square root of 32 by breaking it down into perfect squares and non-squares.

Introduction of absolute value in simplifying radicals with even indices and odd exponents.

Simplification of square root of 50 by breaking it down into square root of 25 times the square root of 2.

Process of simplifying cube roots with variables, such as cube root of x to the fifth.

Explanation of how to simplify radicals with multiple variables and exponents, like cube root of x to the fifth y to the fourteenth.

Final problem example involving cube root of 16 with multiple variables and exponents.

Simplification of complex radical expressions with division and exponent subtraction.

Use of absolute values in radicals with even indices and odd exponents after simplification.

Rationalizing the denominator by multiplying numerator and denominator by the square root of the denominator.

Final answer for a complex problem involving radicals with variables and exponents, showcasing the simplification process.

Encouragement for viewers to pause the video and try the example problems themselves.

Conclusion summarizing the method of simplifying radicals with variables and exponents.

Transcripts

in this video we're going to focus on

how to simplify radicals with variables

and exponents

so let's say if you want to simplify the

square root of x to the fifth

the index number is a two now one way

you can do this is you can write x five

times

and because there's a two you need to

take out two at a time

so this will come out as one x

and this will come out as another x

and

you're gonna get x times x square root

of 1 just x by itself

so this is equal to x squared

root x

now another way you can simplify this or

get the same answer

is if you do it this way

how many times does two go into five

two goes into five two times because two

times two is four two times three is six

that's too much

and what's remaining five minus four is

one so you get one remaining

and that's another way you can simplify

it so let's say for example if

you want to simplify the square root of

x to the seven

how many times does

two go into seven two goes into seven

three times with one remaining

now let's try this one

how many times does two go into eight

two goes into eight or eight divided by

two is four

two goes into eight four times with no

remainder

two goes into nine four times and 2 goes

into 12 6 times with no remainder

for the 9 there's a remainder of 1 so

the y is still on the inside that's a

quicker way that you can use to simplify

radicals

now let's say if you have a number let's

say if you want to simplify the square

root of

let's say um 32

what you want to do is break this down

into

two numbers one of which was is a

perfect square

so 32 can be broken down into 16 and 2.

now the reason why i chose 16 and 2 is

because we know what the square root of

16 is and that's 4. and so this is just

4 root 2.

now let's say if we have a problem that

looks like this

let's say if we want to simplify the

square root

of 50

x cubed

y to the 18th

so how many times does two go into three

two goes into three one time

with uh one remaining

and two goes into eighteen nine times

now usually when you have an even index

and an odd exponent you got to put it in

absolute value

now your teacher may not go over this

but some teachers do but just in case if

you have one of those teachers who wants

you to use an absolute value you only

need it if you have an even index and if

you get an odd exponent after it comes

out of the radical

now the only thing we have to simplify

is root 50.

square root 50 we can break it down into

square root 25 and 2 because 25 times 2

is 50.

and the square root of 25 is 5

but the 2 stays inside the radical so we

can put a 5 on the outside and let's put

the 2 inside so this is the final answer

that's how you can simplify

that expression

let's try some other problems so let's

say if we have the cube root of

x to the fifth

y to the knife

and z to the fourteenth

so how many times does three go into

five

three goes into five one time

with uh two remaining so we're gonna put

x squared inside

and the index number would it's going to

stay 3. now how many times is 3 going to

9 9 divided by 3 is 3 with no remainder

and how many times does 3 go into 14

3 goes into 14 four times

and 3 times 4 is 12

so

14 minus 12

is 2 so we have 2 remaining

so that's how you can simplify radicals

let's try one final problem

feel free to pause the video and see if

you can see if you can get the answer

for this one

so the cube root of 16

x to the 14th

y to the 15th

z to the 20th

so how many times does 3 go into 14

3 goes into 14

4 times

3 times 4 is 12 and 14 minus 12 is 2 so

we're going to get x squared on the

inside

3 goes into 15 five times with no

remainder because 15 divided by 3 is 5.

3 goes into 20 six times

3 times 6 is 18.

three doesn't go into twenty evenly

and twenty minus eighteen is two so

there's two remaining

now let's simplify the cube root of

sixteen

perfect cubes are one one cube is one

two to the third power is eight

three to the third power is twenty seven

so a perfect cube

that goes into sixteen is eight so

sixteen divided by eight is two

so you want to write cube root of 16 as

the cube root of 8 times cube root of 2

because the cube root of 8 simplifies to

2.

so this 2 is going to go on the outside

which we're going to put it here

and this 2 remains on the inside which

i'm going to put it there

so this is our final answer for that

problem

so that's how you can simplify radicals

with variables and exponents but

actually let's try one more let's say if

you have a question it looks like this

let's say the square root of

75

x to the seventh

y to the third

z to the tenth

over

eight

let's say x to the third

y to the ninth

z to the fourth

so the first thing we can do is um

let's simplify everything

let's rewrite it

so 75

is uh 25

times three

we can square root 25 that's five but

we'll do that later and eight is four

times two because we can take the square

root of four

now when you divide exponents i mean

when you divide variables you gotta

subtract the exponents seven minus three

is four

and that goes on top because there's

more x values on top

now for this one you can do three minus

nine but

i think it's easy if you subtract it

backwards the nine minus three which is

six

and because we subtract it backwards the

six goes on the bottom

and then ten minus four

so there's more z's on top down the

bottom so we're gonna put it on top so z

to the

sixth and now let's simplify it the

square root of 25

is five

and the square root of four

is two

now two goes into four two times four

divided by two is two so we get x

squared

two goes into six three times so we get

z to the third

and six divided by two is three so we

get y to the third

and inside the radical we still have a

radical three and the square root 2 left

over

so now

we also need to add some absolute values

because we have an even index and we

have a few odd exponents we need to put

z an absolute value

and a y so our last step is to multiply

top and bottom

by square root of two we need to

rationalize the denominator we need to

get rid of that radical

so our answer our final answer is five

x squared absolute value of z to the

third

square root six

over

square root two times square root two is

is square root of four which simplifies

to two and two times two gives us four

so we get four absolute value y cubed

this is our final answer for that

particular problem

okay let's try just one more problem

so let's say if we have

the cube root

of 16

x to the seven

y to the fourth z to the ninth

divided by

54

x

squared y to the knife

z to the 15th

so feel free to pause the video and try

this example yourself

so the first thing i would do is within

a radical

i would divide both numbers by two

referring to the sixteen and the fifty

four

so right now what i have is the cube

root

of

eight

which is a perfect cube over 27. 16

divided by 2 is 8 half of 54 is 27.

so now what i'm going to do is subtract

the exponents 7 minus 2

is 5.

and for the y's i'm going to subtract it

backwards

9 minus 4

is 5.

so y to the fifth

and for z i'm going to subtract it

backwards 15 minus 9

is 6 but that's going to go on the

bottom

and so now we can simplify it

so the cube root of 8

is 2

and 3 goes into

5 only one time

with

two remaining

and the cube root of 27 is three

and three goes into five one time just

like x with two remaining three goes

into six two times so that becomes z

squared

now we don't need any absolute values

because this is an odd index we only

need it for even index numbers that

produce an odd exponent

so now let's simplify what we have

so

we need to get rid of the radical on the

bottom

so we're going to multiply top and

bottom by the cube root of y to the

first power

so what we now have

is two x

cube root

x squared times y

divided by

three y times z squared

times the cube root of y to the third

the cube root of y to the third cancels

and so that becomes y to the first

and y to the first times y to the first

is y squared so our final answer is two

x

cube root

x squared y

over three y squared z squared

and that's it so now you know how to

simplify radicals with variables and

exponents

so that's it for this video thanks for

watching and have a have a wonderful day