Probability, Sample Spaces, and the Complement Rule (6.1)
Summary
TLDRThis video script introduces the fundamental concepts of probability, focusing on sample spaces and the complement rule. It explains how to calculate the probability of events using favorable outcomes and total outcomes, exemplified by coin flips. The script also demonstrates creating sample spaces for multiple coin tosses, calculating joint probabilities, and using the complement rule to find the likelihood of an event not occurring. The importance of probabilities summing to 1 and the range of 0 to 1 for individual events is highlighted, providing a clear foundation for understanding probability.
Takeaways
- 📚 Probability is defined as the chance that an event will occur, calculated as the number of favorable outcomes divided by the total number of possible outcomes.
- 🔄 Flipping a coin has two possible outcomes, heads or tails, each with a 50% chance, making the probability of either outcome 0.5.
- 🎲 The probability of getting two heads when flipping a coin twice is found by multiplying the probabilities of each individual event (0.5 * 0.5 = 0.25 or 25%).
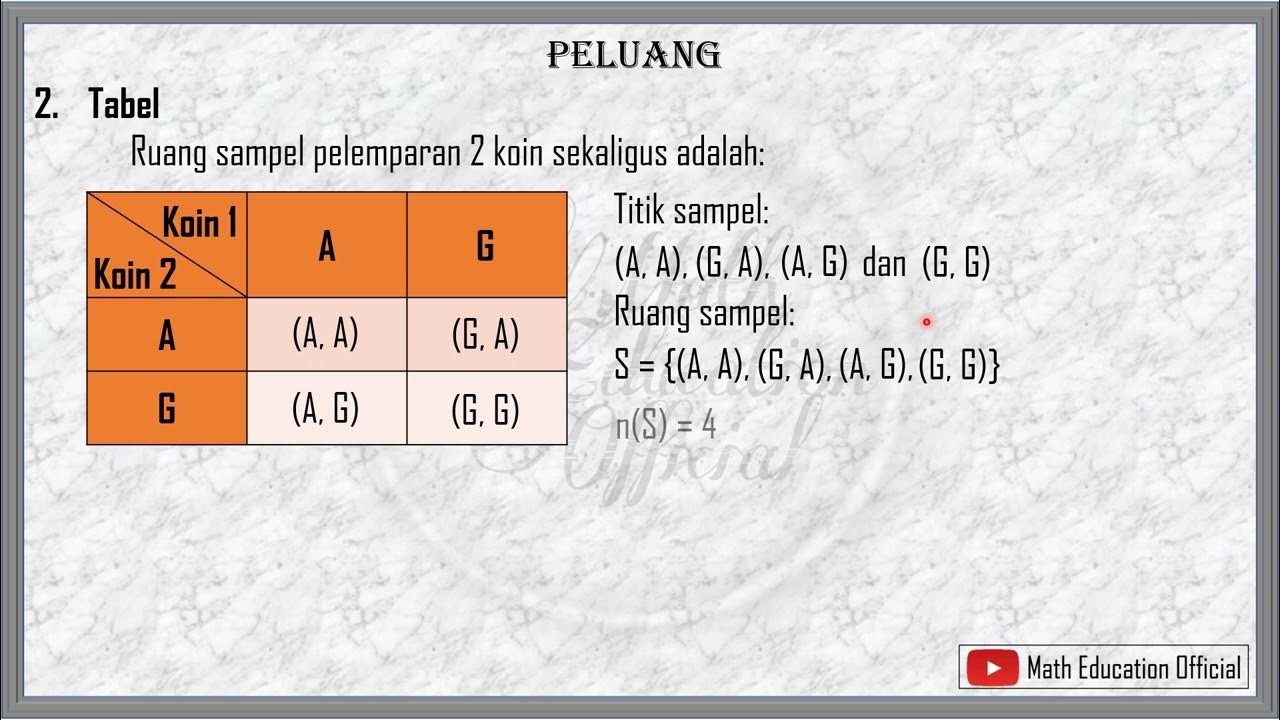
- 🌐 A sample space represents all possible outcomes of an event, like flipping a coin twice, which includes outcomes like HT, TH, TT, and HH.
- 📈 To find the probability of each outcome in a sample space, multiply the probabilities of each event in the sequence.
- 🤔 The concept of 'at least one' in probability involves identifying outcomes that meet the condition and summing their probabilities.
- 📉 The complement rule states that the probability of an event not occurring is 1 minus the probability of it occurring, useful for indirect calculations.
- 🔢 Probability values for all possible outcomes of an event must sum up to 1, ensuring all possibilities are accounted for.
- 🚫 The probability of an event occurring must be between 0 and 1, inclusive, with 0 meaning it will never occur and 1 meaning it will always occur.
- 🔄 The formula for the probability of two independent events A and B occurring together is P(A and B) = P(A) * P(B).
- 🛑 The complement rule can be applied to find the probability of an event not happening, as demonstrated with the example of not getting two tails when flipping two coins.
Q & A
What is the basic definition of probability?
-Probability is defined as the chance that an event will occur, which is calculated as the total number of favorable outcomes divided by the total number of possible outcomes.
What is the probability of getting heads when flipping a fair coin once?
-The probability of getting heads when flipping a fair coin once is 50%, or 0.5, since there is one favorable outcome (getting heads) out of two possible outcomes (heads or tails).
How can you calculate the probability of getting two heads when flipping a coin twice?
-The probability of getting two heads when flipping a coin twice is calculated by multiplying the probability of getting heads on the first flip (0.5) by the probability of getting heads on the second flip (0.5), which equals 0.25 or 25%.
What is a sample space in the context of probability?
-A sample space refers to the entire set of possible outcomes for a given event. In the context of flipping a coin twice, the sample space includes all possible combinations of heads and tails for both flips.
How many possible outcomes are there when flipping a coin twice?
-When flipping a coin twice, there are four possible outcomes, which can be represented as HH (heads-heads), HT (heads-tails), TH (tails-heads), and TT (tails-tails).
How do you determine the probability of each outcome in a sample space?
-To determine the probability of each outcome in a sample space, you multiply the probability of each event in the outcome together. Since each flip of a fair coin has a probability of 0.5 for heads or tails, the probability for each outcome in the sample space is 0.5 * 0.5 = 0.25.
What is the concept of 'at least one' in probability problems, and how is it used?
-The concept of 'at least one' in probability problems refers to the occurrence of an event in at least one of the outcomes. It is used to calculate the probability of an event happening in at least one of the possible scenarios, such as getting at least one tail when flipping a coin twice.
What are the two conditions that all probabilities must satisfy?
-The two conditions that all probabilities must satisfy are: 1) The probability of an event occurring must have a value between 0 and 1, inclusive. 2) The sum of the probabilities of all possible outcomes must equal 1.
What is the complement rule in probability, and how is it applied?
-The complement rule in probability states that the probability that an event does not occur is equal to 1 minus the probability that it will occur. It is applied by subtracting the probability of the event from 1 to find the probability of its complement.
Can you provide an example of using the complement rule from the script?
-An example of using the complement rule from the script is calculating the probability of not getting two tails when flipping two coins. Since the probability of getting two tails (TT) is 0.25, the probability of not getting two tails is 1 - 0.25, which equals 0.75.
How does the script suggest finding the probability of two independent events happening together?
-The script suggests that to find the probability of two independent events happening together, you multiply the probability of the first event by the probability of the second event. This is represented by the formula P(A and B) = P(A) * P(B).
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade Now5.0 / 5 (0 votes)