peluang koin dan dadu

Summary

This video provides an introduction to probability concepts, covering fundamental topics such as sample spaces, events, and probability calculations using dice and coin tosses. It explains how to determine the probability of specific outcomes and introduces the concept of expected frequency by multiplying probability by the number of trials. The video further explores more complex scenarios like rolling two dice or tossing two coins, showing how to calculate combined probabilities. Interactive examples are included to help viewers practice and reinforce their understanding of these key probability concepts.

Takeaways

• 😀 The video introduces the concept of probability, focusing on events such as coin tosses, dice rolls, and combined events.
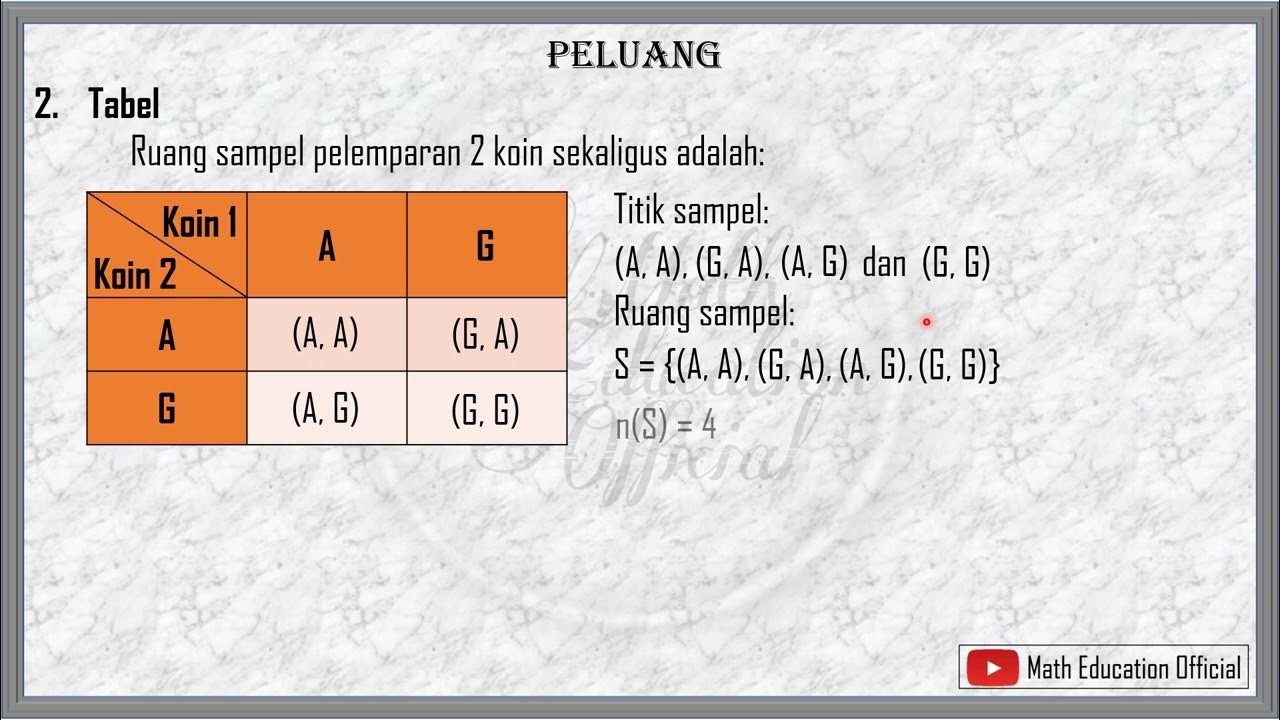
• 😀 The sample space (denoted as S) is the set of all possible outcomes of an experiment, such as the numbers 1-6 for a die roll.
• 😀 Events are subsets of the sample space, with each event having a probability represented by P(E), which is the ratio of favorable outcomes to total outcomes.
• 😀 Probability values range between 0 and 1, with 0 indicating an impossible event and 1 indicating a certain event.
• 😀 Expected frequency is calculated by multiplying the probability of an event by the number of trials, showing the theoretical number of occurrences in a series of experiments.
• 😀 The probability of getting an odd number on a single die roll is 3/6 (or 1/2), as there are 3 odd numbers (1, 3, 5) in the sample space.
• 😀 The probability of getting a number divisible by 2 (even) on a die is 3/6, simplifying to 1/2.
• 😀 When calculating the probability of multiple dice events, the total number of outcomes is the product of the number of possibilities for each die.
• 😀 For combined events like rolling two dice, the total number of outcomes increases, and probabilities can be calculated by counting favorable outcomes.
• 😀 The concept of conditional probability is illustrated with the example of rolling a die and tossing a coin, where the two events are independent, and probabilities are combined based on the rules of each event.

Q & A

What is the concept of 'sample space' in probability?

The sample space refers to the set of all possible outcomes of an experiment. For example, in a dice throw, the sample space would be {1, 2, 3, 4, 5, 6}, representing all the possible results from rolling the dice.

What does 'event' mean in probability?

An event is a specific outcome or set of outcomes from the sample space that we are interested in. For instance, when rolling a die, an event could be rolling an odd number, which corresponds to the outcomes {1, 3, 5}.

How is probability mathematically defined?

Probability is defined as the ratio of the number of favorable outcomes (events) to the total number of possible outcomes in the sample space. The formula is: Probability = (Number of favorable outcomes) / (Total number of outcomes).

What does a probability of 0 signify?

A probability of 0 means that the event is impossible and will not occur. For example, the probability of rolling a 7 on a standard six-sided die is 0 because 7 is not a possible outcome.

What does a probability of 1 mean?

A probability of 1 means that the event is certain and will definitely occur. For example, when tossing a fair coin, the probability of getting either heads or tails is 1, as one of them must occur.

How is expected frequency calculated in probability?

Expected frequency is calculated by multiplying the probability of an event by the number of trials (experiments). For example, if the probability of an event is 1/2 and the experiment is conducted 100 times, the expected frequency would be 1/2 * 100 = 50.

What is the sample space for a dice roll?

The sample space for a single die roll consists of the outcomes {1, 2, 3, 4, 5, 6}, as these are the only possible results when rolling a six-sided die.

How do you calculate the probability of rolling an even number on a die?

The even numbers on a die are 2, 4, and 6. Since there are 6 possible outcomes, the probability of rolling an even number is the ratio of favorable outcomes (3 even numbers) to the total outcomes (6), which is 3/6, or 1/2.

How is the sample space affected when two dice are thrown?

When two dice are thrown, the sample space increases significantly. There are 6 outcomes for each die, so the total number of possible outcomes is 6 * 6 = 36. Each outcome corresponds to a pair of numbers, one from each die.

What is the probability of getting a 'prime' number on the first die and a 'multiple of 3' on the second die?

The prime numbers on a die are 2, 3, and 5, while the multiples of 3 are 3 and 6. There are 3 prime numbers and 2 multiples of 3, so the probability of getting a prime number on the first die and a multiple of 3 on the second die is (3/6) * (2/6) = 6/36, which simplifies to 1/6.