Standard Deviation and Variance

Summary

The video script explains the concept of standard deviation as a measure of data dispersion around the mean. It illustrates the calculation process using an example of people's heights, emphasizing the difference between standard deviation and variance. The script clarifies that the standard deviation is the square root of the sum of squared deviations, divided by the number of observations (n) or n-1 for sample data, to estimate the population's standard deviation. The variance is the square of the standard deviation, making it less intuitive to interpret due to squared units, whereas the standard deviation retains the original data's unit, aiding in easier data interpretation.

Takeaways

• 📊 Standard Deviation is a statistical measure that quantifies the amount of variation or dispersion in a set of data values.
• 🧩 The mean (average) is calculated by summing all data points and dividing by the number of data points.
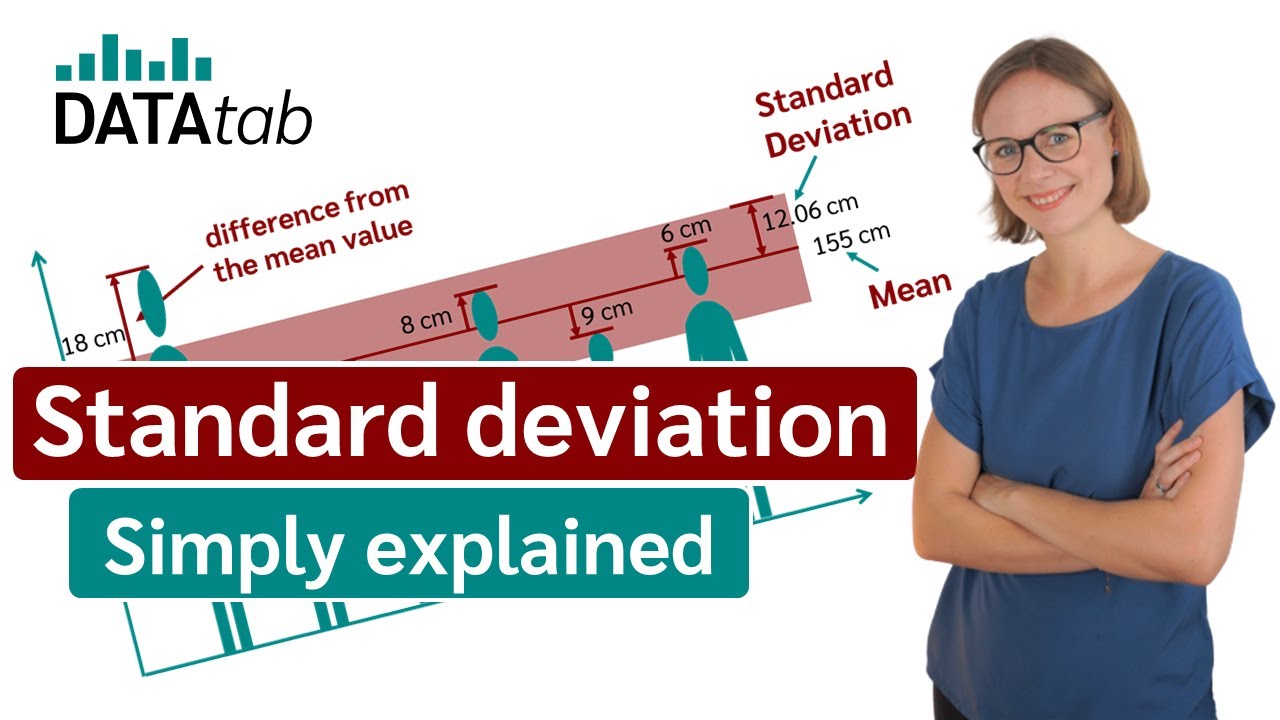
• 📏 Deviation is the difference between each data point and the mean value.
• 🔍 The standard deviation is found by taking the square root of the average of the squared deviations from the mean.
• 🌐 In the example given, the average deviation from the mean is 11.5 centimeters, which is the standard deviation.
• 📘 The standard deviation formula involves summing the squared differences from the mean and dividing by the number of values (n) or (n-1).
• 🔑 The choice between dividing by n or n-1 depends on whether the data represents a population or a sample.
• 🌐 When estimating a population standard deviation from a sample, n-1 is used, which is known as Bessel's correction.
• 🔄 The variance is the square of the standard deviation and is used to quantify the dispersion of data points around the mean.
• 📐 The standard deviation is easier to interpret than the variance because it is in the same unit as the original data, not squared.
• 📋 The script provides a clear explanation of the concepts of standard deviation and variance, and their calculation methods.

Q & A

What is the standard deviation?

The standard deviation is a measure that indicates how much data scatter around the mean. It tells us how much, on average, the individual data points deviate from the mean value.

How is the mean calculated?

The mean is calculated by summing the heights (or any other data points) of all individuals and dividing it by the number of individuals.

What does the standard deviation measure in the context of the given example?

In the context of the given example, the standard deviation measures how much the heights of individuals in a group scatter around the mean height.

Why do we calculate the square of the deviations from the mean?

We calculate the square of the deviations to ensure that all values are positive, as negative deviations would otherwise cancel out positive ones when summed.

What is the formula for calculating the standard deviation?

The formula for calculating the standard deviation is the square root of the sum of the squared deviations divided by the number of values (n) or n-1, depending on whether the data represents a population or a sample.

What is the difference between dividing by n and n-1 in the standard deviation formula?

Dividing by n is used when the data represents the entire population, while dividing by n-1 is used for a sample to provide an unbiased estimate of the population standard deviation.

Why is the standard deviation preferred over the variance for describing data?

The standard deviation is preferred because it is in the same unit as the original data, making it easier to interpret. The variance, being the square of the standard deviation, is in the square of the original unit, which is more difficult to interpret.

What is the variance in relation to the standard deviation?

The variance is the squared standard deviation. It represents the average of the squared deviations from the mean.

Why might the average deviation from the mean be different from the standard deviation?

The average deviation from the mean would be different from the standard deviation because the standard deviation uses a quadratic mean (square root of the sum of squared deviations), whereas the average deviation would be the arithmetic mean of absolute deviations.

How does the standard deviation relate to the concept of a normal distribution?

In a normal distribution, the standard deviation describes the spread of the data. Approximately 68% of the data falls within one standard deviation of the mean, and about 95% falls within two standard deviations.

Can you provide an example of how the standard deviation is used in real-life scenarios?

The standard deviation is used in various scenarios such as measuring the variability in test scores, stock price fluctuations, or the range of heights in a population.

Outlines

00:00

📏 Understanding Standard Deviation and Variance

This paragraph introduces the concept of standard deviation as a measure of data dispersion around the mean. It uses the example of measuring the height of a group of people to illustrate how standard deviation quantifies the average deviation from the mean. The process of calculating the mean, determining individual deviations, and then calculating the standard deviation using the formula involving the sum of squared deviations is explained. The paragraph also discusses the difference between using the standard deviation formula for a population (dividing by N) versus a sample (dividing by N-1), highlighting when to use each method.

05:01

🔢 The Relationship Between Standard Deviation and Variance

The second paragraph focuses on the relationship and the difference between standard deviation and variance. It clarifies that while standard deviation is the square root of the average of squared deviations from the mean, variance is the squared standard deviation. The key point is that standard deviation is expressed in the same unit as the original data, making it more intuitive and easier to interpret. Variance, on the other hand, is in the square of the original unit, which can be less intuitive. The summary emphasizes the preference for using standard deviation when describing data due to its interpretability.

Keywords

💡Standard Deviation

Standard deviation is a statistical measure that quantifies the amount of variation or dispersion in a set of values. In the video, it is used to illustrate how much the heights of individuals in a group deviate from the mean height. The script explains that standard deviation is the square root of the variance, indicating the average distance of data points from the mean. It is a key concept in the video as it helps in understanding the spread of the data.

💡Variance

Variance is the average of the squared differences from the Mean. It measures the dispersion of a set of data points. In the context of the video, variance is the squared standard deviation, and it is used to calculate the standard deviation. The script clarifies the relationship between variance and standard deviation, noting that variance is in the square of the original unit, making it less intuitive to interpret compared to standard deviation.

💡Mean

The mean, often referred to as the average, is calculated by summing all the values in a data set and then dividing by the number of values. In the video, the mean height of a group of people is determined to understand how much each individual deviates from this average. The mean serves as a reference point for calculating both the standard deviation and variance.

💡Deviation

Deviation refers to the difference between an individual data point and the mean of the data set. In the script, the deviation of each person's height from the mean height is calculated to determine the standard deviation. It is a fundamental concept in the video, as it shows how each data point varies from the central value.

💡Quadratic Mean

The quadratic mean, also known as the root mean square, is the square root of the average of the squares of the data points. The video explains that the standard deviation is the quadratic mean of the deviations from the mean, emphasizing that it is not the arithmetic mean but a different type of average that accounts for the spread of the data.

💡Population

In statistics, a population refers to the entire set of individuals or items that are being studied. The video mentions that if one had the height data of all Austrian professional soccer players, it would represent the population. The concept of population is important as it determines which formula to use for calculating the standard deviation.

💡Sample

A sample is a subset of a population that is used to represent and analyze the entire population. The video script discusses that in most cases, it is not possible to measure the entire population, so a sample is used instead. The concept of a sample is crucial in the video as it affects the calculation of the standard deviation, with different formulas used depending on whether the data is from a sample or the entire population.

💡Arithmetic Mean

The arithmetic mean is the sum of all data points divided by the number of points, and it is the most common type of average. The video script contrasts the arithmetic mean with the quadratic mean, noting that the standard deviation is calculated using the quadratic mean rather than the arithmetic mean.

💡Units

Units are the measurements used to express the values in a data set. The video script mentions that the standard deviation is in the same unit as the original data, such as centimeters in the example of height, making it easier to interpret. In contrast, the variance is in the square of the original unit, which can be more difficult to understand.

💡Interpretation

Interpretation in statistics refers to the analysis and explanation of data in a meaningful way. The video emphasizes the ease of interpreting standard deviation due to its use of the same units as the original data, as opposed to variance, which requires understanding the concept of squared units.

Highlights

The standard deviation is a measure indicating how much data scatter around the mean.

Calculating the mean involves summing heights and dividing by the number of individuals.

Deviation from the mean is the difference between an individual's height and the mean value.

Standard deviation quantifies the average deviation from the mean.

The equation for standard deviation involves summing square deviations and dividing by the number of values.

The standard deviation is the square root of the sum of squared deviations divided by the number of people.

In the example, the standard deviation of height is calculated to be 11.5 centimeters.

The average deviation from the mean is different from the arithmetic mean used in standard deviation calculation.

There are two equations for standard deviation, one dividing by n for the entire population and another by n-1 for a sample.

When the survey doesn't cover the whole population, the equation with n-1 is used.

The standard deviation and variance are related, with variance being the squared standard deviation.

The standard deviation is always in the same unit as the original data, making it easier to interpret.

The variance is more difficult to interpret due to its unit being the square of the original unit.

It is advisable to use standard deviation to describe data for ease of interpretation.

The video provides a clear explanation of the concepts of standard deviation and variance.

The difference between using n and n-1 in standard deviation calculation is based on whether the data represents a population or a sample.

Transcripts

what is the standard deviation and what

is the difference to the variance the

standard deviation is a measure that

indicates how much data scatter around

the mean here is a simple example let's

say we measure the height of a small

group of people the standard deviation

tells us how much our data scatter

around the mean so first we need to

calculate the mean we can get the mean

simply by summing the heights of all

individuals and dividing it by the

number of individuals let's say we get a

mean value of

155 centimeters now we want to know how

much each person deviates from the mean

so we look at the first person who

deviates 18 centimeters from the mean

value the second person deviates 8

centimeters from the mean value the

third 15 centimeters the fourth eight

centimeters the fifth nine centimeters

and finally the last person deviates 6

centimeters from the mean value simply

said people that are very tall or very

small deviate more from the mean value

but we are not interested in the

deviation of each individual person from

the mean value value we want to know how

much the person's on average deviate

from the mean value so how much do these

persons on average deviate from the mean

value this is what the standard

deviation tells us in our example the

average deviation from the mean value is

11.5 centimeters to calculate the

standard deviation we can use this

equation Sigma is the standard deviation

n is the number of persons x i is the

size of each person and X bar is the

mean value of all persons so the

standard deviation is the root of the

sum of square deviations divided by the

number of values we calculate the size

of the first person minus the mean and

square that the size of the second

person minus the mean and then square

that and so on until we arrive at the

last person then we divide this number

by the number of people so six the

result is a standard deviation of 11.5

centimeters so each individual person

has some deviation from the mean but on

average the people deviate 11.5

centimeters from the mean which is the

standard deviation now you might notice

one thing I always talk about the

average deviation from the mean but for

the average deviation we would actually

just add up all deviation and divided by

the number of participants just like you

calculate a mean value right you're

absolutely right but there are different

mean values in the case of the standard

deviation it is not the arithmetic mean

which is used but a quadratic mean so

far is the good but now there's one more

thing to consider there are two slightly

different equations for the standard

deviation the difference is that in the

first case we divide by n and in the

second case by n minus 1 but why are

there two different equations usually we

want to know the standard deviation of

the population for example we want to

know the standard deviation of the

height of all Austrian professional

soccer players now if we had the height

of really all Austrian professional

soccer players we would take this

equation in with 1 divided by n however

it is usually not possible to serve the

entire population so we draw a sample

then we use the sample to estimate the

standard deviation of the population in

that case you use this equation with n

minus 1. to keep it simple if our survey

doesn't cover the whole population we

always use this equation

likewise if we have conducted a clinical

study then we also use this equation to

infer the population but what is the

difference between the standard

deviation and the variance as we now

know the standard deviation is the

quadratic mean of the distance from the

mean the variance now is the squared

standard deviation so we have one at the

same equation the only difference is

that in order to calculate the standard

deviation we take the root and in order

to calculate the variance we don't

because the root is taken the standard

deviation is always in the same unit as

the original data in our case

centimeters for this reason it is

advisable to always use the standard

deviation to describe data as this makes

interpretation easier the variance is

more difficult to interpret because the

unit is the square of the original unit

in our case centimeters squared thanks

for watching and I hope you enjoyed the

video