8.01x - Lect 3 - Vectors - Dot Products - Cross Products - 3D Kinematics

Summary

In this educational video, Professor Lewin explores the fundamentals of vectors, crucial for understanding physics. He explains scalars, vectors, their addition, and the difference between them. The lecture delves into vector decomposition, the dot and cross products, and their applications in physics. With a practical demonstration involving a moving golf ball, he illustrates the independent behavior of motion in different dimensions, emphasizing the power of vector analysis in simplifying complex physical phenomena.

Takeaways

• 📚 The lecture introduces the concept of scalars and vectors in physics, explaining that scalars like mass, temperature, and speed are described by a single number, while vectors like velocity and acceleration require both magnitude and direction.
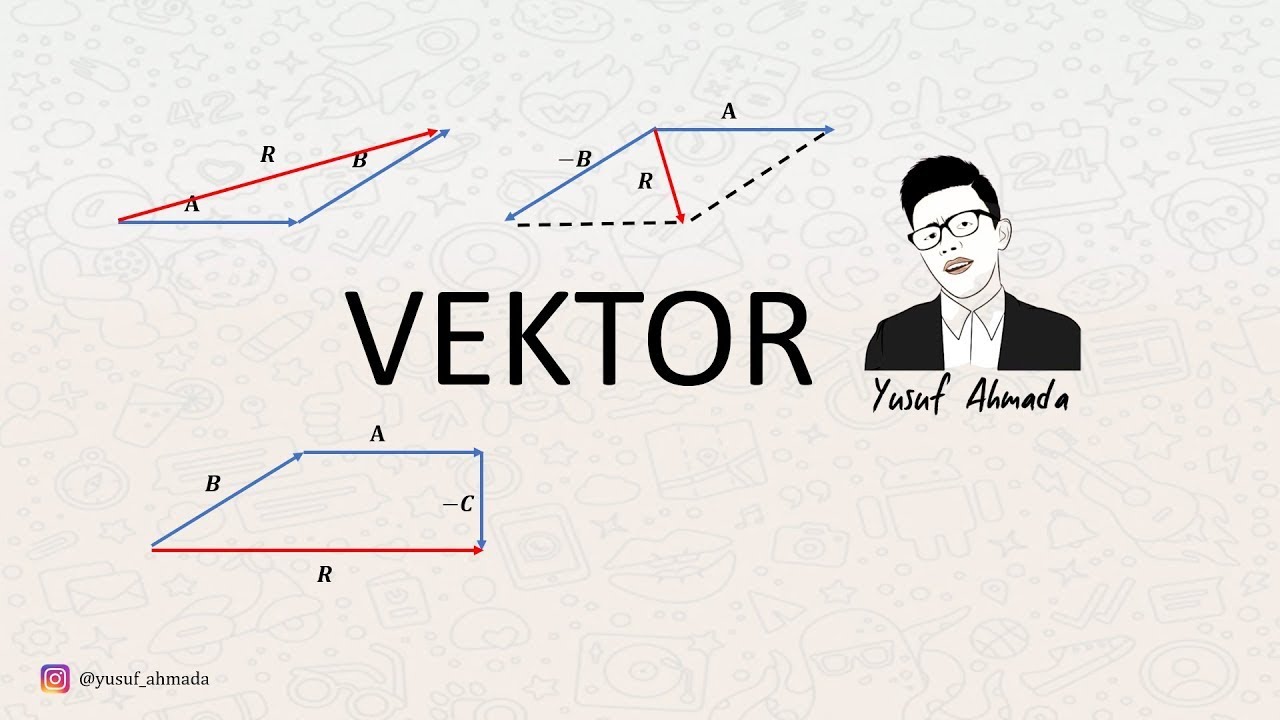
• 📝 Vectors are represented by arrows to indicate direction and can be manipulated using the 'head-tail' technique or the 'parallelogram method' for addition.
• 🔍 The concept of negative vectors is explored, showing that subtracting a vector from itself results in zero, and that a negative vector is the original vector flipped 180 degrees.
• ⚖️ Vector subtraction is explained as being equivalent to adding a negative vector, which can be visualized using both the head-tail technique and the parallelogram technique.
• 📊 The magnitude of a vector is calculated as the square root of the sum of the squares of its components, and the angles with the axes (theta and phi) are used to decompose a vector into its components.
• 📐 Unit vectors are introduced as vectors with a magnitude of one that point in the direction of the positive axes, and are used to express vectors in terms of their components along the axes.
• ✖️ The dot product of two vectors is defined as a scalar resulting from the sum of the products of their corresponding components or as the product of the magnitudes and the cosine of the angle between them.
• ✂️ The cross product, or vector product, is introduced as a method to multiply vectors resulting in another vector perpendicular to the plane containing the original vectors, with direction determined by the right-hand rule.
• 🔄 The motion of a particle in three-dimensional space is described by decomposing its position, velocity, and acceleration into components along the x, y, and z axes, allowing for the analysis of complex motion as a combination of independent one-dimensional motions.
• 🚀 The independence of motion in the x and y directions is demonstrated through projectile motion, where the horizontal velocity remains constant while the vertical motion is influenced by gravity.
• 🎯 An experiment is proposed to launch a golf ball with a horizontal velocity matching that of a moving cart to illustrate the principle that the horizontal motion is independent of the vertical motion influenced by gravity.

Q & A

What is the main topic discussed in the provided script?

The main topic discussed in the script is the concept of vectors in physics, including their addition, subtraction, and multiplication (dot and cross products), as well as their applications in motion and forces.

What is a scalar in physics?

A scalar in physics is a quantity that is determined by a single number and has no direction, such as mass, temperature, and speed.

How is a vector represented?

A vector is represented by an arrow, where the length of the arrow indicates the magnitude and the direction of the arrow indicates the direction of the vector.

What are the two common methods for adding vectors?

The two common methods for adding vectors are the 'head-tail' technique and the 'parallelogram method'.

What is the significance of the negative vector in the context of vector subtraction?

The negative vector is significant in vector subtraction because it represents the vector that, when added to a given vector, results in a zero vector. It is essentially the original vector flipped over 180 degrees.

What is the dot product of two vectors?

The dot product of two vectors is a scalar value obtained by multiplying corresponding components of the vectors and summing the results. It can also be represented as the product of the magnitudes of the two vectors and the cosine of the angle between them.

What is the cross product of two vectors?

The cross product of two vectors results in a vector that is perpendicular to both of the original vectors. It is calculated using a determinant involving the unit vectors and components of the original vectors.

How does the magnitude of a vector relate to its components?

The magnitude of a vector is the square root of the sum of the squares of its components along each axis (Ax^2 + Ay^2 + Az^2)^(1/2).

What is the physical interpretation of the dot product being zero?

A dot product of zero indicates that the two vectors are perpendicular to each other, as the cosine of the angle between them (90 degrees) is zero.

How can the motion of a particle in three-dimensional space be decomposed?

The motion of a particle in three-dimensional space can be decomposed into three independent one-dimensional motions along the x, y, and z axes, each with its own position, velocity, and acceleration components.

What is the practical demonstration mentioned in the script to illustrate the independent behavior of motion in the x and y directions?

The practical demonstration involves shooting a golf ball with a horizontal velocity that matches the motion of a moving cart, showing that the ball maintains a constant horizontal velocity while the cart moves underneath it.

Outlines

00:00

📚 Introduction to Scalars and Vectors

The script begins by introducing the concept of scalars and vectors in physics. Scalars are quantities defined by a single number, such as mass, temperature, and speed. Vectors, on the other hand, require both magnitude and direction, such as velocity and acceleration. The script explains that vectors are represented by arrows, and their addition can be visualized using the 'head-tail' technique or the 'parallelogram method'. The importance of understanding vectors is emphasized as it is fundamental to the study of physics.

05:03

🔍 Vector Addition and Scalar Multiplication

This paragraph delves deeper into vector operations, specifically the addition and subtraction of vectors. It clarifies that the sum of vectors can vary greatly depending on their direction relative to each other, contrasting this with the straightforward nature of scalar addition. The concept of vector decomposition is introduced, where a single vector in three-dimensional space can be broken down into its x, y, and z components. The paragraph also introduces unit vectors and their role in representing the components of a vector in a given direction.

10:06

📐 Understanding Vector Projections and Magnitudes

The script discusses the process of projecting a vector onto the axes in a three-dimensional space and using these projections to decompose a vector into its components. It explains how to calculate the magnitude of a vector by taking the square root of the sum of the squares of its components. The paragraph also covers how to determine the angles associated with a vector in three-dimensional space and how these angles relate to the vector's components.

15:08

🤔 Exploring Vector Multiplication

The script introduces the concept of vector multiplication, which is more complex than addition or subtraction. Two types of multiplication are discussed: the dot product (or scalar product) and the cross product. The dot product is a scalar value obtained by multiplying corresponding components of two vectors and summing the results. The paragraph also presents an alternative method for calculating the dot product using the magnitudes of the vectors and the cosine of the angle between them.

20:10

🔄 The Dot Product and Its Implications

This paragraph expands on the dot product, explaining its geometric interpretation as the product of the magnitudes of two vectors and the cosine of the angle between them. It discusses the implications of the dot product for determining the angle between vectors and its role in physics, such as in calculating work done by a force. The script provides examples to illustrate how the dot product is calculated in different scenarios.

25:11

🌐 The Cross Product and Its Geometric Interpretation

The script introduces the cross product, a vector multiplication that results in a vector perpendicular to the plane containing the two original vectors. It presents a method for calculating the cross product using a determinant with unit vectors and components of the original vectors. The paragraph also offers a geometric interpretation of the cross product, relating it to the sine of the angle between the vectors and emphasizing the importance of using a right-handed coordinate system.

30:17

🎯 Direction of the Cross Product and Right-Handed System

This paragraph focuses on determining the direction of the cross product, explaining the right-hand rule for finding the direction of the resulting vector. It emphasizes the importance of using a right-handed coordinate system and the consequences of not doing so. The script uses the analogy of a corkscrew to help visualize the direction of the cross product and discusses the relationship between the cross product and physical concepts such as torque and angular momentum.

35:18

🚀 Equations of Motion for a Particle in 3D Space

The script presents the equations of motion for a particle moving in three-dimensional space. It breaks down the position, velocity, and acceleration of the particle into their x, y, and z components, demonstrating how to express these quantities as functions of time. The paragraph shows how to decompose a complex three-dimensional motion into simpler, independent one-dimensional motions along each axis.

40:19

🏐 Projectile Motion Decomposition

This paragraph applies the concept of motion decomposition to the example of projectile motion, such as throwing a ball at an angle. It explains how to calculate the initial velocity components in the x and y directions and how these components evolve over time. The script highlights that the horizontal motion (x-direction) maintains a constant velocity, while the vertical motion (y-direction) is affected by gravity, resulting in a parabolic trajectory.

45:23

🎾 Demonstrating Independent Horizontal Motion

The script concludes with a demonstration of the independent horizontal motion in projectile motion. It describes an experiment where a golf ball is shot with a horizontal velocity that matches the velocity of a moving cart, illustrating that the ball can be caught by the cart if the initial conditions are met. The paragraph emphasizes the superposition of the horizontal and vertical motions to describe the actual trajectory of the projectile.

Keywords

💡Scalars

Scalars are quantities in physics that are defined by a single numerical value and have no direction, such as mass, temperature, and speed. In the video, the concept of scalars is introduced to contrast with vectors, which have both magnitude and direction. Scalars are fundamental in understanding the basic properties of physical systems.

💡Vectors

Vectors are quantities that have both magnitude and direction, making them essential for describing phenomena like velocity and acceleration in physics. The video emphasizes the importance of vectors in representing motion and introduces the concept of vector addition and subtraction, which is central to understanding motion in multiple dimensions.

💡Head-Tail Technique

The head-tail technique is a method for adding vectors by aligning the tail of one vector with the head of another and then drawing the resultant vector from the original tail to the new head. This technique is highlighted in the video as a simple and effective way to visualize and perform vector addition.

💡Parallelogram Method

The parallelogram method is another approach to vector addition, where the tails of two vectors are placed together, and a parallelogram is completed by drawing the opposite side from the last vector's head. The script uses this method to demonstrate an alternative way to add vectors, emphasizing that the order of addition does not affect the result.

💡Negative Vector

A negative vector is a vector that has the same magnitude but opposite direction to another vector. In the video, the concept of negative vectors is introduced to explain vector subtraction, where subtracting a vector is equivalent to adding its negative counterpart.

💡Decomposition

Decomposition in the context of vectors refers to the process of breaking down a vector into components along different axes. The video discusses decomposing a three-dimensional vector into its x, y, and z components, which is crucial for analyzing motion in 3D space.

💡Unit Vectors

Unit vectors are vectors with a magnitude of one, pointing in the direction of a coordinate axis. The script introduces unit vectors as a way to express vectors in terms of their components along the axes, which simplifies calculations and understanding of vector operations.

💡Dot Product

The dot product, also known as the scalar product, is a mathematical operation that takes two vectors and returns a scalar value. The video explains the dot product as a means to find the projection of one vector onto another and introduces two methods for calculating it, which is important for understanding the relationship between vectors.

💡Cross Product

The cross product is a binary operation on two vectors in three-dimensional space, resulting in a vector that is perpendicular to the plane containing the two original vectors. The script describes the cross product and its geometric interpretation, which is essential for understanding vector relations in three dimensions.

💡Right-Handed Coordinate System

A right-handed coordinate system is a convention in which the x, y, and z axes are oriented in a way that the cross product of the unit vectors i (x) and j (y) equals k (z). The video emphasizes the importance of using a consistent coordinate system when working with cross products to avoid confusion and errors.

💡Projectile Motion

Projectile motion is the motion of an object thrown or projected into the air, subject to only the acceleration of gravity. The video uses projectile motion as an example to demonstrate the decomposition of a three-dimensional motion into two independent one-dimensional motions, along the x and y axes, which simplifies the analysis of the object's trajectory.

Highlights

Introduction to the concept of scalars and vectors in physics, emphasizing the unique determination by a single number for scalars and the need for both magnitude and direction for vectors.

Explanation of vector representation through arrows, illustrating direction and magnitude.

Demonstration of vector addition using the 'head-tail' technique and the 'parallelogram method'.

Clarification on the commutative property of vector addition, showing A + B is equivalent to B + A.

Introduction to the concept of negative vectors and their role in vector subtraction.

Illustration of vector subtraction using both head-tail and parallelogram techniques.

Discussion on the range of possible magnitudes for the sum of two vectors based on their direction.

Introduction to vector decomposition, a key concept for advanced physics applications.

Projection of a three-dimensional vector onto the x, y, and z axes, and the introduction of unit vectors.

Expression of a vector in terms of its components along the axes using unit vectors.

Calculation of a vector's magnitude from its components.

Introduction to the dot product, a method of multiplying vectors resulting in a scalar.

Geometric interpretation of the dot product using the angle between two vectors.

Introduction to the cross product, a vector multiplication resulting in a vector perpendicular to both original vectors.

Geometric interpretation of the cross product direction using the right-hand rule.

Equations for the motion of a particle in three-dimensional space, decomposing motion into components along each axis.

Demonstration of constant horizontal velocity in projectile motion due to the independence of x and y motion.

Experimental demonstration of the independence of horizontal and vertical motion using a moving cart and a projectile.

Transcripts

The bad news today is that there will be quite a bit of math.

But the good news is that we will only do it once

and it will only take something like half-hour.

There are quantities in physics

which are determined uniquely by one number.

Mass is one of them.

Temperature is one of them.

Speed is one of them.

We call those scalars.

There are others where you need more than one number

for instance, on a one- dimensional motion, velocity

it has a certain magnitude-- that's the speed--

but you also have to know

whether it goes this way or that way.

So there has to be a direction.

Velocity is a vector and acceleration is a vector

and today we're going to learn how to work with these vectors.

A vector has a length and a vector has a direction

and that's why we actually represent it by an arrow.

We all have seen... this is a vector.

Remember this-- this is a vector.

If you look at the vector head-on, you see a dot.

If you look at the vector from behind, you see a cross.

This is a vector

and that will be our representation of vectors.

Imagine that I am standing on the table in 26.100.

This is the table and I am standing, say, at point O

and I move along a straight line from O to point P

so I move like so.

That's why I am on the table

and that's where you will see me when you look from 26.100.

It just so happens

that someone is also going to move the table--

in that same amount of time-- from here to there.

So that means that the table will have moved down

and so my point P will have moved down exactly the same way

and so you will see me now at point S.

You will see me at point S in 26.100

although I am still standing

at the same location on the table.

The table has moved.

This is now the position of the table.

See, the whole table has shifted.

Now, if these two motions take place simultaneously

then what you will see from where you are sitting...

you will see me move in 26.100 from O straight line to S

and this holds the secret behind the adding of vectors.

We say here that the vector OS-- we'll put an arrow over it--

is the vector OP, with an arrow over it, plus PS.

This defines how we add vectors.

There are various ways that you can add vectors.

Suppose I have here vector A and I have here vector B.

Then you can do it this way

which I call the "head-tail" technique.

I take B and I bring it to the head of A.

So this is B, this is a vector

and then the net result is A plus B.

This vector C equals A plus B.

That's one way of doing it.

It doesn't matter whether you take B...

the tail of B to the head of A

or whether you take the tail of A and bring it to the head of B.

You will get the same result.

There's another way you can do it

and I call that "the parallelogram method."

Here you have A.

You bring the two tails together, so here is B now

so the tails are touching

and now you complete this parallelogram.

And now this vector C is the same sum vector

that you have here, whichever way you prefer.

You see immediately

that A plus B is the same as B plus A.

There is no difference.

What is the meaning of a negative vector?

Well, A minus A equals zero--

vector A subtract from vector A equals zero.

So here is vector A.

So which vector do I have to add to get zero?

I have to add minus A.

Well, if you use the head-tail technique...

This is A.

You have to add this vector to have zero

so this is minus A

and so minus A is nothing but the same as A

but flipped over 180 degrees.

We'll use that very often.

And that brings us to the point of subtraction of vectors.

How do we subtract vectors?

So A minus B equals C.

Here we have vector A and here we have--

let me write this down here-- and here we have vector B.

One way to look at this is the following.

You can say A minus B is A plus minus B

and we know how to add vectors and we know what minus B is.

Minus B is the same vector but flipped over

so we put here minus B

and so this vector now here equals A minus B.

Here's vector C, here's A minus B.

And, of course, you can do it in different ways.

You can also think of it as A plus... as C plus B is A.

Right? You can say you can bring this to the other side.

You can say C plus B is A, C plus B is A.

In other words, which vector do I have to add to B to get A?

And then you have the parallelogram technique again.

There are many ways you can do it.

The head-tail technique

is perhaps the easiest and the safest.

So you can add a countless number of vectors

one plus the other, and the next one

and you finally have the sum of five or six or seven vectors

which, then, can be represented by only one.

When you add scalars, for instance, five and four

then there is only one answer, that is nine.

Five plus four is nine.

Suppose you have two vectors.

You have no information on their direction

but you do know that the magnitude of one is four

and the magnitude of the other is five.

That's all you know.

Then the magnitude of the sum vector could be nine

if they are both in the same direction-- that's the maximum--

or it could be one, if they are in opposite directions.

So then you have a whole range of possibilities

because you do not know the direction.

So the adding and the subtraction of vectors

is way more complicated than just scalars.

As we have seen, that the sum of vectors

can be represented by one vector

equally can we take one vector

and we can replace it by the sum of others.

And we call that "decomposition" of a vector.

And that's going to be very important in 801

and I want you to follow this, therefore, quite closely.

I have a vector which is in three-dimensional space.

This is my z axis...

this is my x axis, y axis and z axis.

This is the origin O and here is a point P

and I have a vector OP-- that's the vector.

And what I do now, I project this vector

onto the three axes, x, y and z.

So there we go.

Each one has her or his own method of doing this.

There we are.

I call this vector vector A.

Now, this angle will be theta, and this angle will be phi.

Notice that the projection of A on the y axis has here

a number which I call A of y.

This number is A of x and this number here is A of z--

simply a projection of that vector onto the three axes.

We now introduce what we call "unit vectors."

Unit vectors are always pointing in the direction

of the positive axis

and the unit vector in the x direction is this one.

It has a length one, and we write for it "x roof."

"Roof" always means unit vector.

And this is the unit vector in the y direction

and this is the unit vector in the z direction.

And now I'm going to rewrite vector A

in terms of the three components that we have here.

So the vector A, I'm going to write

as "A of x times x roof, plus A of y times y roof

plus A of z times z roof."

And this A of x times x is really a vector

that runs from the origin to this point.

So we could put in that as a vector, if you want to.

This makes it a vector.

This is that vector.

A of y times... oh, sorry, it is A of x, this one.

A of y times y roof is this one

and A of z times z roof is this one.

And so these three green vectors added together

are exactly identical to the vector OP

so we have decomposed one vector into three directions.

And we will see that very often, this is of great use in 801.

The magnitude of the vector is

the square root of Ax squared plus Ay squared plus Az squared

and so we can take a simple example.

For instance, I take a vector A--

this is just an example, to see this in action--

and we call A three X roof,

so A of axis is three minus five y roof plus 6 Z roof

so that means that it's three units in this direction

it is five units in this direction--

in the minus y direction--

and six in the plus z direction.

That makes up a vector and I call that vector A.

What is the magnitude of that vector--

which I always write down with vertical bars--

if I put two bars on one side, that's always the magnitude

or sometimes I simply leave the arrow off,

but to be always on the safe side, I like this idea

that you know it's really the magnitude

becomes the scalar when you do that.

So that would be the square root of three squared is nine

five squared is 25, six squared is 36

so that's the square root of 70.

And suppose I asked you, "What is theta?"

It's uniquely determined, of course.

This vector is uniquely determined

in three-dimensional space

so you should be able to find phi and theta.

Well, the cosine of theta...

See, this angle here... 90 degrees projection.

So the cosine of theta is A of z divided by A itself.

So the cosine of theta equals A of z divided by A itself

which in our case

would be six divided by the square root of 70.

And you can do fine.

It's just simply a matter of manipulating some numbers.

We now come to a much more difficult part of vectors

and that is multiplication of vectors.

We're not going to need this until October, but I decided

we might as well get it over with now.

Now that we introduced vectors, you can add and subtract

you might as well learn about multiplication.

It's sort of, the job is done, it's like going to the dentist.

It's a little painful, but it's good for you

and when it's behind you, the pain disappears.

So we're going to talk about multiplication of vectors

something that will not come back until October

and later in the course.

There are two ways that we multiply vectors

and one is called the "dot product"

often also called the scalar product.

A dot B, a fat dot, and that is defined as it is a scalar.

A of x times B of x, just a number

plus A of y times B of y-- that's another number--

plus A of z times B of z-- that's another number.

It is a scalar.

It has no longer a direction.

That is the dot product.

So that's method number one.

That's completely legitimate and you can always use that.

There is another way to find the dot product

depending upon what you're being given--

how the problem is presented to you.

If someone gives you the vector A and you have the vector B

and you happen to know this angle between them,

this angle theta--

which has nothing to do with that angle theta;

it's the angle between the two--

then the dot product is also the following

and you may make an attempt to prove that.

You project the vector B on A.

This is that projection.

The length of this vector is B cosine theta.

And then the dot product

is the magnitude of A times the magnitude of B

times the cosine of the angle theta.

The two are completely identical.

Now, you may ask me, you may say,

"Gee, how do I know what theta is?

"How do I know I should take theta this angle

"or maybe I should take theta this angle?

I mean, what angle is A making with B?"

It makes no difference

because the cosine of this angle here

is the same as the cosine of 360 degrees minus theta

so that makes no difference.

Sometimes this is faster

depending upon how the problem is presented to you;

sometimes the other is faster.

You can immediately see by looking at this--

it's easier to see than looking here--

that the dot product can be larger than zero

it can be equal to zero and it can be smaller than zero.

A and B are, by definition, always positive.

They are a magnitude.

That's always determined by the cosine of theta.

If the cosine of theta is larger than zero,

well, then it's larger than zero.

The cosine of theta can be zero.

If the angle for theta is pi over two--

in other words, if the two vectors

are perpendicular to each other--

then the dot product is zero, and if this angle theta

is between 90 degrees and 180 degrees

then the cosine is negative.

We will see that at work, no pun implied

when we're going to deal with work in physics.

You will see that we can do positive work

and we can do negative work

and that has to do with this dot product.

Work is a dot product.

I could do an extremely simple example with you;

the simplest that I can think of.

Perhaps it's almost an insult-- it's not meant that way.

Suppose we have A dot B

and A is the one that you really have on the blackboard there.

Right here, that's A.

But B is just two y roof.

Two y roof, that's all it is.

Well, what is A dot B?

A dot B... there's no x component of B

so that becomes zero, this term becomes zero.

There is only a y component of B

so it is minus five times plus two

so I get minus ten, because there was no z component.

Simple as that, so it's minus ten.

I can give you another example, example two.

Suppose A itself is the unit vector in the y direction

and B is the unit vector in the z direction.

Then A dot B is what?

I want to hear it loud and clear.

CLASS: Zero.

LEWIN: Yeah! Zero.

It is zero-- you don't even have to think about anything.

You know that these two are at 90 degrees.

If you want to waste your time

and want to substitute it in here

you will see that it comes out to be zero.

It should work, because clearly A of y means

that this... this is one.

That's what it means.

And B is z, that means that B of z... this is one

and all the others do not exist.

Well, I wish you luck with that and we now go

to a way more difficult part of multiplication

and that is vector multiplication

which is called "the vector product."

Or also called... most of the time

I refer to it as "the cross product."

The cross product is written like so: A cross B equals C.

It's a cross, very clear cross.

And I will tell you how I remember...

that is, method number one.

I'm going to teach you-- just like with the dot product--

two methods.

I will tell you method number one

which is the one that always works.

It's time-consuming, but it always works.

You write down here a matrix with three rows.

The first row is x roof, y roof, z roof.

The second one is A of x, A of y, A of z.

It's important, if A is here first

that that second row must be A and the third row is then B.

B of x, B of y, B of z.

So these six are numbers and these are the unit vectors.

I repeat this here verbatim--

you will see in a minute why I need that--

and I will do the same here.

Okay, and now comes the recipe.

You take... you go from the upper left-hand corner

to the one in this direction.

You multiply them, all three, and that's a plus sign.

So you get Ay... so C

which is A cross B equals Ay, times Bz, times the x roof--

but I'm not going to put the x roof in yet--

because I have to subtract this one... minus sign

which has Az By

so it is minus Az By, and that is in the direction x.

The next one is this one.

Az Bx...

minus this one

Ax Bz

in the direction y.

And last but not least

Ax By...

minus Ay Bx...

in the direction of the unit vector z.

So this part here is what we call "C of x".

It's the x component of this vector

and this we can call "C of y" and this we can call "C of z."

So we can also write that vector, then,

that C equals C of x, x roof, plus C of y, y roof

plus C of z, z roof.

Cross product of A and B.

We will have lots of exercises,

lots of chances you will have on assignment, too

to play with this a little bit.

Now comes my method number two and method number two is, again

as we had with the dot product, is a geometrical method.

Let me try to work on this board in between.

If you know vector A and you know vector B

and you know that the angle is theta

then the cross product, C, equals A cross B

is the magnitude of A times the magnitude of B

times the sine of theta

not the cosine of theta as we had before with the dot product.

It is the sine of theta.

So you can really immediately see that this will be zero

if theta is either zero degrees or 180 degrees

whereas the dot product was zero

when the angle between them was 90 degrees.

This number can be larger than zero

if the sine theta is larger than zero.

It can also be smaller than zero.

Now we only have the magnitude of the vector

and now comes the hardest part.

What is the direction of the vector?

And that is something

that you have to engrave in your mind and not forget.

The direction is found as follows.

You take A, because it's first mentioned

and you rotate A over the shortest possible angle to B.

If you had in your hand a corkscrew--

and I will show that in a minute--

then you turn the corkscrew as seen from your seats clockwise

and the corkscrew would go into the blackboard.

And if the corkscrew goes into the blackboard

you will see the tail of the vector

and you will see a cross, little plus sign

and therefore we put that like so.

A cross product is always perpendicular to both A and B

but it leaves you with two choices:

It can either come out of the blackboard

or it can go in the blackboard

and I just told you which convention to use.

And I want to show that to you

in a way that may appeal to you more.

This is what I have used before

on my...

television help sessions that I have given at MIT.

I have an apple-- not an apple...

This is a tomato-- not a tomato...

It's a potato.

(class laughs)

I have a potato here and here is a corkscrew.

There is a corkscrew.

I'm going to turn the corkscrew

as seen from your side, clockwise.

And you'll see that the corkscrew goes into the potato

in -- that's the direction, then, of the vector.

If we had B cross A, then you take B in your hands

and you rotate it over the shortest angle to A.

Now you have to rotate counterclockwise

and when you rotate counterclockwise

the corkscrew comes to you-- there you go--

and so the vector is now pointing in this direction.

And if the vector is pointing towards you

then we would indicate that with a circle and a dot.

In other words, for this vector

B cross A would have exactly the same magnitude--

no difference-- but it would be coming out of the blackboard.

In other words, A cross B equals minus B cross A

whereas A dot B is the same as B dot A.

We will encounter cross products when we deal with torques

and when we deal with angular momentum

which is not the easiest part of 801.

Let's take an extremely simple example.

Again, I don't mean to insult you with such a simple example

but you will get chances,

more advanced chances on your assignment.

Suppose I have the vector A is x roof.

It's a unit vector in the x direction.

That means A of x is one

and A of y is zero and A of z is zero.

And suppose B is y roof.

That means B of y is one

and B of x is zero and B of z is zero.

What, now, is the dot product, the cross product, A cross B?

Well, you can apply that recipe

but it's much easier to go

to the x, y, z axes that we have here.

A was in the x direction, the unit vector

and B in the y direction.

I take A in my hand, I rotate over the smallest angle

which is 90 degrees to y, and my corkscrew will go up.

So I know the whole thing already.

I know that this cross product must be z roof.

The magnitude must be one.

That's immediately clear.

But I immediately have the direction

by using the corkscrew rule.

Now if you're very smart

you may say, "Aha! You find plus z

"only because you have used this coordinate system.

"If this axis had been x, and this one had been y

"then the cross product of x and y would be

in the minus z direction."

Yeah, you're right.

But if you ever do that, I WILL KILL YOU!

(class laughs)

You will always, always have to work

with what we call "a right- handed coordinate system."

And a right-handed coordinate system, by definition

is one whereby the cross product

of x with y is z and not y minus z.

So whenever you get, in the future, involved

with cross products and torques and angular momentum

always make yourself an xyz diagram

for which x cross y is z.

Never, ever make it such that x cross y is minus z.

You're going to hang yourself.

Because for one thing, that wouldn't work anymore.

So be very, very careful.

You must work... if you use the right-hand corkscrew rule

make sure you work with the right-handed coordinate system.

All right, now the worst part is over.

And now I would like to write down for you...

We pick up some of the fruits now

although it will penetrate slowly.

I want to write down for you equations for a moving particle

a moving object in three-dimensional space--

a very complicated motion

which I can hardly imagine what it's like.

It is a point that is going to move around in space

and it is this point P

this point P is going to move around in space

and I call this vector OP, I call that now vector r

and I give it a sub-index t

which indicates it's changing with time.

I call this location A of y, I am going to call that y of t.

It's changing with time.

I call this x of t-- it's going to change with time--

and I call this point z of t

which is going to change with time

because point P is going to move.

And so I'm going to write down the vector r

in its most general form that I can do that.

R, which changes with time

is now x of t-- which is the same as a over x there, before--

times x roof plus y of t,

y roof plus z of t, z roof.

I have decomposed my vector r into three independent vectors.

Each one of those change with time.

What is the velocity of this particle?

Well, the velocity is the first derivative of the position

so that it is dr dt.

So there we go-- first the derivative of this one

which is dx dt, x roof.

I am going to write for dx dt "x dot," because I am lazy

and I am going to write for d2x dt squared, "x double dots."

It's often done, but not in your book.

But it is a notation that I will often use

because otherwise the equations look so clumsy.

Plus y dot times y roof plus z dot times z roof.

So z dot is the dz/dt.

What is the acceleration as a function of time?

Well, the acceleration as a function of time

equals dv/dt.

So that's the second derivative of x versus time

and so that becomes x double dot times x roof

plus y double dot times y roof plus z double dot times z roof.

And look what we have now accomplished.

It looks like minor, but it's going to be big later on.

We have a point P going in three-dimensional space

and here we have the entire behavior of the object

as it moves its projection along the x axis.

This is the position, this is its velocity

and this is its acceleration.

And here you can see the entire behavior on the z axis.

This is the position on the z axis

this is the velocity component in the z direction

and this is the acceleration on the z axis.

And here you have the y.

In other words, we have now... the three-dimensional motion

we have cut into three one-dimensional motions.

This is a one-dimensional motion.

This is behavior only along the x axis

and this is a behavior only along the y axis

and this is a behavior only along the z axis

and the three together make up

the actual motion of that particle.

What have we gained now?

It looks like... this looks like a mathematical zoo.

You would say, "Well, if this is what it is going to be like

it's going to be hell."

Well, not quite--

in fact, it's going to help you a great deal.

First of all, if I throw up a tennis ball in class

like this, then the whole trajectory is...

the whole trajectory is in one plane

in the vertical plane.

So even though it is in three dimensions

we can always represent it by two axes, by two dimensionally

a y axis and an x axis

so already the three-dimensional problem

often becomes a two-dimensional problem.

We will, with great success, analyze these trajectories

by decomposing this very complicated motion.

Imagine what an incredibly complicated arc that is

and yet we are going to decompose it

into a motion in the x direction

which lives a life of its own

independent of the motion in the y direction

which lives a life of its own

and, of course, you always have to combine the two

to know what the particle is doing.

We know the equations so well from our last lecture

from one-dimensional motion with constant acceleration.

The first line tells you

what the x position is as a function of time.

The index t tells you that it is changing with time.

It is the position at t equals zero

plus the velocity at t equals zero

times t plus one-half ax t squared

if there is an acceleration in the x direction.

The velocity immediately comes

from taking the derivative of this function

and the acceleration comes

from taking the derivative of this function.

Now, if we have a motion which is more complicated--

which reaches out to two or three dimensions--

we can decompose the motion in three perpendicular axes

and you can replace every x here by a y

which gives you the entire behavior in the y direction

and if you want to know the behavior in the z direction

you replace every x here by z

and then you have decomposed the motion in three directions.

Each of them are linear.

And that's what I want to do now.

I'm going to throw up an object, golf ball or an apple in 26.100

and we know that it's in the vertical plane, so we have...

we only deal with a two-dimensional problem

this being...

I call this my x axis and I'm going to call this my y axis.

I call this increasing value of x

and I call this increasing value of y.

I could have called this increasing value of y.

Today I have decided to call this increasing value of y.

I am free in that choice.

I throw up an object at a certain angle

and I see a motion like this-- boing!--

and it comes back to the ground.

My initial speed when I threw it was v zero

and the angle here is alpha.

The x component of that initial velocity

is v zero cosine alpha

and the y component equals v zero sine alpha.

So that's the "begin"-velocity of the x direction.

And this is the "begin"-velocity in the y direction.

A little later in time, that object is here at point P

and this is now the position vector

which we have called r of t, it's this vector.

That's the vector that is moving through space.

At this moment in time, x of t is here

and at this moment in time, y of t is here.

And now you're going to see, for the first time

the big gain by the way that we have divided the two axes

which live an independent life.

First x.

I want to know everything about x that there has to be known.

I want to know where it is at any moment in time

velocity and the acceleration, only in x.

First I want to know that at t = 0.

Well, at t = 0, I look there

X zero-- that's the, I can choose that to be zero.

So I can say x zero is zero, that's my free choice.

Now I need v zero x-- what is the velocity?

The velocity at t = 0, which we have called v zero x

is this velocity-- v zero cosine alpha.

And it's not going to change.

Why is it not going to change?

Because there is no a of x, so this term here is zero

we only have this one.

So at all moments in time

the velocity in the x direction is v zero cosine alpha

and the a of x equals zero.

Now I want to do the same in the x direction for time t.

Well, at time t, I look there at the first equation.

There it is-- x zero is zero.

I know v zero x, that is v zero cosine alpha

so x of t is v zero cosine alpha times t

but there is no acceleration, so that's it.

What is vx of t?

The velocity in the x direction at any moment in time.

That is that equation, that is simply v zero x.

It is not changing in time

because there is no acceleration.

So the initial velocity at t zero is the same

as t seconds later and the acceleration is zero.

Now we're going to do this for the y direction.

And now you begin to see the gain for the decomposition.

In the y direction, we change the x by y

and so we do it first at t = 0.

So look there.

This becomes y zero-- I call that zero.

I can always call my origin zero.

I get v zero y times t.

Well, v zero y is this quantity

is v zero sine alpha, v zero sine alpha.

This is v zero sine alpha.

That is the velocity at time zero, and this is zero.

At time zero... this is zero at time zero.

What is the acceleration in the y direction at time zero?

What is the acceleration? That has to do with gravity.

There is no acceleration in the x direction

but you better believe that there is one in the y direction.

So only when we deal with the y equations

does this acceleration come in--

not at all when we deal with the x direction.

Well, if we call the acceleration due to gravity

g equals plus 9.80, and I always call it g

what would be the acceleration in the y direction

given the fact that I call this increasing value of y?

CLASS: Minus 9.8.

LEWIN: Minus 9.8, which I will also say

always call minus g because my g is always positive.

So it is minus g.

So that tells the story of t equals zero in the y direction

and now we have to complete it at time t equals t.

At time t equals t, we have the first line there.

Y zero is zero.

So we have y as a function of time, y zero is zero

so we don't have to work with that.

Where is my... so this is zero, so I get v zero y times t

so I get v zero sine alpha times t

plus one-half, but it is minus one-half g t squared

and now I get the velocity in the y direction at time t--

that is my second line.

That is going to be v zero sine alpha minus g t

and the acceleration in the y direction at any moment in time

equals minus g.

And now I have done all I can

to completely decompose this complicated motion

into two entirely independent one-dimensional motions.

And the next lecture

we're going to use this again and again and again and again.

This lecture is not over yet but I want you to know

that this is what we're going to apply

for many lectures to come--

the decomposition of a complicated trajectory

into two simple ones.

Now, when you look at this

there is something quite remarkable

and the remarkable thing is

that the velocity in the x direction

throughout this whole trajectory--

if there is no air draft, if there is no friction--

is not changing.

It's only the velocity in the y direction that is changing.

It means if I throw up this golf ball--

I throw it up like this--

and it has a certain component in x direction

a certain velocity

if I move myself with exactly that same velocity--

with exactly the same horizontal velocity--

I could catch the ball here.

It would have to come back exactly in my hands.

That is because there is only an acceleration in the y direction

but the motion in the y direction

is completely independent of the x direction.

The x direction doesn't even know

what's going on with the y direction.

In the x direction, if I throw an object like this

the x direction simply, very boringly,

moves with a constant velocity.

There is no time dependence.

And the y direction, on its own, does its own thing.

It goes up, comes to a halt and it stops.

And, of course, the actual motion is the sum

the superposition of the two.

We have tried to find a way

to demonstrate this quite bizarre behavior

which is not so intuitive.

That the x direction really lives a life of its own.

And the way we want to do that is as follows.

We have here a golf ball, a...

a gun we can shoot up the golf ball

and we do that in such a way

that the golf ball, if we do it correctly

exactly comes back here.

That's not easy-- that takes hours and hours of adjustments.

The golf ball goes up and comes back here.

Not here, not here, not there-- that's easy.

You can shoot it up a little at an angle

and the golf ball will come back here.

Once we have achieved that--

that the golf ball will come back there--

then I'm going to give this cart a push

and the moment that it passes through this switch

the golf ball will fire

so that the golf ball will go straight up

as seen from the cart

but it has a horizontal velocity

which is exactly the same horizontal velocity as the cart

so the cart are like my hands.

As the golf ball goes like this

the cart stays always exactly under the golf ball

always exactly under the golf ball

and if all works well

the ball ends up exactly on the cart again.

Let me first show you--

otherwise, if that doesn't work, of course, it's all over--

that if we shoot the ball straight up

that it comes back here.

If it doesn't do that

I don't even have to try this more complicated experiment.

So here's the golf ball.

I'm going to fire the gun now.

Close... close.

Reasonably close.

Well, since it's only reasonably close, perhaps...

(class laughs)

Perhaps it would help if we give it a little bit of leeway.

There goes the gun.

Here comes the ball.

And this is just in case.

Tape it down.

So as I'm going to push this now, give it a push

the gun will be triggered

when the middle of the car is here.

You've seen how high that ball goes

so that ball will go... (makes whooshing sound)

And depending upon how hard I push it

they may meet here or they may meet there.

You ready for this?

You ready?

CLASS: Ready.

LEWIN: I'm ready.

Physics works!

(class applauds)

LEWIN: See you Wednesday.