Matematika kelas 8 | cara menghitung panjang sisi segitiga siku-siku yang memiliki sudut 30° dan 60°
Summary
TLDRIn this tutorial, the video explains how to calculate the unknown sides of a right-angled triangle with angles of 30° and 60°. Using the properties of 30-60-90 triangles, the video walks through the step-by-step process of finding the lengths of sides AB and AC, given side BC as 8 cm. The video uses the proportional relationships of 1:√3:2 for the sides opposite to 30°, 60°, and 90° respectively. By applying these ratios, viewers learn how to solve for the unknown side lengths, with AB being 4 cm and AC being 4√3 cm.
Takeaways
- 😀 The tutorial focuses on solving for unknown sides in a right triangle with angles of 30° and 60°.
- 😀 The given triangle has a hypotenuse and two other sides with known ratios based on the angles.
- 😀 BC (opposite the 90° angle) is given as 8 cm.
- 😀 The triangle uses special ratios: opposite the 30° angle is 1, opposite the 60° angle is √3, and the hypotenuse is 2.
- 😀 The goal of the tutorial is to find the lengths of sides AB and AC using these ratios.
- 😀 AB is calculated as half of BC, yielding 4 cm.
- 😀 AC is calculated by multiplying BC by √3/2, resulting in 4√3 cm.
- 😀 The tutorial emphasizes using trigonometric ratios for a 30°-60°-90° triangle to determine side lengths.
- 😀 The process is shown step-by-step, making it easy to follow for students learning this concept.
- 😀 The method for calculating both AB and AC involves simple arithmetic and ratio manipulation.
- 😀 The video encourages viewers to understand the relationship between the angles and side lengths in a 30°-60°-90° triangle to solve similar problems.
Q & A
What is the problem presented in the video?
-The video demonstrates how to calculate the unknown sides of a right triangle with angles of 30° and 60°, where the length of side BC is given as 8 cm. The goal is to find the lengths of sides AB and AC.
How are the angles in the triangle related to the sides?
-In a right triangle with angles of 30° and 60°, the sides have a specific ratio. The side opposite the 30° angle is 1, the side opposite the 60° angle is √3, and the hypotenuse is 2.
What is the ratio of the sides of a right triangle with 30° and 60° angles?
-The ratio of the sides in a right triangle with angles of 30° and 60° is 1:√3:2, where 1 is the side opposite the 30° angle, √3 is the side opposite the 60° angle, and 2 is the hypotenuse.
What is the formula used to find the length of side AB?
-The formula used to find the length of side AB is AB = BC * (1 / √2), where BC is given as 8 cm.
What is the value of AB?
-Using the formula, AB = 8 * (1 / √2), the length of AB is calculated as 4 cm.
How do you find the length of side AC?
-To find the length of side AC, the formula used is AC = BC * (√3 / 2), where BC is given as 8 cm.
What is the value of AC?
-Using the formula, AC = 8 * (√3 / 2), the length of AC is 4√3 cm, or approximately 6.93 cm.
Why is the ratio for the side opposite the 30° angle 1?
-In a right triangle with angles of 30° and 60°, the ratio for the side opposite the 30° angle is 1 because it is a fundamental property of such triangles, where the side opposite the 30° angle is always half the hypotenuse.
What does the term 'sides of a right triangle' refer to?
-The term 'sides of a right triangle' refers to the three edges of the triangle: the hypotenuse (the longest side opposite the right angle), and the two legs (the other two sides), one of which is opposite the 30° angle and the other opposite the 60° angle.
How do you apply the triangle ratios to solve for the unknown sides?
-The triangle ratios are applied by using the known length of one side (BC) and the ratios of the sides. For example, to find AB, you multiply BC by the ratio corresponding to the side opposite the 30° angle. For AC, you use the ratio corresponding to the side opposite the 60° angle.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video
¿Qué son las razones trigonométricas? @MatematicasprofeAlex

TRIGONOMETRIA NO TRIÂNGULO RETÂNGULO (EXERCÍCIOS) | Resumo de Matemática para o Enem
PERBANDINGAN TRIGONOMETRI (PART 1) SINUS, COSINUS, TANGEN, SECAN, COSECAN, COTANGEN

SEGITIGA ISTIMEWA SUDUT 30,60,90 DAN SUDUT 45,45,90
When Do I use Sin, Cos or Tan?
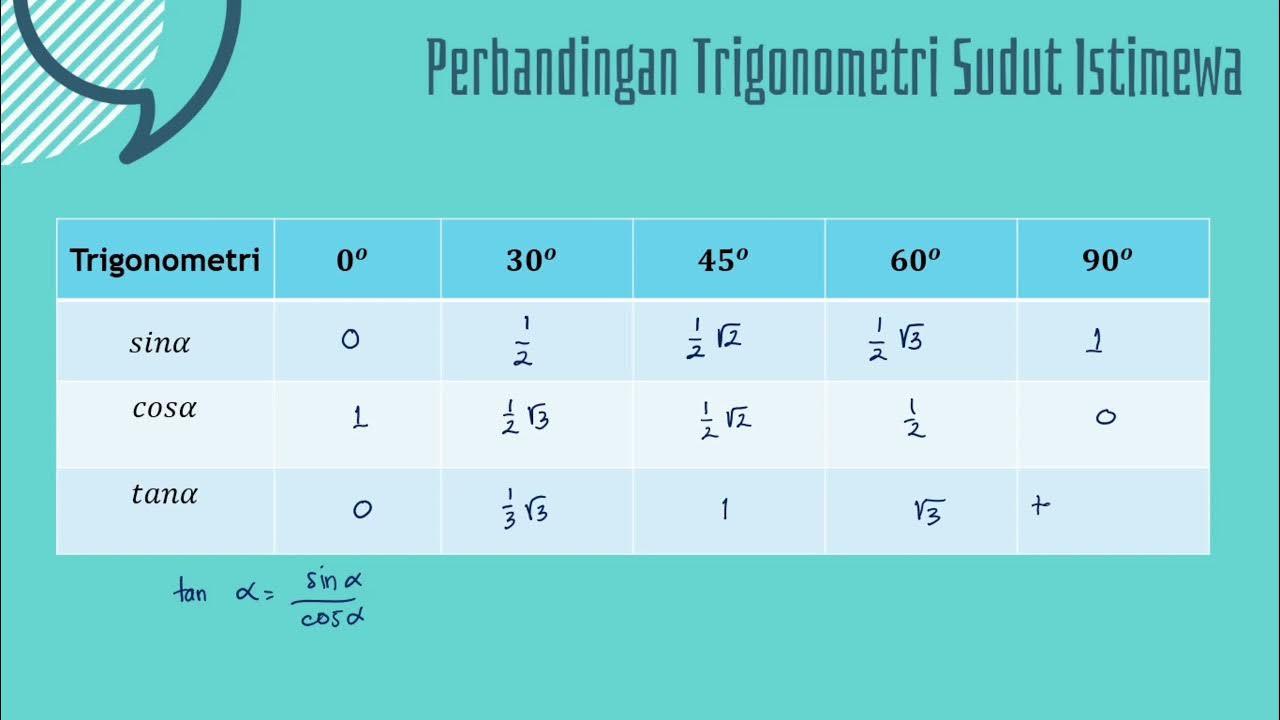
Perbandingan Trigonometri Sudut Istimewa
5.0 / 5 (0 votes)