Graph from slope-intercept equation example | Algebra I | Khan Academy

Summary

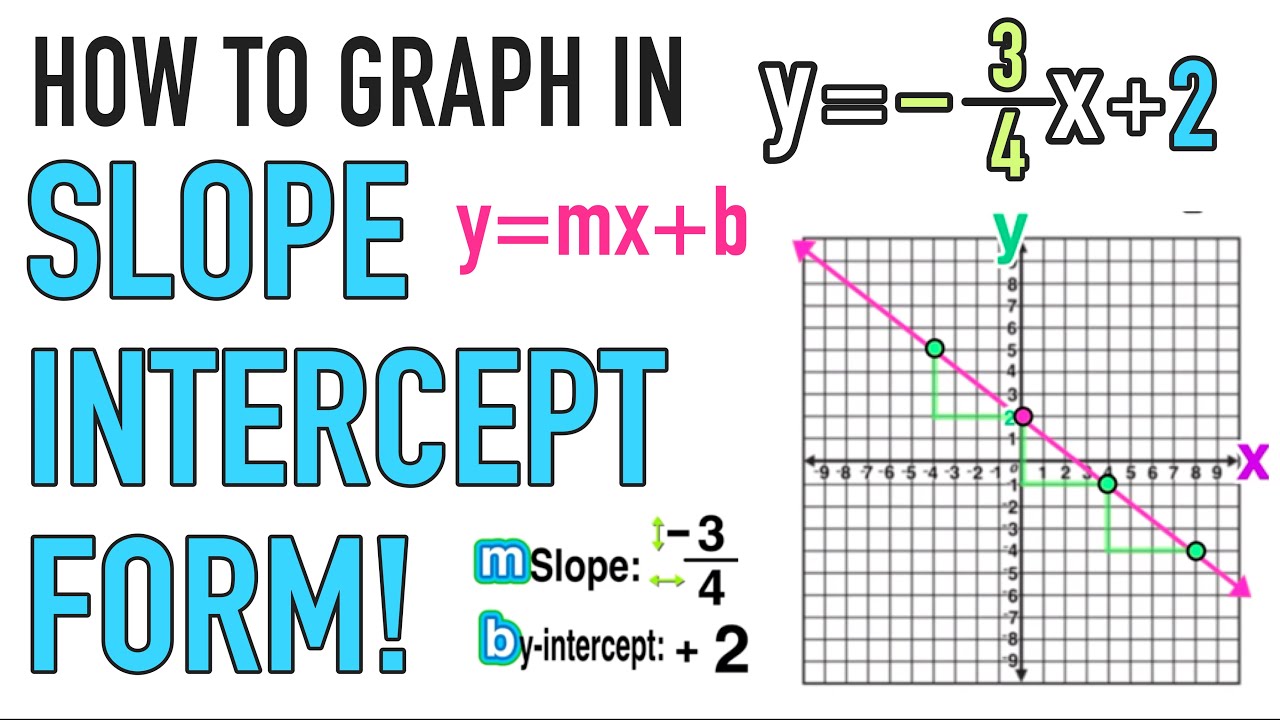
In this lesson, the process of graphing a line in slope-intercept form is explained. The equation y = 1/3x - 2 is used as an example, where the slope (m) is 1/3 and the y-intercept (b) is -2. The video details how to interpret the slope as the ratio of change in y to change in x and illustrates the graphing steps, starting from the y-intercept and using the slope to plot additional points. The lesson emphasizes understanding the relationship between slope and intercept to graph a straight line accurately.

Takeaways

• 😀 The equation given is in slope-intercept form: y = mx + b, where m is the slope and b is the y-intercept.
• 😀 The slope of the given equation y = (1/3)x - 2 is m = 1/3, indicating the line rises by 1 unit for every 3 units it moves to the right.
• 😀 The y-intercept of the equation is b = -2, meaning the line crosses the y-axis at the point (0, -2).
• 😀 The y-intercept occurs when x = 0, simplifying the equation to y = b, which verifies that the line intersects at (0, -2).
• 😀 The slope of 1/3 can be interpreted as 'rise over run' (change in y over change in x).
• 😀 The equation's slope means that for every 3 units moved horizontally to the right (positive x direction), the graph moves 1 unit up vertically.
• 😀 Starting from the y-intercept (0, -2), moving 3 units to the right results in the point (3, -1).
• 😀 The same slope allows for consistent spacing of points along the line, such as (6, 0) when x increases by 3 more units.
• 😀 The slope's ratio (1/3) works symmetrically; moving 3 units to the left (negative x direction) will move the graph 1 unit down.
• 😀 The final graph of the equation is a straight line, which is drawn through the points (0, -2), (3, -1), and (6, 0).

Q & A

What is the general form of the equation used in this video?

The general form of the equation is y = mx + b, where m represents the slope and b represents the y-intercept.

What does the slope of the line tell us in this equation?

The slope of the line tells us how much the value of y changes for a given change in x. In this case, the slope is 1/3, meaning for every 3 units moved horizontally (along the x-axis), the y value increases by 1 unit.

How do we identify the y-intercept from the equation?

The y-intercept is the value of y when x is equal to 0. In the equation y = (1/3)x - 2, the y-intercept is -2, meaning the line intersects the y-axis at the point (0, -2).

What does the value of b represent in the equation y = mx + b?

The value of b represents the y-intercept, which is the point where the line crosses the y-axis. It is the value of y when x = 0.

How can we verify that the y-intercept is correct?

To verify the y-intercept, substitute x = 0 into the equation. For y = (1/3)x - 2, when x = 0, the equation simplifies to y = -2, confirming the y-intercept is indeed -2.

What is the significance of the slope value 1/3?

The slope value of 1/3 means that for every 3 units you move to the right along the x-axis, the y value will increase by 1 unit. This gives us the ratio of vertical to horizontal change in the line.

How can we plot more points on the graph using the slope?

To plot more points, starting from the y-intercept (0, -2), move 3 units to the right and 1 unit up to plot the next point. You can repeat this process for additional points. Alternatively, you can move 3 units to the left and 1 unit down.

What happens if we move 3 units to the left on the graph?

If we move 3 units to the left on the x-axis, the slope tells us that the y value will decrease by 1 unit. This means the point will move down by 1 unit on the graph.

How would you describe the overall shape of the graph for this equation?

The graph of the equation y = (1/3)x - 2 is a straight line with a positive slope, meaning the line rises gradually as we move from left to right. The line intersects the y-axis at -2.

What is the relationship between x and y in this equation?

The equation y = (1/3)x - 2 describes a linear relationship between x and y. As x changes, y changes according to the slope (1/3) and the y-intercept (-2). For every 3 units increase in x, y increases by 1 unit.