12-5 Angle Relationships in Circles

Summary

This video explains angle relationships in circles, focusing on inscribed angles, secant-tangent intersections, and angles formed inside or outside a circle. It covers theorems related to angles formed by secants, tangents, and their interactions with arcs. Key concepts include how to calculate angle measures when the vertex is on the circle (half the arc), inside the circle (half the sum of two arcs), and outside the circle (half the difference of two arcs). Several examples are provided to reinforce these concepts, allowing viewers to practice and apply these geometric principles effectively.

Takeaways

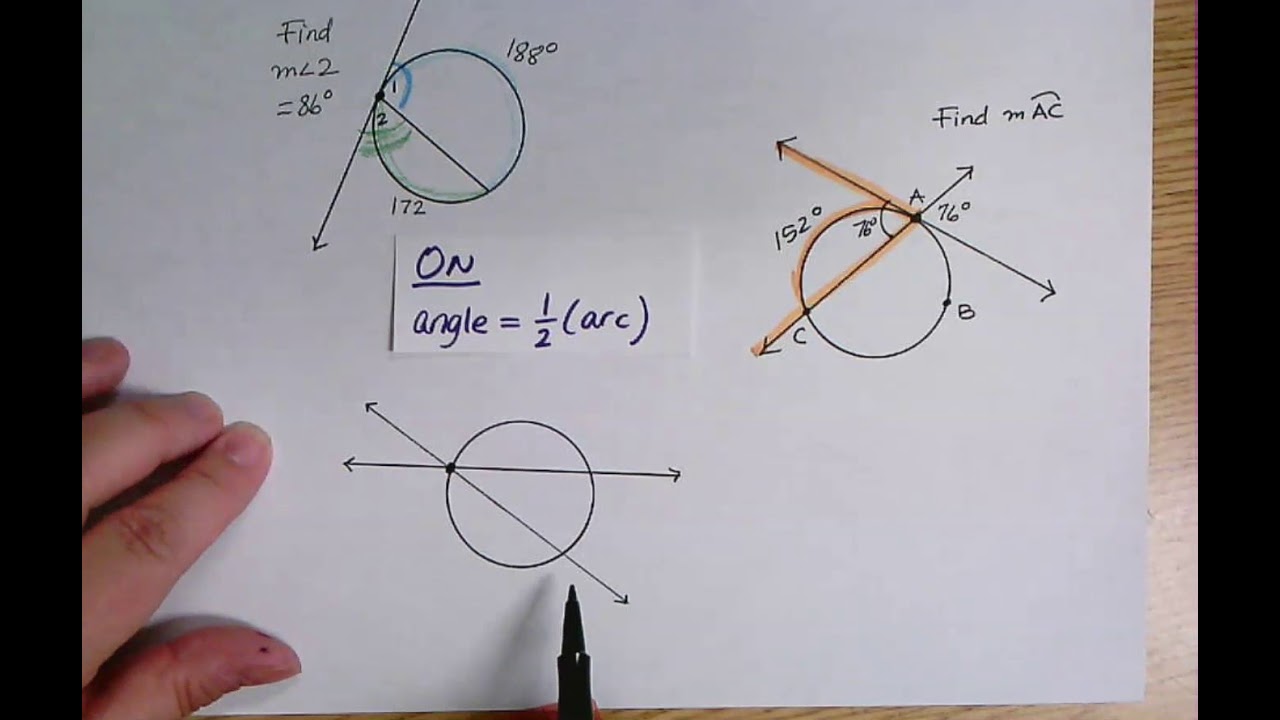
• 😀 When the vertex of an angle is on the circle, the angle is half the measure of the intercepted arc.
• 😀 The measure of an arc can be calculated by multiplying the inscribed angle by 2, as long as the vertex is on the circle.
• 😀 If the vertex is inside the circle, the angle is half the sum of the two intercepted arcs from the angle and its vertical counterpart.
• 😀 For an angle with its vertex outside the circle, the measure of the angle is half the difference between the two intercepted arcs.
• 😀 In problems involving secant and tangent lines, the angle is still half of the intercepted arc if the vertex is on the circle.
• 😀 If you have two tangents or two secants, the formula for calculating the angle remains the same: half of the difference of the two arcs.
• 😀 When two tangent lines meet at a point outside the circle, the arc between them can be calculated by subtracting the given arcs from 360°.
• 😀 To find the measure of an arc when multiple arcs are involved, subtract the known arcs from 360° to find the remaining arc.
• 😀 If the vertex is inside the circle and intercepts arcs with a linear pair, the angle can be found by using half the sum of the intercepted arcs.
• 😀 When solving for an angle outside the circle, always subtract the smaller arc from the larger one before halving the result.

Q & A

What is the relationship between the vertex of an angle and its intercepted arc when the vertex is on the circle?

When the vertex of an angle is on the circle, the measure of the angle is always half the measure of the intercepted arc.

How do you calculate the measure of an angle when the vertex is inside the circle?

When the vertex of an angle is inside the circle, the measure of the angle is half the sum of the measures of the two arcs that the angle intercepts.

What formula is used to find the measure of an angle when the vertex is outside the circle?

When the vertex is outside the circle, the measure of the angle is half the difference between the two intercepted arcs.

How can you find the measure of angle BCD if the measure of arc BC is 142° and angle ACD is 90°?

Since the vertex of angle BCD is on the circle, the measure of angle BCD is half of the measure of arc BC. Therefore, angle BCD is 71°.

How do you calculate the measure of arc ABC if angle ACD is 90°?

To calculate the measure of arc ABC, multiply the measure of angle ACD (90°) by 2. This gives a measure of 180° for arc ABC.

In the case where the vertex of an angle is outside the circle, which arc is subtracted to calculate the angle?

When the vertex is outside the circle, the larger (outer) arc is subtracted by the smaller (inner) arc to calculate the angle.

If the measure of arc AD is 174° and arc BD is 98°, what is the measure of angle X, assuming the vertex is outside the circle?

The measure of angle X is half the difference between arc AD and arc BD. So, 174° - 98° = 76° and half of that is 38°.

What should be done to calculate the measure of an angle formed by two tangent lines to a circle?

When the angle is formed by two tangent lines, the angle is calculated by taking half the difference of the larger outer arc and the smaller inner arc, with the entire circle contained between the tangents.

How can you find the measure of arc AF if you know that arcs 48°, 160°, and 60° are already accounted for in the circle?

To find the measure of arc AF, subtract the measures of the three known arcs (48°, 160°, and 60°) from 360°. This gives the measure of arc AF as 92°.

In the example where the angle is 25° and the outside arc is 83°, how would you solve for X, the inside arc?

To solve for X, first multiply the angle by 2 to get 50°. Then, subtract this from the outside arc, so 83° - 50° = 33°. Therefore, the inside arc X is 33°.