Sudut Pusat dan Sudut Keliling Lingkaran - Matematika SMA Kelas XI Kurikulum Merdeka

Summary

This video explains the concepts of central and inscribed angles in a circle, highlighting their differences and key relationships. The central angle, whose vertex is at the circle's center, is always twice the size of the inscribed angle that subtends the same arc. The video provides clear examples to demonstrate these properties, including a practical exercise where viewers can apply these concepts. The presenter emphasizes the importance of understanding the relationship between these angles to solve geometry problems involving circles.

Takeaways

• 😀 Sudut Pusat (Central Angle) is an angle with its vertex at the center of the circle, and its legs are the radii of the circle.
• 😀 Sudut Keliling (Inscribed Angle) is an angle with its vertex on the circumference of the circle, and its legs are the chords of the circle.
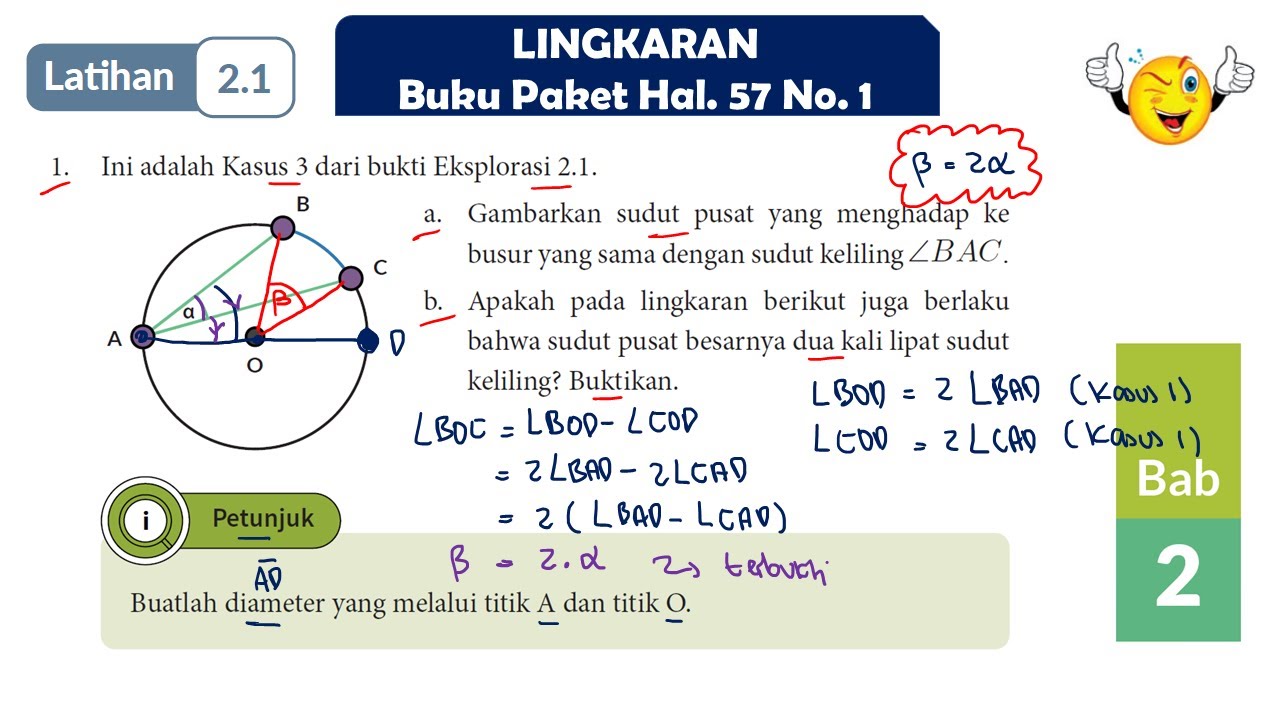
• 😀 The central angle is always twice the size of the inscribed angle when they subtend the same arc.
• 😀 The formula for the relationship between the central angle and the inscribed angle is: Sudut Pusat = 2 × Sudut Keliling.
• 😀 Two inscribed angles subtending the same arc are congruent (equal in size).
• 😀 If Sudut Pusat and Sudut Keliling subtend the same arc, the central angle is always twice the size of the inscribed angle.
• 😀 In the example problem, if ∠ABD (inscribed angle) is 46°, the inscribed angle ∠ACD will also be 46°, as both subtend the same arc.
• 😀 For central angles, ∠APD (central angle) = 2 × ∠ABD. If ∠ABD = 46°, then ∠APD = 92°.
• 😀 It’s important to recognize that Sudut Pusat has its vertex at the circle’s center, while Sudut Keliling’s vertex lies on the circle’s circumference.
• 😀 The two main properties of angles in a circle that must be understood are: 1) Sudut Pusat = 2 × Sudut Keliling for the same arc, and 2) Two Sudut Keliling subtending the same arc are equal.

Q & A

What is a central angle in a circle?

A central angle is an angle whose vertex is at the center of the circle, and whose arms are the radii of the circle. It subtends an arc of the circle.

How is an inscribed angle different from a central angle?

An inscribed angle is an angle whose vertex is on the circle and whose arms are chords of the circle. In contrast, a central angle has its vertex at the center of the circle and its arms as radii.

What is the key property that relates a central angle and an inscribed angle that subtend the same arc?

The central angle is always twice the measure of the inscribed angle that subtends the same arc. In other words, if a central angle and an inscribed angle subtend the same arc, the central angle is 2 times larger than the inscribed angle.

What does it mean when two inscribed angles are equal?

If two inscribed angles subtend the same arc, they are equal in measure. This is a key property of inscribed angles in circles.

In the example provided in the script, what is the relationship between ∠APB and ∠ACB?

In the example, ∠APB is a central angle and ∠ACB is an inscribed angle. The measure of ∠APB is always twice the measure of ∠ACB because they subtend the same arc AB.

What is the formula for calculating the central angle when you know the inscribed angle that subtends the same arc?

The formula is: Central Angle = 2 × Inscribed Angle. If the inscribed angle is known, multiply it by 2 to find the central angle.

If ∠ABD is 46 degrees, what is the measure of ∠ACD in the example from the script?

Since ∠ACD is an inscribed angle subtended by the same arc AD as ∠ABD, and both angles are inscribed, they are equal. Therefore, ∠ACD = 46 degrees.

How do you find the measure of a central angle given an inscribed angle that subtends the same arc?

To find the measure of a central angle, you multiply the measure of the inscribed angle by 2. For example, if the inscribed angle is 46 degrees, the central angle will be 92 degrees.

What is the significance of the relationship between a central angle and an inscribed angle in solving geometry problems?

This relationship is crucial for solving problems involving circles, as it allows you to calculate unknown angles based on known ones, making it easier to understand and apply geometric principles in circle-related problems.

Can an inscribed angle and a central angle ever be equal?

Yes, an inscribed angle and a central angle can only be equal if the inscribed angle is 0 degrees, meaning the arc they subtend is nonexistent or degenerate. This would happen if the vertex of the inscribed angle coincides with the center of the circle, which is a special case.