Menentukan Model Matematika
Summary
TLDRIn this educational video, the instructor explains how to solve real-life problems using systems of linear equations. The first problem involves calculating the number of goats and chickens in a cage, based on their total count and leg numbers. The second problem involves determining the cost of books and pencils based on their prices and quantities purchased by different people. The video walks through the process of setting up mathematical models, applying substitution, elimination, and graphical methods to solve the systems, and solving practical applications like pricing. It concludes with an emphasis on understanding the goal and careful model formulation.
Takeaways
- 😀 The video explains how to determine mathematical models in everyday life using systems of linear equations with two variables.
- 😀 The first example involves a pen with goats and chickens, where the total number of animals and legs are used to form a system of linear equations.
- 😀 The variables used in the first problem are 'x' for goats and 'y' for chickens, with the system of equations being x + y = 13 and 4x + 2y = 32.
- 😀 To solve the system of equations, the video discusses three methods: substitution, elimination, and graphing.
- 😀 The second example focuses on a bookstore purchase involving books and pencils, where the goal is to find the total cost for Citra's purchase of 6 books and 2 pencils.
- 😀 In the second problem, 'x' represents the price of a book, and 'y' represents the price of a pencil, with the system of equations being 4x + 3y = 12,500 and 2x + y = 5,500.
- 😀 After forming the system of equations in the second problem, the solution involves finding the values of x and y (prices) using one of the three methods.
- 😀 The second problem also introduces an objective function: to calculate the total cost for Citra's purchase, represented by 6x + 2y.
- 😀 The video stresses the importance of carefully identifying the variables and the objective function when solving word problems with linear equations.
- 😀 Viewers are encouraged to watch the previous video if they need a refresher on the methods of solving systems of linear equations and applying them to real-life scenarios.
Q & A
What is the primary topic of the video?
-The primary topic of the video is how to determine a mathematical model for real-life problems, specifically focusing on solving systems of linear equations with two variables.
How is the number of goats and chickens represented in the first problem?
-In the first problem, the number of goats is represented by the variable 'x' and the number of chickens is represented by the variable 'y'.
What does the first equation represent in the first problem (x + y = 13)?
-The equation 'x + y = 13' represents the total number of animals in the cage, which consists of both goats and chickens. The sum of goats (x) and chickens (y) equals 13.
Why is the second equation in the first problem 4x + 2y = 32?
-The second equation '4x + 2y = 32' represents the total number of legs of all animals. Each goat has 4 legs, and each chicken has 2 legs. Thus, the total number of legs is 32.
What methods can be used to solve the system of equations in the first problem?
-The system of equations in the first problem can be solved using three methods: substitution, elimination, and graphing.
In the second problem, what are the variables and what do they represent?
-In the second problem, 'x' represents the price of one notebook, and 'y' represents the price of one pencil.
What do the equations 4x + 3y = 12,500 and 2x + y = 5,500 represent in the second problem?
-The first equation '4x + 3y = 12,500' represents the total cost when Beni buys 4 notebooks and 3 pencils for 12,500 IDR. The second equation '2x + y = 5,500' represents the total cost when Udin buys 2 notebooks and 1 pencil for 5,500 IDR.
What is the objective of the second problem involving Citra's purchase?
-The objective of the second problem is to determine how much Citra has to pay for 6 notebooks and 2 pencils by using the values of 'x' and 'y' found from solving the system of equations.
What is the importance of understanding the 'objective function' in word problems like the second one?
-Understanding the 'objective function' is crucial in word problems because it helps identify the specific quantity being asked for, such as the total cost or the number of items, which guides how to set up and solve the system of equations.
Why is it important to carefully analyze the problem and set up the correct system of equations?
-It is important to carefully analyze the problem and set up the correct system of equations because it ensures that the mathematical model reflects the real-world situation accurately, allowing for correct and meaningful solutions.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video

SPLTV Kelas 10 Kurikulum Merdeka

SISTEM PERSAMAAN LINEAR 3 VARIABEL / Mat kls 10

ILLUSTRATING SYSTEMS OF LINEAR EQUATIONS IN TWO VARIABLES || GRADE 8 MATHEMATICS Q1
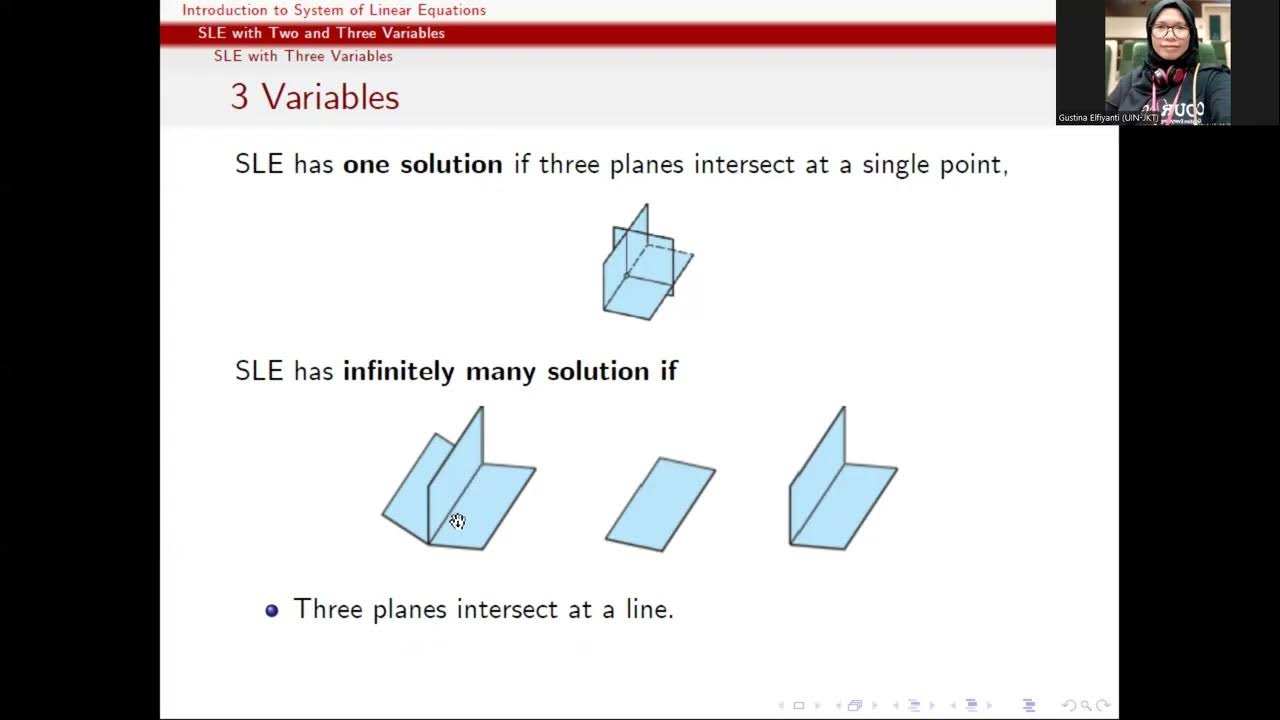
Introduction to SLE

Kurikulum Merdeka Matematika Kelas 9 Bab 1 Sistem Persamaan Linear Dua Variabel

SOLVING SYSYEM OF NONLINEAR EQUATIONS || PRECALCULUS
5.0 / 5 (0 votes)