Fluidos Ideais em Movimento parte I

Summary

This video explains the complex dynamics of fluid motion, specifically focusing on ideal fluids in a stationary, incompressible, non-viscous, and irrotational flow. It covers concepts like steady flow, where particles move with consistent velocity, and how this relates to real-life examples like water flowing through a channel or smoke exiting a cigarette. The script also dives into the idea of streamlines, the continuity equation for volume flow, and the behavior of fluids in confined spaces, like narrowing tubes. The video aims to make these fluid mechanics principles accessible by breaking them down into key concepts with clear examples.

Takeaways

• 😀 The study of fluid motion is complex, especially for real fluids, but the discussion focuses on ideal fluid flow.
• 😀 The flow under consideration is steady, incompressible, non-viscous, and irrotational.
• 😀 Steady flow means that particles passing a fixed point always have the same velocity over time.
• 😀 In incompressible flow, the fluid's density remains constant throughout the movement.
• 😀 Viscosity is a measure of a fluid's resistance to flow, with more viscous fluids like oil flowing slower than less viscous fluids like water.
• 😀 Irrotational flow means that there is no rotational motion in the flow, as seen in the analogy with a non-rotating wheel in a fluid.
• 😀 Streamlines are used to represent the trajectories of particles in steady flow, and they do not cross each other.
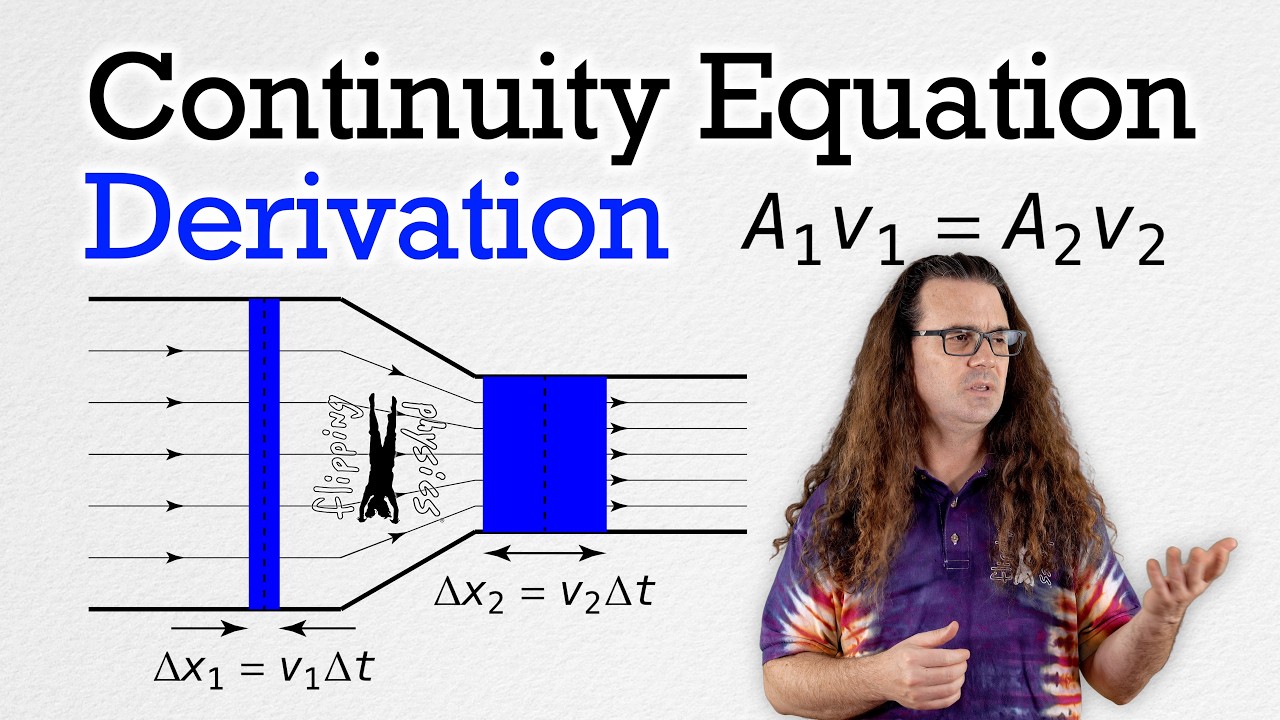
• 😀 In a pipe with varying cross-sectional areas, the flow speed increases where the area narrows to keep the flow rate constant.
• 😀 The continuity equation states that the product of the cross-sectional area and the velocity is constant along a streamline.
• 😀 The flow behavior in the pipe can be described by using the continuity equation: A1 * V1 = A2 * V2, where A is the area and V is the velocity at different points.
• 😀 A tube of flow is formed by streamlines passing through a closed curve and behaves as if it were a real physical tube, ensuring that the same volume of fluid enters and exits the tube.

Q & A

What does the script define as a fluid ideal for study in hydraulics?

The script defines an ideal fluid as one that exhibits stationary, incompressible, non-viscous, and irrotational flow.

What is meant by 'steady flow' in fluid dynamics?

In steady flow, different fluid particles passing through a fixed point always move with the same velocity at any given time.

What is the difference between steady and turbulent flow?

Steady flow maintains constant velocity for fluid particles at a given point, while turbulent flow involves irregular and chaotic movement of fluid particles, where velocities vary significantly at different points.

What is an incompressible flow in fluid dynamics?

In an incompressible flow, the fluid density remains constant and uniform across the flow at all times and points, meaning no changes in volume occur within the fluid.

How does viscosity affect fluid flow?

Viscosity is a measure of a fluid's resistance to flow. A fluid with higher viscosity, such as oil, resists flow more than a less viscous fluid like water.

What does 'irrotational flow' mean in the context of fluid dynamics?

Irrotational flow refers to a flow where the fluid particles do not rotate around their own axis. This means there is no angular velocity at any point in the flow.

How does the concept of streamlines apply to steady flow?

In steady flow, streamlines represent the paths followed by fluid particles, and these streamlines do not intersect. They provide a visual representation of the fluid’s velocity field.

What happens to the velocity of fluid in a narrowing channel?

In a narrowing channel, the fluid velocity increases. This is because the flow rate must remain constant, and as the cross-sectional area decreases, the velocity must increase to maintain the same volume of flow.

What is the principle of conservation of mass in fluid dynamics as applied to a pipe?

The principle of conservation of mass in fluid dynamics, as applied to a pipe, states that the volume flow rate must remain constant across any cross-section of the pipe. This means the product of the cross-sectional area and fluid velocity must be the same at all points along the flow.

What is the equation of continuity and how is it derived?

The equation of continuity is derived from the principle of conservation of mass. It states that the flow rate, represented as the product of the cross-sectional area and velocity, remains constant along the pipe. Mathematically, A1v1 = A2v2, where A is the area and v is the velocity at two different points.