Prinsip Dinamika Fluida | Fluida | Part 4 | Fisika Dasar

Summary

The video explores the key principles of fluid dynamics, particularly focusing on ideal fluids. It explains concepts such as incompressibility, non-viscosity, steady flow, and irrotational flow, making complex topics more accessible. The ideal fluid is defined as one with constant density, no internal friction, steady flow, and no rotational behavior. The principle of continuity in fluid dynamics is also introduced, highlighting how fluid flow remains constant through a system. The application of this principle in real-world scenarios, like rivers splitting into branches, is demonstrated to make the concepts clear and practical.

Takeaways

• 😀 Ideal fluids are characterized by four key properties: incompressibility, non-viscosity, steady flow, and irrotationality.
• 😀 Incompressible fluids maintain a constant density regardless of external pressure, unlike compressible fluids where density changes with pressure.
• 😀 Non-viscosity means ideal fluids have no internal friction between their layers, unlike real fluids that experience friction and energy loss.
• 😀 Steady flow indicates that the velocity of fluid at any given point remains constant over time, though it may vary across different points in space.
• 😀 Irrotational flow means the fluid particles do not rotate around their own axes, ensuring no vortex or swirling motion in the flow.
• 😀 The principle of continuity states that the flow rate (debit) remains constant in an incompressible fluid, regardless of changes in the cross-sectional area of the pipe.
• 😀 The equation for the principle of continuity is A1V1 = A2V2, where A is the cross-sectional area and V is the velocity of the fluid.
• 😀 A practical example shows that when a river branches, the total flow rate remains constant, but the velocity adjusts according to the change in the cross-sectional area.
• 😀 Understanding fluid dynamics helps simplify complex concepts by focusing on idealized, simplified fluid properties, allowing for more manageable calculations and predictions.
• 😀 The key to analyzing fluid behavior in channels is applying the continuity equation, which ensures the volume flow remains constant despite changes in the pipe's shape or size.

Q & A

What is the main focus of the video discussed in the script?

The video focuses on the principles of fluid dynamics, specifically examining ideal fluids and their properties, including incompressibility, non-viscosity, steady flow, and irrotational flow.

What is the difference between fluid statics and fluid dynamics?

Fluid statics deals with fluids that are at rest, whereas fluid dynamics studies the behavior of fluids in motion, which is the focus of this video.

What defines an ideal fluid?

An ideal fluid is one that is incompressible, non-viscous, exhibits steady flow, and is irrotational. These properties simplify the study of fluid flow in various contexts.

What does 'incompressible' mean in the context of fluid dynamics?

Incompressible means that the fluid’s density remains constant regardless of the pressure applied, so the mass per unit volume does not change.

How does a compressible fluid differ from an incompressible one?

A compressible fluid experiences changes in its density when pressure is applied, whereas an incompressible fluid maintains a constant density despite changes in pressure.

What is viscosity, and how does it affect fluid flow?

Viscosity refers to the internal friction or resistance to flow within a fluid. A fluid with high viscosity (like honey) resists flow more than one with low viscosity (like water). Non-viscous fluids are idealized as having negligible resistance to flow.

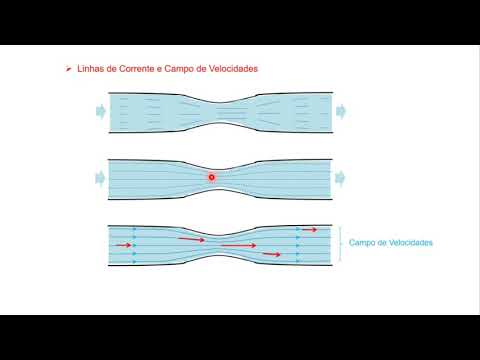
What is meant by 'steady flow' or 'tunak' in fluid dynamics?

'Steady flow' means that the velocity of the fluid at any given point does not change over time. The flow pattern remains consistent even as the fluid moves.

What is the significance of 'irrotational' flow in fluid dynamics?

Irrotational flow means that the fluid particles do not rotate about their center of mass as they move. This is idealized in fluid dynamics because it simplifies the analysis of the flow.

What does the Continuity Principle state about fluid flow?

The Continuity Principle states that the flow rate (debit) of an ideal fluid remains constant at any point along a pipe or channel, meaning the volume of fluid passing through one section per unit of time is equal to that passing through any other section.

How does the Continuity Principle apply to varying pipe cross-sections?

According to the Continuity Principle, if the cross-sectional area of a pipe decreases, the velocity of the fluid increases to maintain the constant flow rate. Conversely, if the area increases, the velocity decreases.