Continuity Equation for Ideal Fluid Flow - Derivation

Summary

This video explains the continuity equation of ideal fluid flow through a circular pipe. It covers key concepts like mass flow rate, velocity, and cross-sectional area in relation to the fluid's movement. The flow is non-viscous, incompressible, and laminar, ensuring that the mass passing through different sections of the pipe remains constant. By examining the relationship between the pipe's diameter and velocity, the video demonstrates how the fluid's velocity increases when the cross-sectional area decreases. The concept is explained through everyday examples, like using a thumb to control water flow from a garden hose, offering practical insights into fluid dynamics.

Takeaways

• 😀 Ideal fluid flow refers to a nonviscous, incompressible, laminar, and irrotational flow through a pipe.
• 😀 The mass of fluid flowing through two planes in the pipe must be the same, assuming no losses of fluid, because the fluid is incompressible.
• 😀 The equation for density is mass over volume, and we consider the mass of the fluid passing through a plane during a specific time interval (delta t).
• 😀 The displacement of the fluid through each plane is related to velocity and the change in time (delta t).
• 😀 The volume of fluid passing through a plane is the cross-sectional area times the displacement (delta x) of the fluid.
• 😀 The mass passing through plane 1 equals the mass passing through plane 2, so the equation for mass flow is consistent across planes.
• 😀 The continuity equation for fluid flow states that the mass flow rate remains constant across planes in the pipe.
• 😀 The mass flow rate has units of kilograms per second, showing that mass is conserved as the fluid moves through the pipe.
• 😀 In ideal fluid flow, the density of the fluid remains constant, so it can be removed from the mass flow rate equation.
• 😀 The continuity equation of ideal fluid flow focuses on volumetric flow rate, where the cross-sectional area times the velocity remains constant.
• 😀 When the cross-sectional area of a pipe decreases, the velocity of the fluid increases to maintain constant volumetric flow, as demonstrated by the example with a garden hose.

Q & A

What is meant by ideal fluid flow in the context of the script?

Ideal fluid flow refers to a flow where the fluid is nonviscous, incompressible, and the flow is laminar and irrotational, meaning there is no turbulence and the fluid behaves like a perfect, ideal substance.

Why does the mass of fluid passing through plane 1 have to be the same as the mass passing through plane 2?

The mass flow rate must be constant because the fluid is incompressible. If there were a loss or accumulation of mass, the fluid would be compressible, which is not the case in this ideal fluid scenario.

What equation is used to represent the density of the fluid?

The equation for density is: density = mass / volume.

What is the significance of using delta m and delta V in the mass flow rate equation?

Delta m represents the mass of the fluid passing through a plane during a time interval (delta t), while delta V represents the volume of the fluid passing through that same plane during the same time interval.

How is the displacement of the fluid through the pipe related to the velocity?

Displacement is related to velocity through the equation: displacement = velocity * time. This helps determine how far the fluid moves through the pipe during a given time interval.

What is the continuity equation of fluid flow?

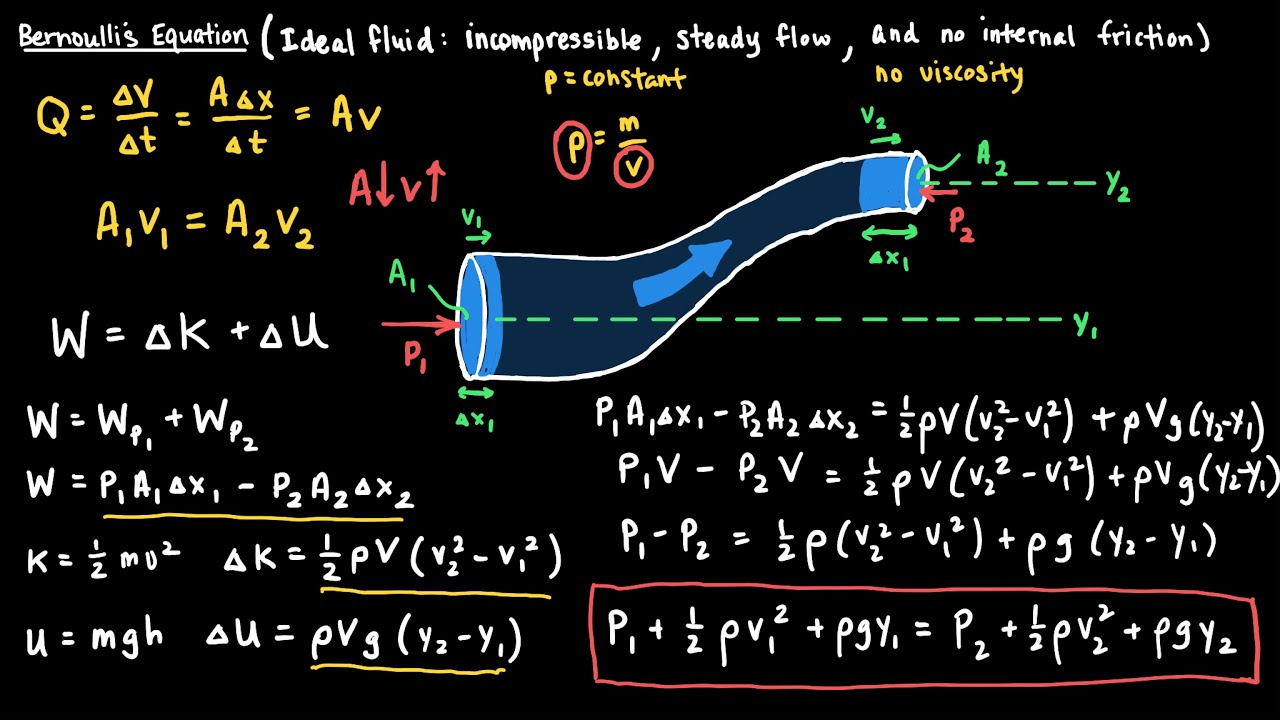
The continuity equation states that the mass flow rate of an ideal fluid in an enclosed space is constant. It is expressed as: density * cross-sectional area * velocity = constant.

How do the units of the continuity equation check out?

The units for density are kilograms per meter cubed (kg/m³), for area are meters squared (m²), and for velocity are meters per second (m/s). When multiplied together, they cancel out appropriately, leaving the unit of mass flow rate, which is kilograms per second (kg/s).

What does it mean when the density is removed from the continuity equation for ideal fluids?

For ideal fluids, density remains constant due to incompressibility. As a result, it can be removed from the equation, simplifying it to: area * velocity = constant, which is the volumetric flow rate equation.

What happens to the velocity of the fluid when the diameter of the pipe decreases, based on the continuity equation?

As the diameter of the pipe narrows, the cross-sectional area decreases, and the velocity of the fluid must increase to maintain the same volumetric flow rate, as per the continuity equation.

How can the continuity equation be demonstrated using a garden hose?

By putting a thumb over the end of a garden hose, the cross-sectional area at the nozzle is reduced, causing the velocity of the water to increase. This is a real-world example of how the continuity equation works: as the area decreases, velocity increases to maintain constant volumetric flow.