Materi 6 - Nilai Optimum fungsi Objektif
Summary
TLDRThis video focuses on the concept of 'optimal values' in linear programming, explaining the maximum and minimum optimal values in various real-world scenarios, such as production costs and profits. It covers the method of graphing, finding intersections, and solving problems using objective functions. Through examples like maximizing profits from selling cakes and minimizing production costs of furniture, the video illustrates step-by-step techniques for solving these problems. Additionally, it emphasizes the importance of understanding limits, preparing students for more advanced topics in mathematics.
Takeaways
- 😀 Linear programming focuses on optimizing objective functions, with the highest difficulty level involving both inequalities and graphical solutions.
- 😀 The concept of 'optimum value' includes both maximum and minimum values, which are crucial in real-world problems like production cost and profit maximization.
- 😀 In the given example, the goal is to maximize revenue from selling two types of cakes—chocolate and cheese—by determining the best combination of cakes to produce.
- 😀 The first step in solving such problems involves writing mathematical models, where the objective function is formed by multiplying the price of each product by the number of units produced.
- 😀 After formulating the objective function, a graph is drawn to visually represent the constraints and find the feasible region for the solution.
- 😀 The feasible region is the area where all the constraints overlap, and the optimal solution lies at one of the corner points (vertices) of this region.
- 😀 To find the optimal solution, we calculate the objective function at each of the corner points and select the one with the maximum value in case of maximization problems.
- 😀 For the cake example, the revenue maximum occurs when 150 chocolate cakes and 100 cheese cakes are produced, resulting in a maximum revenue of Rp95,000.
- 😀 In a different example about a furniture company, the goal was to minimize production costs, where the objective function is again based on the cost per unit multiplied by the number of units produced.
- 😀 The process for solving the furniture production problem involves similar steps: formulating a mathematical model, drawing a graph to represent constraints, and finding the feasible region, with the optimal solution being the minimum cost.
- 😀 For the furniture example, the minimum production cost is achieved when 10 luxury cabinets and 4 sport cabinets are produced, with a total cost of Rp512,000.
Q & A
What is the concept of 'optimum value' in linear programming?
-In linear programming, the 'optimum value' refers to the maximum or minimum value that an objective function can take. This is used to find the best solution to a problem, such as maximizing profit or minimizing cost.
What is the difference between maximum and minimum optimum values?
-The maximum optimum value is used when the goal is to achieve the highest possible outcome, such as maximizing profit. The minimum optimum value is used when the goal is to reduce or minimize a particular outcome, such as minimizing production costs.
Can you provide an example where maximum optimum value is applied?
-An example of maximum optimum value is a bakery trying to maximize its revenue by selling two types of cakes. By determining the number of cakes to make and sell, the bakery can maximize its revenue, which is the maximum optimum value in this case.
How do you calculate the maximum revenue in a cake production scenario?
-To calculate the maximum revenue, you model the situation with an objective function that multiplies the price per cake by the number of cakes of each type. By solving the constraints and testing the feasible solutions, the maximum revenue can be identified from the feasible points.
What mathematical function is used to represent revenue in the cake production example?
-The revenue function in the cake production example is represented by the equation R = 300x + 500y, where 'x' is the number of chocolate cakes and 'y' is the number of cheese cakes. The coefficients 300 and 500 represent the prices per cake.
What is the purpose of graphing in linear programming optimization problems?
-Graphing is used to visualize the constraints and objective function. By plotting the feasible region and testing points where the objective function reaches its maximum or minimum, you can identify the optimal solution.
In the furniture production example, how is the minimum production cost calculated?
-The minimum production cost is calculated by setting up an objective function that multiplies the cost per unit by the number of units produced. The optimal solution is found by graphing the constraints, determining the feasible region, and evaluating the objective function at the feasible points.
What is the significance of the coordinates (x, y) in optimization problems?
-The coordinates (x, y) represent the number of units for each product or variable. These coordinates are found by solving the system of constraints and testing them within the objective function to determine the optimal solution.
Why is it important to round off non-integer solutions in production problems?
-In production problems, rounding off non-integer solutions is necessary because it is not possible to produce a fraction of a unit, such as 9.6 pieces of furniture. Rounding ensures the solution is practical and corresponds to whole units that can actually be produced.
How do you identify the feasible region in a linear programming problem?
-The feasible region is identified by graphing the constraints and shading the area that satisfies all conditions. This region represents all the possible solutions that meet the problem’s requirements.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video

Menentukan Nilai Optimum Menggunakan Metode Uji Titik Sudut (Uji Titik Pojok)
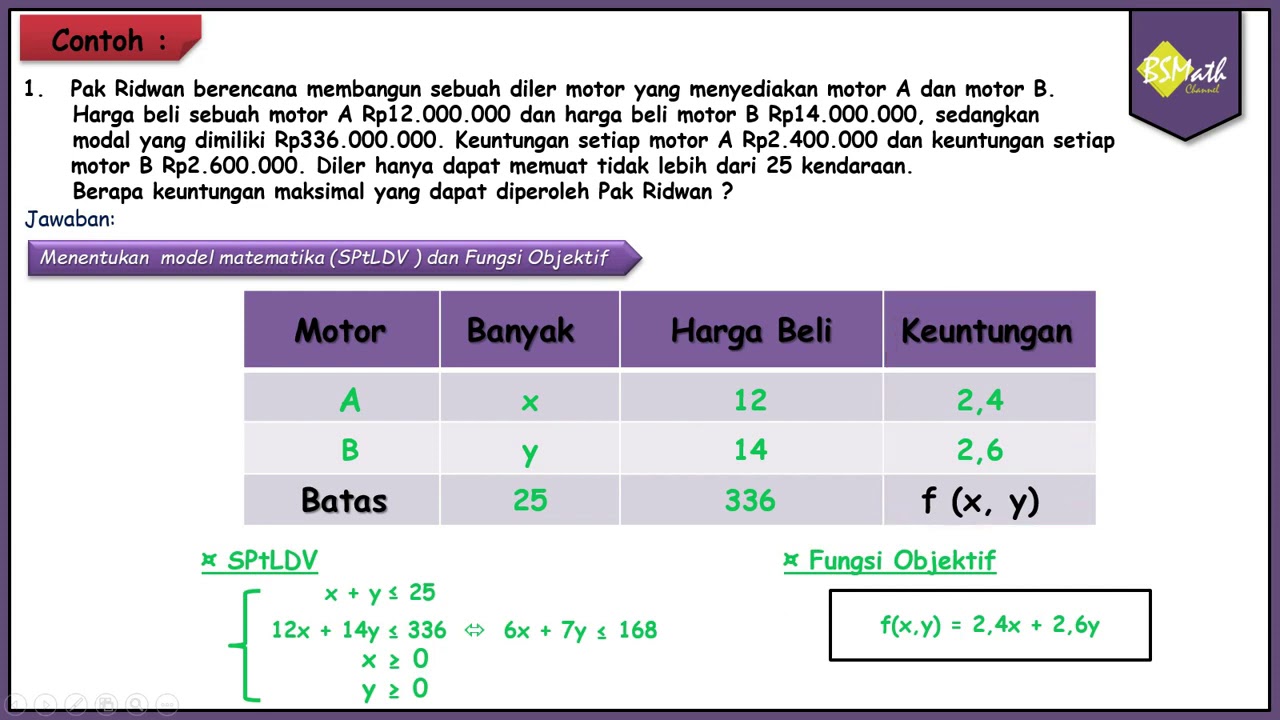
Menyelesaikan Permasalahan Program Linear Menentukan Nilai Optimum dengan Metode Uji Titik Pojok

ART TEACHES MATHEMATICS IN THE MODERN WORLD-LESSON 1: INTRO TO LINEAR PROGRAMMING

Ch04 Linear Programming
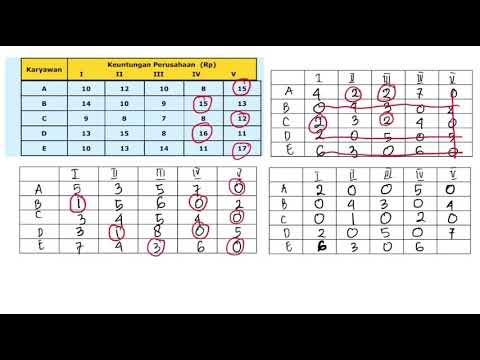
Metode Penugasan Kasus Maksimasi
AI, Hungarian Algo.Education
5.0 / 5 (0 votes)