Konsep Dasar Sistem Persamaan Linear Dua Variabel (SPLDV) | Matematika Wajib Kelas 10
Summary
TLDRIn this mathematics lesson for 10th-grade students, the teacher explains how to solve systems of linear equations with two variables. The lesson begins by defining what a system of linear equations is, then progresses to discussing its general form and how to identify if an equation is linear. The teacher provides two examples, demonstrating step-by-step how to eliminate variables and solve for the unknowns. The lesson emphasizes the importance of matching coefficients for elimination, and offers a clear explanation of substitution methods. By the end, students should be able to solve similar systems independently.
Takeaways
- 😀 Introduction to system of linear equations with two variables, explaining its connection to prior learning in middle school.
- 😀 A system of linear equations involves two or more equations with variables raised to the first power and related to each other, ensuring a single solution.
- 😀 An equation like '3x^2 + 5y = 6' is not a linear equation because it involves a squared term (x^2), which contradicts the definition of linearity.
- 😀 A system with more than two variables, like '3P + 5Q - R = 7', is not a system of linear equations with two variables.
- 😀 The general form of a linear equation with two variables is 'Ax + By = C', where A and B are coefficients, x and y are variables, and C is a constant.
- 😀 In a system of linear equations, the equations must be related so that the system has a single solution for the variables.
- 😀 A system of linear equations with two variables can be written as: 'A1x + B1y = C1' and 'A2x + B2y = C2'.
- 😀 When solving a system of linear equations, one of the variables (either x or y) is eliminated first by comparing the coefficients of the variables.
- 😀 In the first example problem, elimination of variable x is performed because the coefficients of x in both equations are the same.
- 😀 After finding the value of one variable (like y = 2), it is substituted back into one of the original equations to find the value of the other variable (x = 1).
- 😀 In the second example problem, when the coefficients are different, one equation is multiplied to make the coefficients match before eliminating a variable (in this case, y).
Q & A
What is the definition of a system of linear equations in two variables?
-A system of linear equations in two variables is a set of two or more linear equations with two variables that are interconnected, such that there is only one solution for the variables.
What makes an equation linear in two variables?
-An equation is linear in two variables if the highest powers of the variables are 1. This means no variables are squared or raised to higher powers.
Is the equation '3x^2 + 5y = 6' a linear equation in two variables?
-No, this is not a linear equation in two variables because the variable 'x' is squared (x^2), making it a non-linear equation.
What is the general form of a linear equation in two variables?
-The general form of a linear equation in two variables is 'ax + by = c', where 'a' and 'b' are the coefficients of the variables, 'x' and 'y' are the variables, and 'c' is the constant.
How do you solve a system of linear equations in two variables using elimination?
-To solve using elimination, you align the equations to make the coefficients of one of the variables the same in both equations. Then, subtract or add the equations to eliminate one variable and solve for the other.
In the example 'x + 3y = 7' and 'x - 6y = -11', why is the variable 'x' eliminated?
-The variable 'x' is eliminated because its coefficient is the same in both equations (1), allowing us to subtract the equations and eliminate 'x'. This simplifies the system to one equation in 'y'.
How do you find the value of 'y' after eliminating 'x' in the example above?
-After eliminating 'x', you get the equation '9y = 18'. Dividing both sides by 9 gives 'y = 2'.
In the second example, 'x - y = 2' and '2x + 3y = 19', how do you decide which variable to eliminate?
-You choose to eliminate the variable 'y' by making the coefficients of 'y' in both equations equal. In this case, you multiply the first equation by 3, making the coefficient of 'y' in both equations 3.
What happens after multiplying the first equation by 3 in the second example?
-After multiplying the first equation by 3, the system becomes '3x - 3y = 6' and '2x + 3y = 19'. Adding these equations eliminates 'y' and results in '5x = 25', so 'x = 5'.
How do you find the value of 'y' once 'x' is known?
-Once 'x' is found, substitute it into one of the original equations to solve for 'y'. For example, substituting 'x = 5' into 'x - y = 2' gives '5 - y = 2', so 'y = 3'.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video

memahami konsep persamaan linear satu variabel

SISTEM PERSAMAAN LINIER TIGA VARIABEL

PERSAMAAN LINEAR DUA VARIABEL (PLDV) KELAS 9
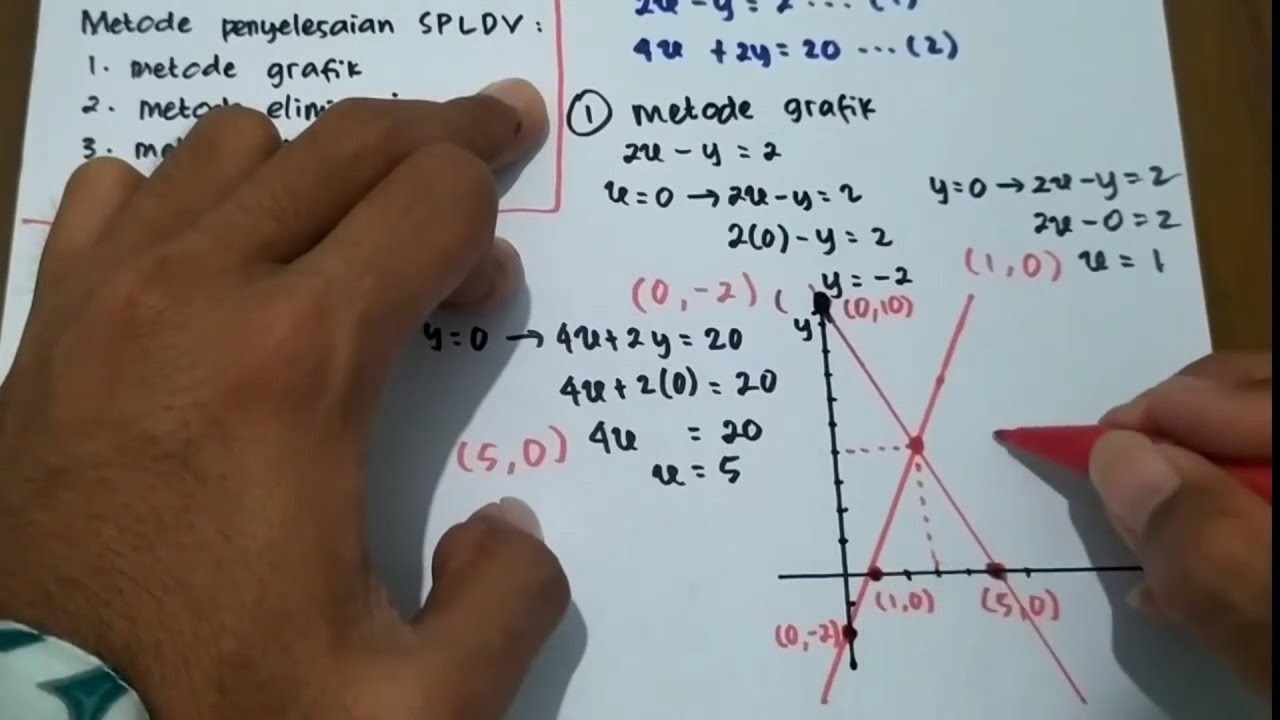
Sistem persamaan linear dua variabel kelas 10 - metode grafik

Penyelesaian Sistem Persamaan Linear Dua Variabel (SPLDV) dengan Metode Grafik.

Kurikulum Merdeka Matematika Kelas 9 Bab 1 Sistem Persamaan Linear Dua Variabel
5.0 / 5 (0 votes)