[PART 11] Matematik Tingkatan 5 BAB 2 KSSM | Matriks | kaedah matriks persamaan linear serentak
Summary
TLDRIn this video, the process of solving simultaneous linear equations using matrix methods is explained in detail. The method involves converting the equations into matrix form and solving for the unknowns by applying matrix operations, particularly using matrix inverses. Practical examples guide viewers step-by-step on how to handle matrices, including the conversion of linear equations to matrix form and the subsequent solution. Emphasis is placed on accuracy in setting up the matrix and calculating the inverse. The video provides clear instructions and examples, ensuring viewers can confidently apply the matrix method for solving linear equations.
Takeaways
- 😀 The video discusses solving simultaneous linear equations using matrix methods.
- 😀 It emphasizes the importance of transforming linear equations into matrix form before applying matrix techniques.
- 😀 The first step is to ensure the arrangement of variables and constants is consistent for proper matrix formulation.
- 😀 Matrices A, X, and B represent coefficients, variables, and constants respectively in the matrix equation.
- 😀 The matrix equation can be solved by finding the inverse of the coefficient matrix (A) and applying it to the constants matrix (B).
- 😀 Matrix operations, like multiplication with the inverse matrix, are used to solve for the variables X and W.
- 😀 The video demonstrates an example where a matrix equation is solved by inverting the matrix and performing multiplication.
- 😀 When performing matrix operations, special care is needed to correctly handle negative signs and constants.
- 😀 The importance of accuracy in matrix manipulation is highlighted to avoid errors in solving the system of equations.
- 😀 The video concludes by showing how to express the solution clearly, specifying the values of X and W, instead of leaving them in matrix form.
Q & A
What is the main topic of the video?
-The main topic of the video is solving simultaneous linear equations using matrices.
What are the steps to solve simultaneous linear equations using matrices?
-The steps involve converting the system of linear equations into matrix form, then multiplying both sides of the equation by the inverse of the coefficient matrix to solve for the variable matrix.
What does the matrix equation Ax = B represent?
-In the equation Ax = B, A is the coefficient matrix, x is the matrix of variables, and B is the constants matrix.
Why is it important to ensure the variables are placed correctly in matrix form?
-It is crucial to maintain the correct order and alignment of variables in matrix form to avoid mistakes when solving the equations.
What is the role of the inverse matrix in solving simultaneous equations?
-The inverse matrix of the coefficient matrix A is used to isolate the variable matrix x, allowing us to solve for the variables by multiplying both sides of the equation by A's inverse.
In Example 28, how do you calculate the solution for X and W?
-In Example 28, the solution for X and W is found by calculating the inverse of the coefficient matrix, then multiplying it with the constants matrix B to obtain the values for X and W.
What is the first step in converting a system of linear equations into matrix form?
-The first step is to write the system of linear equations in the form where the variables are aligned, and the coefficients are clearly identified so they can be placed into the coefficient matrix.
What does the term 'matrix inverse' refer to?
-The matrix inverse refers to a matrix that, when multiplied by the original matrix, yields the identity matrix. It is used to solve for the variable matrix in linear equations.
Why is it important to check the signs and coefficients when converting to matrix form?
-It is important to check the signs and coefficients to ensure accuracy in the matrix representation. Incorrect signs or coefficients will lead to incorrect results when solving the equations.
What should the final answer represent when solving linear equations using matrices?
-The final answer should represent the specific values of the variables, not the matrix form. The variables should be expressed as numerical solutions, such as X = 2 and W = 1/2.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video

Matrices (cayley Hamiton Theorem) IN HINDI Part No 04 As Per New Syllabus (N O C )

Linear equations Gaussian Methods - English

METODE NUMERIK P2 | METODE GAUSS UNTUK MENYELESAIKAN SPL
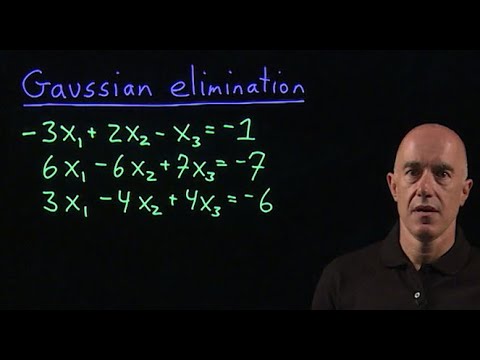
Gaussian elimination | Lecture 10 | Matrix Algebra for Engineers
Determinan part 2
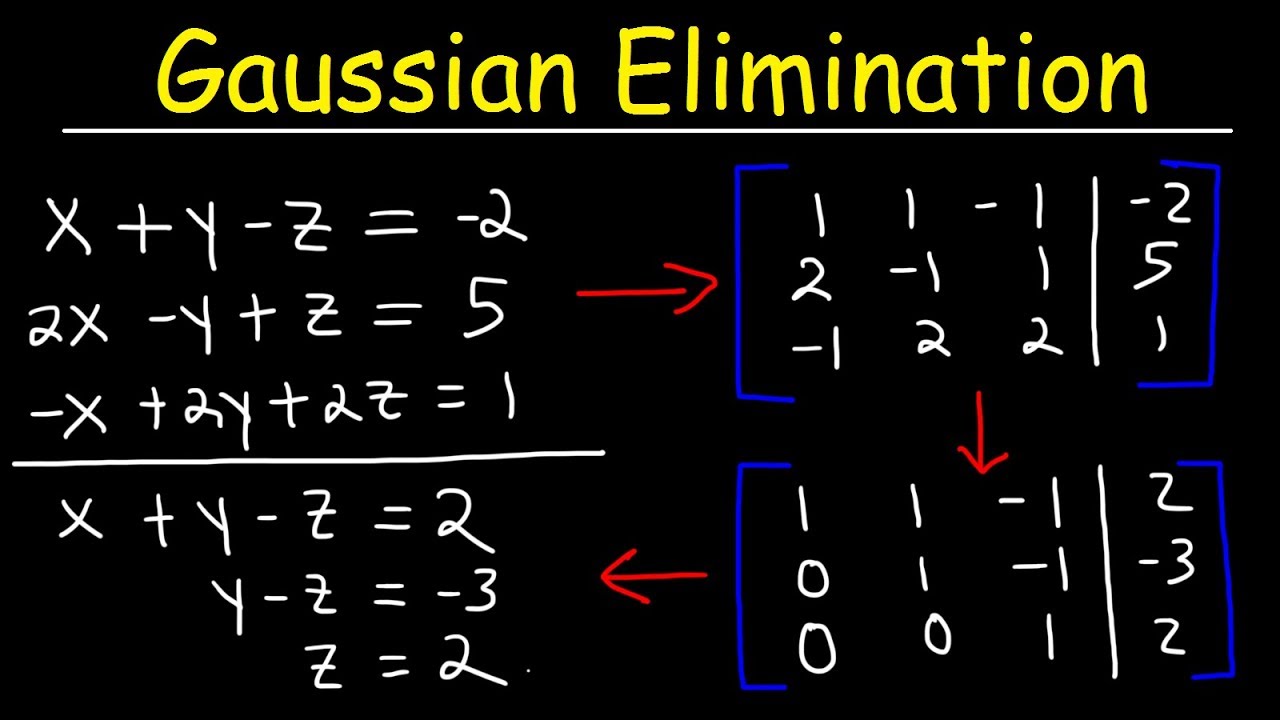
Gaussian Elimination & Row Echelon Form
5.0 / 5 (0 votes)