BELAJAR INTEGRAL DARI DASAR DALAM 12 MENIT!

Summary

In this video, the concept of integrals is explained, focusing on their role as the reverse of derivatives, known as anti-derivatives. It covers the basic integration formulas and properties, such as handling constants, sum/difference of functions, and definite integrals. Examples illustrate how to apply integration rules to various functions, including polynomials and constants. The video emphasizes the importance of understanding integral properties for solving problems in calculus, particularly for preparing for tests. It also hints at more advanced integration techniques, like substitution and integration by parts, which will be explored in future content.

Takeaways

• 😀 Integral is the reverse of differentiation, also called an anti-derivative.
• 😀 It is essential to understand differentiation first, as it directly relates to integral calculations.
• 😀 The general rule for differentiation of powers is: if y = x^n, then the derivative is n * x^(n-1).
• 😀 To integrate, the exponent is increased by 1, and then divided by the new exponent.
• 😀 The constant C is added in integration since the original constant from the function is lost during differentiation.
• 😀 The indefinite integral formula for x^n is: (1/(n+1)) * x^(n+1) + C.
• 😀 When performing integrals, terms with addition or subtraction can be handled separately.
• 😀 Constants outside of the function can be treated as multipliers during integration.
• 😀 For integrals with specific bounds (definite integrals), the constant C is not included in the result.
• 😀 Integral properties, such as the fact that an integral with identical upper and lower bounds equals zero, are important to understand for problem-solving.
• 😀 Techniques like substitution or integration by parts may be required for more complex integrals, and these will be discussed in future videos.

Q & A

What is the relationship between differentiation and integration in the context of calculus?

Differentiation and integration are inversely related. Differentiation refers to finding the rate of change (or derivative) of a function, while integration is the process of finding the original function from its derivative, essentially 'undoing' the process of differentiation.

Why is it important to understand differentiation before learning about integration?

Understanding differentiation is crucial because integration is essentially the reverse process. If you don't have a solid grasp of how to differentiate functions, it will be difficult to understand how to retrieve the original function through integration.

What is an antiderivative in the context of integration?

An antiderivative is the original function that, when differentiated, gives the function being integrated. In other words, integration is the process of finding an antiderivative.

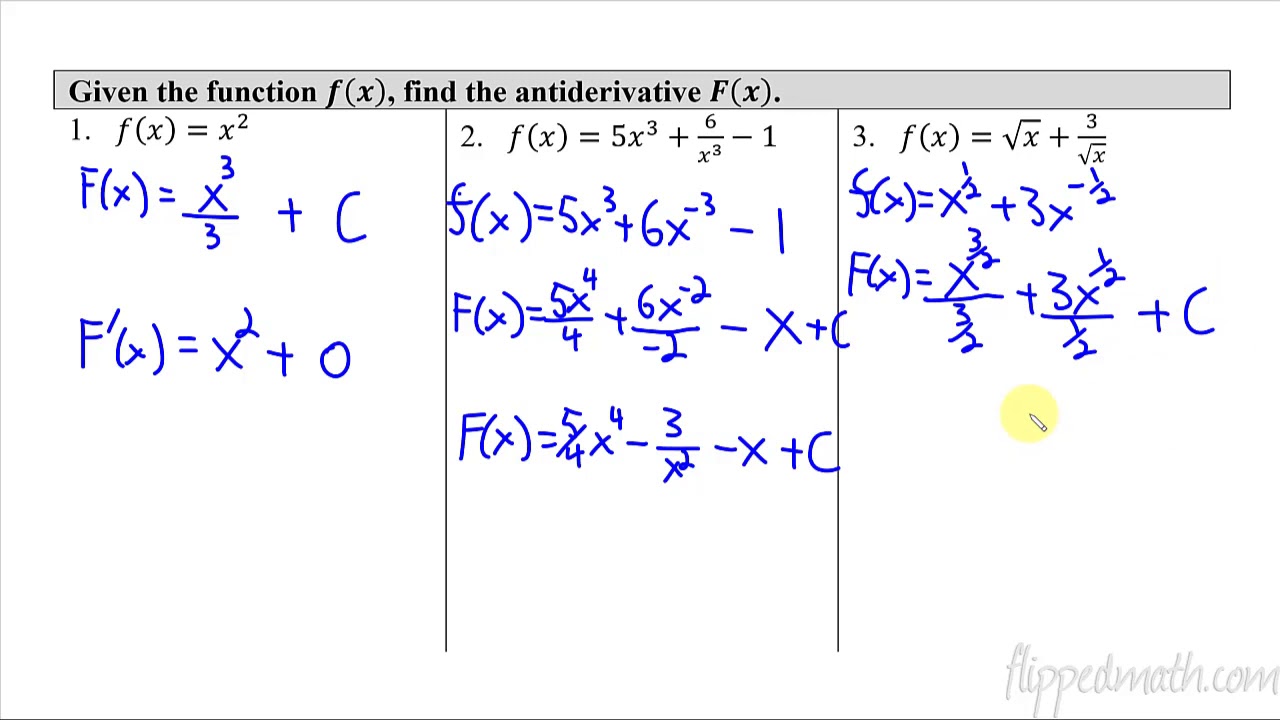
How do you integrate a power function, like x^n?

To integrate a power function x^n, you increase the exponent by 1 and divide by the new exponent. The general formula for this is: ∫x^n dx = (x^(n+1))/(n+1) + C, where C is a constant of integration.

What happens when we integrate a function with a constant, such as 5?

When integrating a constant, the result is the constant multiplied by x, i.e., ∫K dx = Kx + C, where K is a constant and C is the constant of integration.

What is an indefinite integral?

An indefinite integral is the process of finding an antiderivative of a function without specific limits of integration. The result includes an arbitrary constant 'C', which represents any possible constant that could have been part of the original function.

What is the difference between an indefinite and a definite integral?

An indefinite integral does not have upper or lower limits and includes a constant of integration (C), while a definite integral has specific limits (a and b) and calculates the exact area or accumulation between those limits without a constant of integration.

How do you compute the value of a definite integral?

To compute the value of a definite integral, you integrate the function as usual, then substitute the upper and lower limits into the resulting antiderivative and subtract the values: ∫[a to b] f(x) dx = F(b) - F(a), where F(x) is the antiderivative of f(x).

What does it mean if the integral of a function from a to a equals 0?

If the integral of a function from a to a equals 0, it means that the area under the curve is zero, which typically occurs when the function has no net change or the limits of integration are the same.

What are the basic properties of integrals that should be understood?

Some basic properties of integrals include: 1) The integral of a sum of functions is the sum of their integrals, 2) Constants can be factored out of an integral, 3) The integral of a constant is simply the constant multiplied by x, and 4) Changing the limits of integration inverts the sign of the result.