ATPL General Navigation - Class 4: Convergency.

Summary

In this educational video, Grant explores the concept of great circles, the shortest paths between two points on the Earth's surface, and their constantly changing direction. He explains how the convergence of meridians, which depends on latitude, affects the great circle track using simple geometry and the sine function. The video demonstrates how to calculate the change in direction, known as convergence, and how to switch between great circle and rhumb line tracks. It also clarifies the differences and similarities between the two, including the fact that they only match at the midpoint of a journey and that the rhumb line is always closer to the equator.

Takeaways

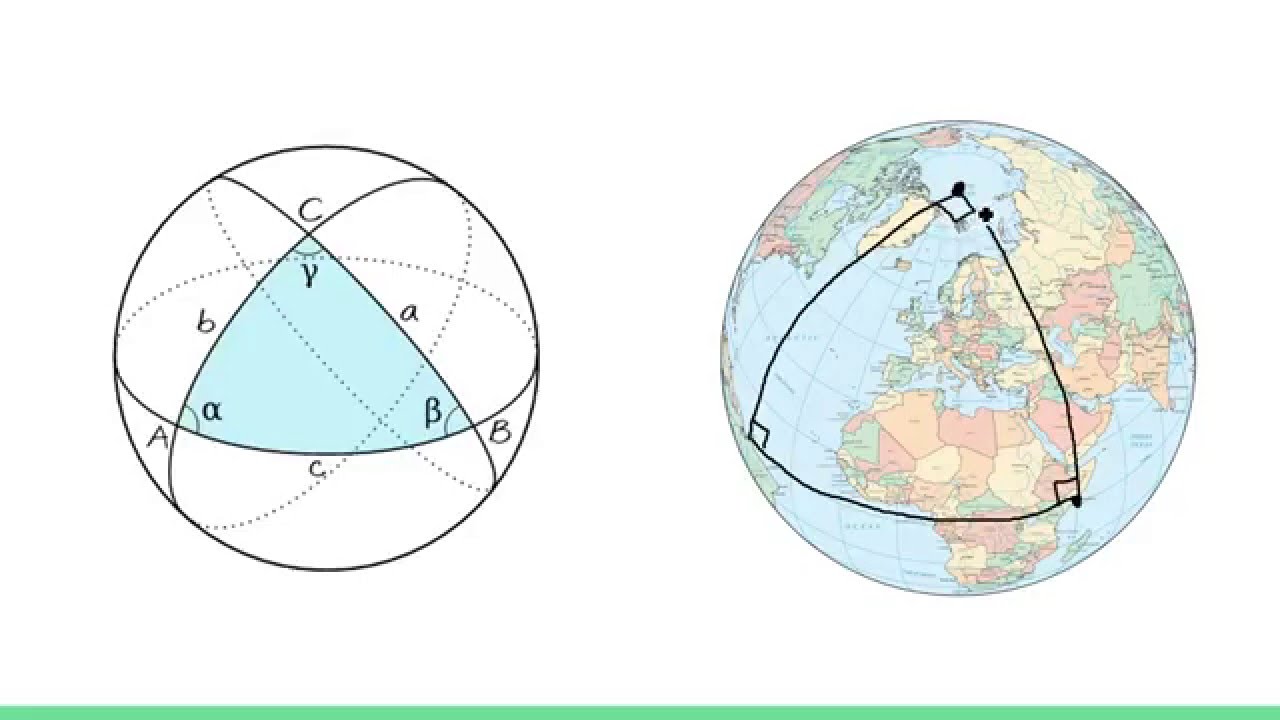
• 🌐 A great circle is the shortest possible distance between two points on Earth, and its direction changes as you travel along it.
• 📐 The direction of a great circle can be determined by simple geometry, comparing angles at different points along the path.
• 🌍 The change in direction of a great circle is influenced by the convergence of lines of longitude, which varies with latitude.
• 📈 Convergence is zero at the equator and increases towards the poles, and it can be calculated using the sine function of the latitude times the change in longitude.
• 🧭 The great circle bearing can be calculated by adding the convergence to the original angle at the starting point.
• 🔢 The average great circle track can be found by averaging the bearings at two points, which is equivalent to the rhumb line track between them.
• 🌟 The rhumb line is a path with a constant direction, which is always closer to the equator than the great circle path.
• 🔄 The highest latitude of a great circle route is reached when the great circle track is at 90 or 270 degrees, indicating purely east or west travel.
• ⚖️ The conversion angle, which is half the convergence, is used to switch between a great circle and a rhumb line.
• 🛰️ The relative position of 'north' changes as you move along a great circle, which is why the direction is constantly changing.
• 📊 The sine function is key to understanding convergence, as it provides a mathematical model that starts at 0 at the equator and reaches 1 at the poles.

Q & A

What is a great circle and why does its direction constantly change?

A great circle is the shortest possible path between two points on the surface of the Earth. Its direction constantly changes because as you move along the circle, the relative position of 'north' changes, necessitating a continuous adjustment in direction to maintain the shortest path.

How can the angle difference at two points on a great circle be determined?

The angle difference at two points on a great circle can be determined using simple geometry by creating parallel lines and comparing the angles at each point. The angle at point B is found by adding the angle at point A to the change in longitude and the convergence angle.

What is convergence and how does it relate to the change in direction of a great circle?

Convergence is the angle by which the direction of a great circle changes as it moves from one meridian to another. It depends on the latitude and the change in longitude between two points, affecting how much the direction of the great circle needs to adjust.

Why is the sine function used to calculate convergence?

The sine function is used to calculate convergence because it provides a value that starts at 0 at the equator and increases to 1 at the poles, which corresponds to the change in convergence from 0 degrees at the equator to full convergence at the poles.

How is the average great circle track calculated?

The average great circle track is calculated by taking the average of the initial and final great circle bearings. This average is equivalent to the rhumb line track between the two points.

What is a rhumb line and how does it differ from a great circle?

A rhumb line is a path that crosses all meridians at a constant angle, maintaining a constant direction from start to finish. It differs from a great circle, which changes direction as it follows the shortest path between two points.

At what point are the great circle track and the rhumb line track the same?

The great circle track and the rhumb line track are the same at the midpoint between two longitudes, where the lines are parallel.

What is the conversion angle and how is it related to convergence?

The conversion angle is the difference between the rhumb line track and the great circle track. It is equal to 0.5 times the convergence, providing a way to convert between the two types of tracks.

How does the latitude affect the convergence of lines of longitude?

The latitude affects the convergence of lines of longitude because the lines converge more as you move from the equator towards the poles. At the equator, there is zero convergence, while at the poles, there is full convergence.

What is the relationship between the great circle track and the change in longitude at the poles?

At the poles, the convergence is equal to the change in longitude. This means that the angle of the great circle track at the poles is determined solely by the change in longitude between the two points.

Outlines

00:00

🌐 Understanding Great Circle Navigation

The script introduces the concept of a great circle, which is the shortest path between two points on the Earth's surface, and its constantly changing direction. The presenter, Grant, explains how to determine the change in direction using a top-down diagram of the Earth centered at the North Pole. He illustrates that the angle between meridians changes as one moves away from the equator towards the poles. By using simple geometry, the script demonstrates how to calculate the difference in angle at two points on a great circle route. The explanation includes creating additional lines for clarity and using the z-angle rules to establish that the change in longitude plus the original angle gives the new great circle direction. The concept of convergence, which depends on latitude, is introduced, with the sine function proposed as a way to calculate it. The script concludes with a theoretical equation for convergence and an example to test the theory.

05:04

📐 Calculating Convergency and Great Circle Bearings

This paragraph delves into the specifics of calculating the convergency and great circle bearings. It begins with an example where the great circle bearing from point A to point B is given, and the task is to find the new bearing at point B. The script explains the use of the sine function to calculate convergency based on latitude and change in longitude. An example calculation is performed, resulting in a convergency of 25 degrees, which is then used to adjust the original bearing to find the new great circle bearing at point B, which is 65 degrees true. The concept of the average great circle track is introduced, which is equivalent to the rhumb line between two points. The script also explains the relationship between the great circle track and the rhumb line, noting that they are equal at the midpoint between two longitudes, and that the rhumb line always curves towards the equator. The paragraph concludes with an explanation of the conversion angle, which is half of the convergency and represents the difference between the rhumb line and the great circle track.

10:05

🛫 Great Circle Tracks and Convergency at Different Latitudes

The script continues with an example of an aircraft following a great circle track from point A to point B, and the task is to determine the great circle track on departure from A. The explanation involves drawing a rhumb line and understanding that it will be at a 90-degree angle to the change in longitude since the track is from west to east. The convergency is calculated using the sine of the latitude (45 degrees) and the change in longitude (60 degrees), resulting in a convergency of approximately 42 degrees. The conversion angle, which is half of the convergency, is then used to adjust the rhumb line to find the great circle track. The script emphasizes that great circle tracks are constantly changing due to the changing relative position of north, and at the poles, convergency equals the change in longitude. At the equator, there is no convergency since the lines of longitude are parallel. The sine function is used to calculate convergency, which is essential for determining the conversion angle. The paragraph concludes by summarizing the differences between rhumb lines and great circle tracks, noting that they only coincide at the midpoint between two points and that the average track of the great circle is equal to the rhumb line track.

Keywords

💡Great Circle

A great circle is a circle on the surface of a sphere that has the same center as the sphere itself. In the context of the video, it refers to the shortest possible path between two points on the Earth's surface, which is a segment of a great circle. The video discusses how the direction of this path constantly changes, emphasizing its significance in navigation and the importance of understanding its properties for accurate tracking.

💡Track Direction

Track direction refers to the path or course taken by a moving object, such as a ship or an aircraft, relative to a fixed point, typically north. The script explains that for a great circle, the track direction is not constant but changes as one moves across the Earth's surface, which is a fundamental concept in understanding how great circles function in navigation.

💡Convergence

Convergence in the script pertains to the coming together of lines of longitude towards the poles. It is a measure of how much the direction of a great circle route changes as one travels along it. The video uses the sine function of latitude to calculate convergence, which is crucial for determining the actual path of a great circle route.

💡Latitude

Latitude is the angular distance of a place north or south of the Earth's equator, expressed in degrees. In the video, latitude is used to calculate the convergence of great circle routes. The sine of the latitude is a key factor in the formula for determining convergence, with the value changing from 0 at the equator to 1 at the poles.

💡Longitude

Longitude is the angular distance of a place east or west of a reference meridian to another meridian. The script discusses how changes in longitude, in conjunction with latitude, affect the convergence and thus the direction of a great circle route. The change in longitude is a critical component in the formula for calculating convergence.

💡Run Line

A run line, also known as a rhumb line, is a path on the Earth's surface that crosses all meridians at the same angle, maintaining a constant bearing. The video contrasts the run line with the great circle, explaining that while a run line has a constant track direction, a great circle's direction changes due to the Earth's curvature.

💡Convergency Equation

The convergency equation is a mathematical formula used to calculate how much the lines of longitude converge towards the poles. The script presents the equation as 'sine of latitude times the change in longitude equals the convergence', which is essential for determining the change in direction for a great circle route.

💡Conversion Angle

The conversion angle is the difference between the track direction of a run line and a great circle. The script explains that this angle is always half of the convergence, and it is used to convert between a great circle track and a run line track, which is essential for navigational calculations.

💡Equator

The equator is an imaginary line that divides the Earth into the Northern and Southern Hemispheres. In the script, the equator is mentioned as a point where there is zero convergence because lines of longitude are parallel to each other at this line, which simplifies navigational calculations.

💡Poles

The poles, specifically the North and South Poles, are the two points where the Earth's axis of rotation intersects its surface. The video script discusses how convergence is at its maximum at the poles, with the convergence being equal to the change in longitude, which is a critical concept for understanding the behavior of great circles at extreme latitudes.

💡Sine Function

The sine function is a mathematical function that relates the ratio of the length of the opposite side to the length of the hypotenuse in a right-angled triangle. In the context of the video, the sine function is used to model the variation of convergence with latitude, starting at 0 at the equator and reaching 1 at the poles, which is essential for the convergency equation.

Highlights

A great circle is the shortest possible distance between two points on the Earth's surface.

The direction of a great circle route constantly changes due to the curvature of the Earth.

The angle difference at two points on a great circle can be determined using simple geometry.

Convergency of lines of longitude depends on latitude, with zero convergence at the equator and full convergence at the poles.

The sine function is used to calculate the convergency based on latitude and change in longitude.

Convergency equals zero at the equator and equals the change in longitude at the poles.

An example demonstrates calculating the new great circle bearing at point B using convergency.

The average great circle track can be found by averaging the bearings at two points.

The run line track is equivalent to the average great circle track at the midpoint between two longitudes.

The run line always curves towards the equator, unlike the great circle.

The highest latitude or vertex of a great circle is reached at tracks of 90 or 270 degrees.

The conversion angle, which converts from a great circle to a run line, is equal to 0.5 times the convergency.

An aircraft's great circle track on departure can be calculated using the run line and convergency.

Convergency at the poles is equal to the change in longitude, affecting the great circle track.

At the equator, there is no convergency because lines of longitude are parallel.

The run line follows a constant track, while the great circle track is constantly changing.

The great circle and run line tracks are the same at the mid-longitude between two points.

The difference between the great circle and run line tracks is the conversion angle.

Transcripts

a great circle is a line across the

earth with a constantly changing track

direction but by how much does it change

let's find out

[Music]

hi i'm grant and welcome to the fourth

class in the gnab series today we're

going to be taking a look at the

constantly changing track of great

circles and then also how to switch

between a great circle and a run line

so we established in the previous class

that a great circle is the shortest

possible distance between two points but

the direction is constantly changing

if we look at a top-down diagram right

at the north pole you can see that if we

move from

this meridian here

the angle is quite small to this

meridian here the angle is clearly

larger

so if we take point a and point b on

this map and we think of the angle at

point a we can find out the difference

in angle at point b

using some simple geometry

so if we take a line parallel

to

point a

and pop it at point b

we can see that this angle in here let's

call it angle

um

x

is equal to this angle in here x

so we basically have to find the

difference that is added on

in this angle

and how do we do that

well we just create some

more lines to help us

so if we come

in here

and we take a parallel line going up we

can see that this angle in here

let's call that y degrees

using simple z-angle rules we can see

that this angle in here

would also be equal to y

and what is the difference between this

point and this point

or this line and this line

we're on different lines of

longitude so it's going to be the change

in longitude

plus our original angle is going to give

us our new great circle direction and

that's true at all points along here

so you can see even at this

midway point i've created this angle in

here is going to be x and then this

small angle in here let's call it z

and the reflective z angles

gives you

the change in longitude plus the

original angle

so as lines of longitude are curved they

go from being perfectly parallel at the

equator

all the way up to a single point at the

poles

this means that the convergency of the

lines of longitude must depend

on how close we are to either of these

points

so there's zero convergence at the

equator and there'd be full convergency

up at the poles

basically it must depend on the latitude

that we are at

so if we think about the equator as

being zero degrees and the poles as

being 90 degrees

we're looking for some sort of function

that gives us zero at zero and the full

convergency at 90.

so we have a function for that and that

is the sine function

so we can come up for a theory

and an equation for how much lines

converge

based on this

sine wave

so if we say

sine of the latitude

times by the change in longitude

then we should get the convergency

and let's just test that theory

so we know that we have zero convergency

at the equator so sine of zero

times whatever the change in longitude

is doesn't matter

is going to be

zero because sine zero is zero

so

convergency

equals zero

parallel lines makes sense

then we'll go all the way up to

sine of 90

times by the change in longitude

sine of 90 is one

and that's what we saw in the previous

example of that top down view at the

north pole we know that the convergence

of those lines is

the change in latitude between them

so there you go we've got a theory and

an equation for convergency

let's take a look at a wee example of

how we would use that

so here we go the great circle bearing

of b from position a

is 0 4 0 degrees true what is the new

great circle bearing at b

so first things first we draw the effing

picture

so we have point b

which is over here north 30

east 60 and point a is further sorry

point a is less east than point b so

point a is going to be over here and

it's at the same uh north 30.

we have north up this direction

and north up this direction

and we have a great circle going between

both of them

we have this angle in here as being 0 4

0 degrees

and we're looking for this angle in here

cool now we've got it all down on the

page

let's just use our equation and figure

this out so the convergency

equals sine lat

times the change in the longitude

so sine of the latitude is 30 sine 30

degrees

times with the change in longitude east

10 to e60 that's going to be 50.

sine 30 is a half times 50. that means

our convergency is going to be 25

degrees

and from our picture we can clearly see

that b

is a bigger angle

than the angle at point a

so we have to add this

onto our original angle

for an answer the great circle bearing

at b

is going to be 4a plus 25 is going to be

65 degrees true

and if we were to take an average of

our zero four zero and our zero six five

we'd find the average great circle track

so in this case it would be

52 and a half for the average great

circle track if it asked us that it's

very simple take one take the other and

divide by two

and that average great circle track

would be equivalent to the rum line

between the two of them

so when i draw a diagram of both the

great circle track from one point to

other and the rum line on it we can see

a few points

first at the midpoint between these two

longitudes

the rum line track and the great circle

track are equal because the lines are

parallel

second the rum line is always closer to

the equator

this case we're looking at the south

pole north is still up there the equator

is going to be up here somewhere and the

rum line curves towards the equator

always

and the third point

is that the highest

latitude or the vertex of the great

circle

is reached at the point where the great

circle track is 90 or 270 degrees we're

going either purely east or purely west

and the very final point is you can see

there's clearly a difference between

our starting

rum line track

so our starting great circle track and

our starting

rum line track

and the difference is this angle in here

this angle in here is known as the

conversion angle

because it converts us from a great

circle to a run line

and the conversion angle

is equal to

0.5 the convergency

so if we know the great circle track we

can work out the run line and vice versa

if we know the convergency and the

conversion angle

the conversion angle is always 0.5 times

the convergency but convergency the

example we saw

um our equation for sine lap times

change of long equals the convergency

isn't always true it depends on what

type of chart we're using but for now

think of this as sine

latitude times the change in longitude

and conversion angle is 0.5 conversion

angle always being half of the

convergency always

so let's take a look at another example

an aircraft follows a great circle track

from a to b

what is the great circle track on

departure from a

so let's draw the effing picture

great circle track from a to b what is

the great circle track on the part from

a

point a is west 50 point b is east 10.

so a is going to be over here

at

uh south 45

west 50 degrees

and this is on the same

latitude

south 45

and it's east 10.

if these were different latitudes and

sort of angled like this

to find the convergence you would take

the average of the two

but this is not the case for this one

but we are going to cross over the

equator right here

so we have south poles going to be down

here

north going up in two directions like

this

and we know that we're looking for the

great circle which is the straight line

between the two

and yeah that's all the information we

have

and it initially looks like we don't

have enough information to find out the

great circle track

in here this is what we're looking for

but we do because we know

that if we follow a line of latitude

that's a rum line

so we can draw on our rum line

roughly like that

and we know that if we're following a

line of latitude it's going to be either

90 degrees or 270 degrees

in this case we're going from point a

which is west to the east

so it's going to be 90 degrees

this angle in here

is going to be 90 degrees between this

rum line

and then we can find out the conversion

angle in here add that onto our great

circle

sorry add that onto our run line to find

the value for the great circle

so let's pop in

our

equation

sine lat

times the change in longitude

is the convergency

so sine 45

multiplied by the change in longitude

from west 50 to east 10 we're passing

through the equator at

sorry the

greenwich meridian so we've got to add

them two together

so it's 60 degree change

and sine 45 times 60 745 is about 70

percent

so we'll say that convergency is 42

degrees

and our conversion angle

which is our difference between the

runway and the great circle

is going to be half of that 21 degrees

okay and then if we look at the picture

we can clearly see that the great circle

is going to be bigger than the rum line

so our value for

x our great circle track

is going to be 90

plus the 21

or

111 degrees

in summary then great circle tracks are

constantly changing because we're

constantly referencing everything to

north and as we move the relative

position of north to us

changes like this

the

at the poles the convergency is equal to

the change in longitude you can see that

the convergency of these lines

means that we get an angle in here

which is equal to the change in

longitude and then we add that onto our

original

uh track

and that would give us our new track for

that great circle at this second point

so convergency at the poles is

the change in longitude but then at the

equator the lines are perfectly parallel

so there is no convergency

and we use the sine function because

that starts at 0 at 0 degrees and goes

up to 1

at 90 degrees

to give us a

equation for the convergency which is

convergency is the sign of the latitude

times the change in longitude

convergency

is very useful for getting the

conversion angle

which is the difference between the rum

line and the great circle

and it is 0.5 times the convergency here

some other points about the great circle

and rum line

the rum line follows a constant track

from point a to point b

and the great circle track is constantly

changing

the only point that these two values for

track will be the same is at the mid

longitude between two points

so you can say that the

average track of the great circle is

equal to the run line track

and as we stated before the difference

between the two will be the conversion

angle