Solusi Persamaan Diferensial dengan Transformasi Laplace | Laplace Transform | Different Equation

Summary

In this video, the host explains how to solve a differential equation using Laplace transforms and partial fraction decomposition. The equation involves a second-order derivative and a cosine function. The process includes transforming the equation using Laplace’s formula, simplifying it, and applying partial fraction decomposition to break it into simpler components. The host emphasizes understanding the transformation steps, referencing tables, and substituting known values. The explanation is thorough, detailing each step clearly, offering viewers a methodical approach to mastering Laplace transforms in physics and mathematics.

Takeaways

• 😀 The video covers the use of Laplace transforms and partial fractions to solve a differential equation in physics and mathematics.
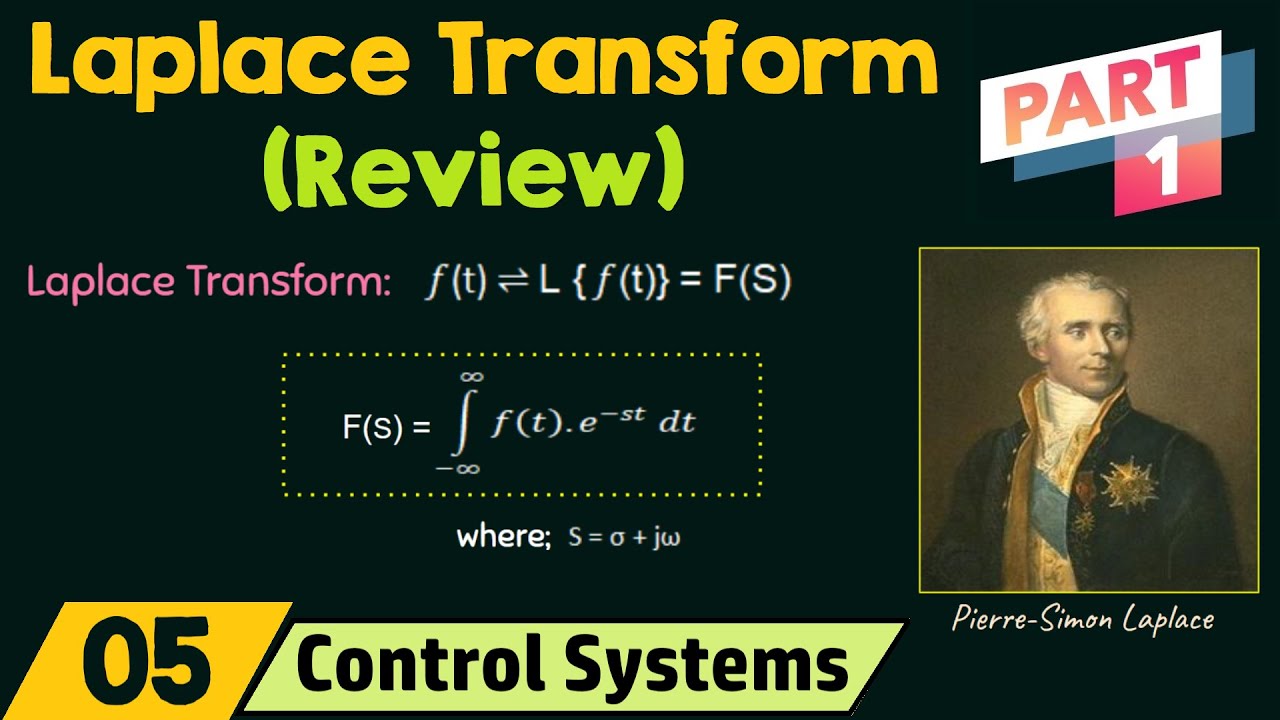
• 😀 The example equation provided is a second-order differential equation: y'' - 2y' + y = 2 cos(θ).
• 😀 Laplace transformation is applied to convert derivatives into algebraic expressions using the Laplace operator, 'P'.
• 😀 The Laplace transform of the second derivative y'' is written as P²Y - P * y(0) - y'(0), where 'P' is the Laplace variable.
• 😀 The transformation of the cosine function 2 cos(θ) involves using standard Laplace transform tables, where cos(θ) is converted into a rational expression.
• 😀 After applying the Laplace transform to both sides of the equation, the next step is isolating Y and simplifying the equation using algebraic manipulation.
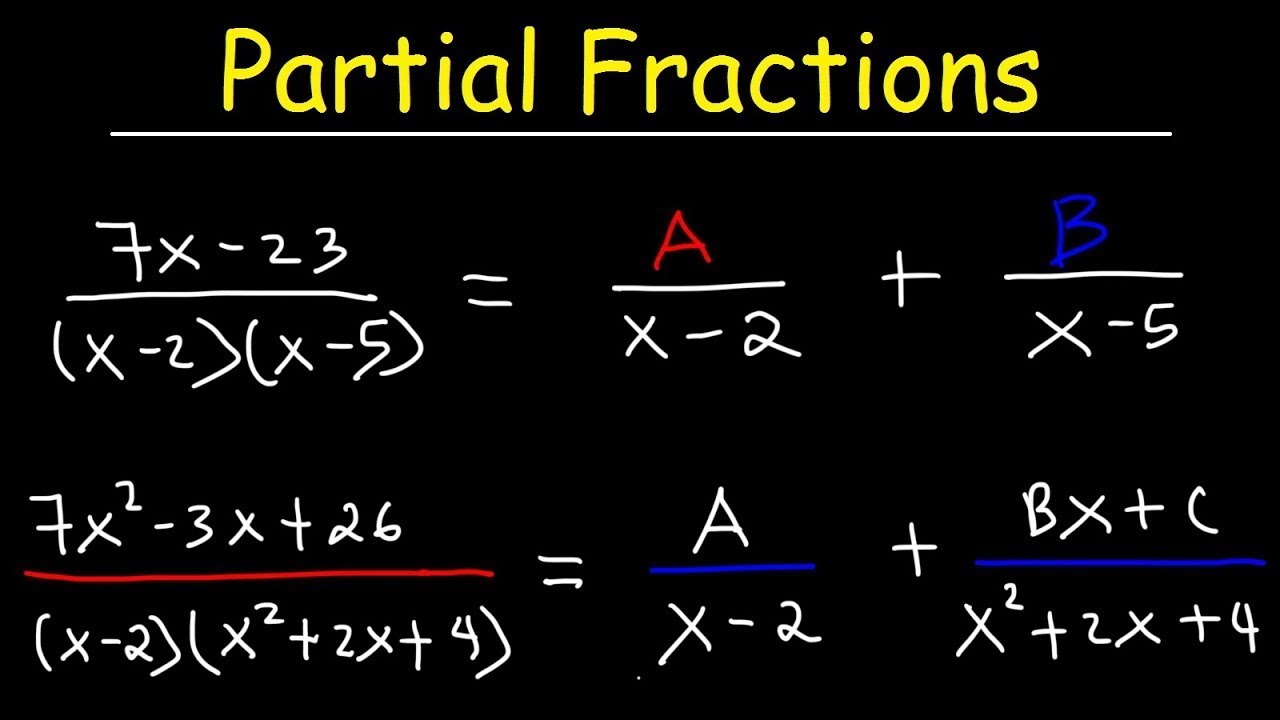
• 😀 Partial fraction decomposition is introduced to simplify complex rational expressions, making it easier to solve for the unknown coefficients.
• 😀 A system of linear equations is solved to determine the values of constants in the partial fraction expansion.
• 😀 Once the coefficients are determined, the inverse Laplace transform is applied to return the solution to the time domain.
• 😀 The video emphasizes the importance of carefully analyzing and simplifying the expressions step-by-step to correctly apply Laplace and partial fractions techniques.
• 😀 The final solution is obtained by combining the results from the Laplace transform and inverse Laplace transform processes, yielding the solution to the original differential equation.

Q & A

What is the main topic of the video?

The video explains how to solve differential equations using Laplace transforms and partial fractions, focusing on an example from a university physics mathematics exam.

What is the general method used for solving the differential equation in the video?

The method involves applying Laplace transforms to the given differential equation, followed by partial fraction decomposition to simplify the equation and find the solution.

How is the second-order derivative (double prime) handled in the Laplace transform?

In the Laplace transform, the second-order derivative (y'') is replaced by the expression P^2Y(s) - P*y(0) - y'(0), where P is the Laplace variable.

What happens to the cosine term in the differential equation when using the Laplace transform?

The cosine term, cos(θ), is transformed using a table of Laplace transforms. In this case, cos(θ) becomes P² / (P² + 1), where P is the Laplace variable.

What is the significance of partial fractions in the solution process?

Partial fractions are used to decompose complex rational expressions into simpler terms, making it easier to inverse Laplace transform and find the solution to the differential equation.

How do you interpret the initial conditions in the Laplace transform?

The initial conditions, such as y(0) and y'(0), are directly substituted into the Laplace-transformed equation to modify the equation and account for the initial values of the function and its derivative.

What is the next step after applying the Laplace transform to the differential equation?

After applying the Laplace transform, the equation is simplified, and the terms involving P are grouped together. Then, partial fraction decomposition is applied to break down the equation further.

How does the speaker handle the equation when dealing with the coefficient of the Laplace variable?

The speaker simplifies the equation by combining like terms and solving for the coefficients, using partial fraction decomposition to handle the complex terms.

What does the speaker mean by 'matching coefficients' in the solution process?

Matching coefficients refers to the step where the speaker compares the coefficients of corresponding powers of P on both sides of the equation and solves for unknown values like A, B, C, and D.

How does the final solution relate to the original differential equation?

The final solution, obtained after applying partial fractions and inverse Laplace transforms, is expressed in terms of the original variable and satisfies the initial differential equation.