283. Integral through partial fractions: repeated quadratic factor
Summary
TLDRIn this video, the presenter demonstrates how to solve an integral using the method of partial fraction decomposition. The integral involves a rational function with a cubic denominator, and the process includes decomposing it into simpler fractions, matching coefficients, and solving for unknown constants. The presenter explains each step in detail, using substitution and trigonometric techniques when necessary. The final result of the integral is presented along with an invitation to explore additional resources on the topic. The video aims to help viewers understand how to apply partial fraction decomposition to solve complex integrals efficiently.
Takeaways
- 😀 The video explains how to solve a complex integral using partial fraction decomposition.
- 😀 The integral involves the expression (2x^3 + 5x^2 + 2x + 3) / (x^3 + 1)^3.
- 😀 The method of partial fractions is used to break down the complex fraction into simpler terms.
- 😀 When dealing with an irreducible quadratic factor in the denominator, the fraction is split into terms with degrees equal to or less than the exponent in the denominator.
- 😀 The coefficients of the partial fractions (A, B, C, D, E, F) are determined by either substituting values for x or equating the coefficients of powers of x.
- 😀 The video demonstrates the process of expanding and simplifying terms to solve for the unknown coefficients.
- 😀 Key steps involve expanding binomials and matching coefficients for each power of x (e.g., x^5, x^4, x^3).
- 😀 Several values are derived from the equation system, such as A = 0, B = 2, C = 0, D = 1, E = 2, and F = 0.
- 😀 Once the coefficients are found, the integral is split into three simpler integrals, which can be solved individually.
- 😀 The integral of the first part is solved using the formula for the arctangent, while the middle integral requires trigonometric substitution for simplification.
- 😀 The result of the integration includes the formula for the arctangent and a simplified rational expression for the remaining term.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video
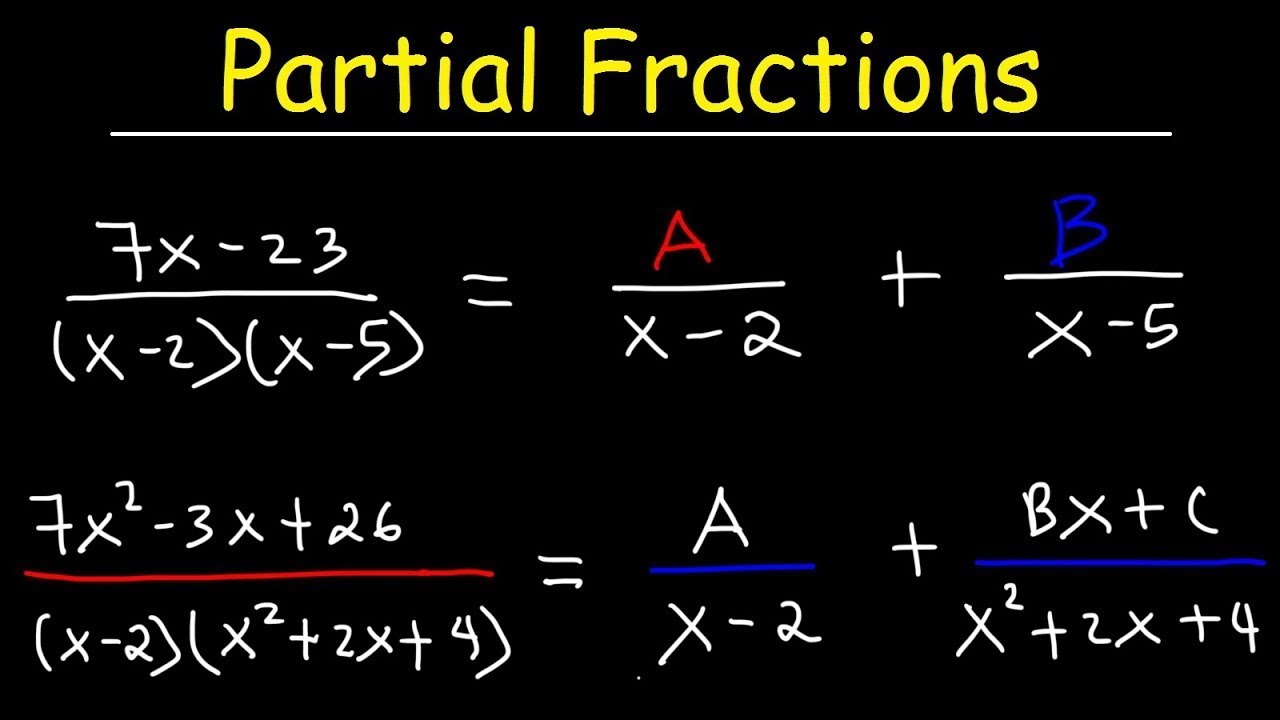
Partial Fraction Decomposion

Solusi Persamaan Diferensial dengan Transformasi Laplace | Laplace Transform | Different Equation

Sistem persamaan linear tiga variabel dengan metode substitusi

Practical use case for fork and pipe in C

KALKULUS | APLIKASI INTEGRAL | VOLUME BENDA PUTAR

Logistic Differential Equation (general solution)
5.0 / 5 (0 votes)