AP Physics 1: Circular Motion 3: Object moving in a horizontal circle
Summary
TLDRIn this video, the presenter walks through a circular motion problem involving a set of keys on a lanyard. The scenario includes spinning the keys at a constant speed of 3 m/s, with a radius of 3 meters and a mass of 0.02 kg. The key objective is to calculate the tension in the rope, which requires analyzing the forces at play, including gravitational force and centripetal force. Through a step-by-step approach, the presenter explains how to determine the tension by applying Newton's second law and solving for both the vertical and horizontal components of the force, ultimately finding the total tension in the system.
Takeaways
- 😀 The problem involves circular motion, where keys are attached to a lanyard and spun with a constant speed of 3 m/s.
- 😀 The system involves a mass of 0.02 kg for the keys and a radius of 3 m for the circular path.
- 😀 The goal of the problem is to find the tension in the string that holds the keys during their circular motion.
- 😀 A force diagram for the keys is drawn, including the weight force (mg) acting downward and the tension force acting along the rope.
- 😀 The weight force is calculated as 0.02 kg * 10 m/s² = 0.2 N, where the downward direction is assigned negative and the upward direction positive.
- 😀 The rope exerts a tension force both in the horizontal and vertical directions, but we focus on the horizontal component that provides centripetal force.
- 😀 There is no acceleration in the vertical direction, meaning the weight force is balanced by the vertical component of the tension force.
- 😀 The keys experience centripetal acceleration, which is responsible for changing their direction and keeping them moving in a circle.
- 😀 The formula for centripetal acceleration is a_c = v² / r, where v is the speed (3 m/s) and r is the radius (3 m), resulting in an acceleration of 3 m/s².
- 😀 Using Newton’s Second Law (F = ma), the net force needed to provide the centripetal acceleration is calculated as F_net = 0.02 kg * 3 m/s² = 0.06 N.
- 😀 The tension in the string is equal to the net force, which in this case is 0.06 N, providing the necessary force to keep the keys moving in a circle.
Please replace the link and try again.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video

PHYS 121 - Week 4 Lecture 2 - Friction & Circular Motion

Fisika SMA - Hukum Newton (6) - Penerapan Hukum Newton Pada Katrol (I)

Movimientos circulares, MCU y MCUA - rápido y fácil
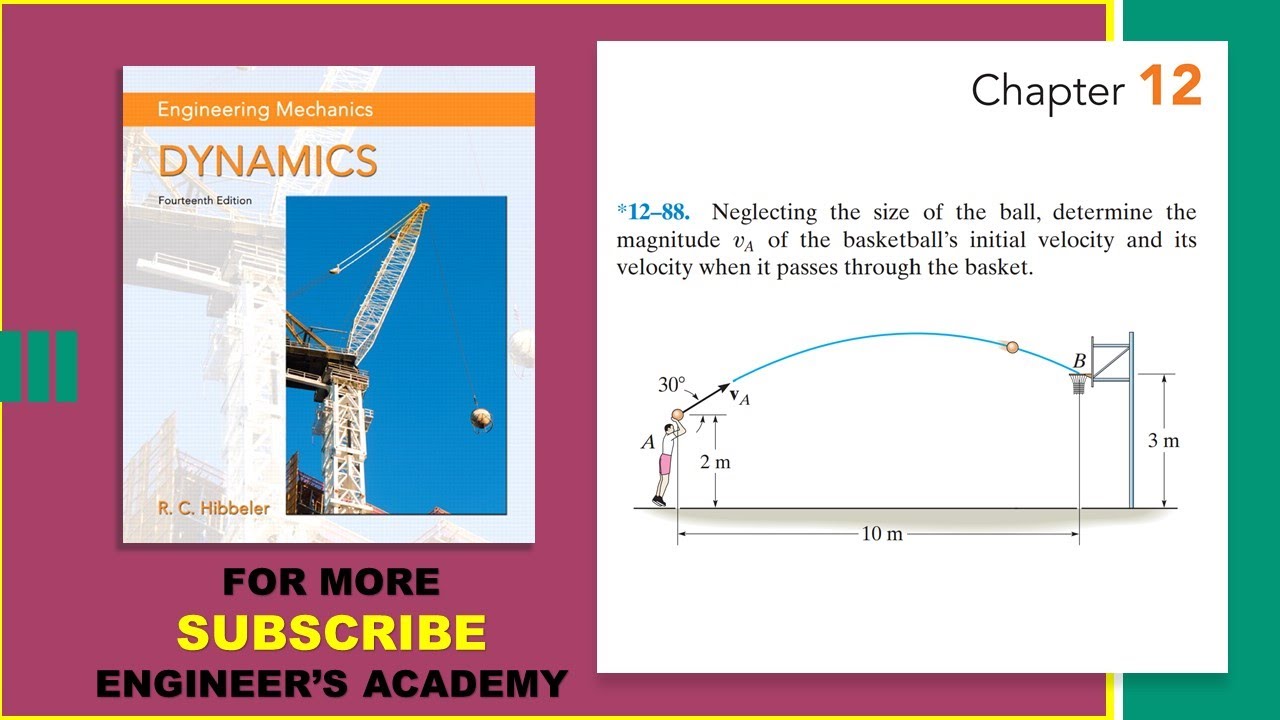
12-88 | Engineering Dynamics Hibbeler 14th Edition | Engineers Academy

The Most Mind-Blowing Aspect of Circular Motion

FREE FALL PHYSICS PERFORMANCE TASK
5.0 / 5 (0 votes)