Fluid Mechanics Lesson 15C: Compressible Flow in Converging-Diverging Ducts

Summary

This lesson on compressible flow and converging-diverging (CD) ducts explores key concepts like the critical role of the throat in achieving supersonic flow, the relationship between Mach number and area ratios, and the behavior of flow in different sections of a CD nozzle. The lesson covers topics such as subsonic and supersonic flow, choked flow, and the calculation of area ratios in duct flow. It also discusses methods like the false position method for solving implicit equations and examines the impact of varying back pressure on flow conditions, including the formation of shock waves.

Takeaways

• 😀 Supersonic flow can only occur downstream of the throat in a converging-diverging duct (CD nozzle) if the back pressure is low enough.
• 😀 At the throat of a converging-diverging duct, the flow reaches Sonic (Mach 1) conditions, where the area is at a minimum (A*).
• 😀 The relationship between area ratio (A/A*) and Mach number (M) for steady, isentropic flow can be derived and used to calculate areas at specific Mach numbers.
• 😀 The flow is subsonic upstream of the throat, reaches Mach 1 at the throat, and becomes supersonic downstream of the throat, provided the back pressure is sufficiently low.
• 😀 For any area ratio greater than 1, there are two solutions for Mach number: one subsonic and one supersonic.
• 😀 The area ratio versus Mach number curve is U-shaped, with a dip at Mach 1, and the throat is always the minimum area (A*).
• 😀 To calculate Mach numbers from a given area ratio, methods like the false position method (or numerical iteration techniques) are used.
• 😀 The false position method is an effective iterative technique for solving implicit equations, commonly applied in fluid dynamics problems like CD nozzle calculations.
• 😀 As back pressure decreases, the flow in the CD nozzle can transition from subsonic to supersonic, and the shock locations move downstream or disappear.
• 😀 In cases where back pressure is lower than a critical point, flow is 'choked,' meaning no changes downstream of the throat affect the flow upstream of it, and the mass flow rate becomes maximum.

Q & A

What is the condition for supersonic flow in a converging-diverging duct?

Supersonic flow can only occur downstream of the throat in a converging-diverging (CD) duct, assuming that the back pressure (PB) is low enough to allow this flow. The flow accelerates from subsonic to supersonic after passing through the throat.

What happens when the Mach number is equal to 1 in a duct flow?

When the Mach number is equal to 1, the conservation of mass equation dictates that the area must be either a maximum or a minimum. Specifically, the flow can be sonic at the throat, where the area is at its minimum.

Why can't the Mach number approach 1 in a diverging duct?

In a diverging duct, if the flow is subsonic, the Mach number decreases as the area increases, so it cannot approach 1. On the other hand, if the flow is supersonic, the Mach number increases with increasing area, so it also cannot approach 1 in this region.

What is the significance of the throat area in a converging-diverging nozzle?

The throat area in a converging-diverging nozzle is the minimum area where the flow becomes sonic (Mach number = 1). This area is critical for determining the mass flow rate and for transitioning from subsonic to supersonic flow.

How does the Mach number behave in the subsonic region of a converging-diverging duct?

In the subsonic region (upstream of the throat) of a converging-diverging duct, the Mach number increases as the area decreases. This is typical of a converging duct, where the flow accelerates as the area shrinks.

What is the relationship between area ratio and Mach number in a converging-diverging duct?

The area ratio (A/A*) is related to the Mach number through the equation derived for isentropic, adiabatic flow. This equation helps determine the area at any point in the duct given the Mach number and the specific heat ratio (k).

What happens when the back pressure is reduced in a converging-diverging duct?

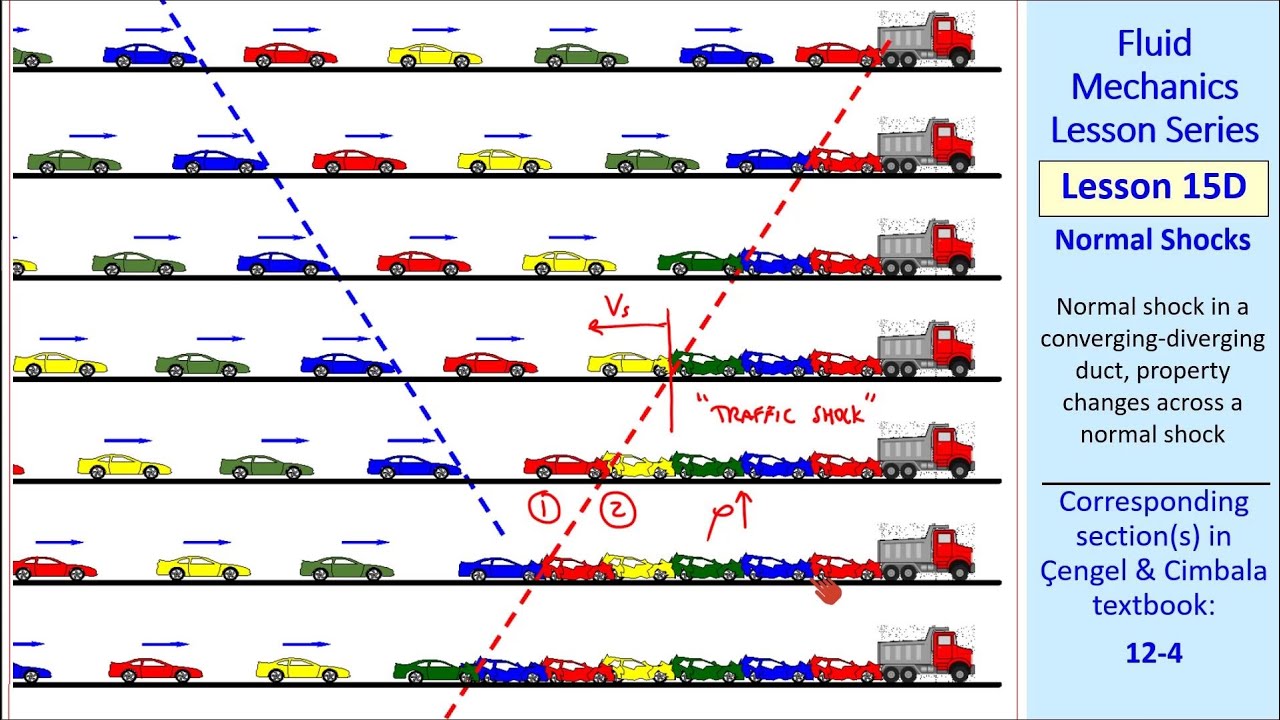
When the back pressure is lowered, the flow transitions from subsonic to supersonic through the throat, and the shock position in the diverging section moves downstream. If the back pressure is too low, shock waves can form downstream of the throat.

What is the false position method, and how is it used in solving for Mach number?

The false position method is an iterative technique used to solve implicit equations. It involves selecting two initial guesses and performing linear interpolation to converge on the correct solution. It is particularly useful for determining Mach number when given an area ratio.

What is the significance of a subsonic and supersonic root when solving for Mach number in a converging-diverging nozzle?

When solving for Mach number from the area ratio, there are typically two possible solutions: a subsonic root (Mach number < 1) and a supersonic root (Mach number > 1). The appropriate root is chosen based on whether the flow is upstream (subsonic) or downstream (supersonic) of the throat.

How does a shock wave affect flow in a converging-diverging duct?

A shock wave causes a sudden rise in pressure and a drop in Mach number. In a converging-diverging duct, if the back pressure is too high, a shock can form in the diverging section, causing the flow to decelerate from supersonic to subsonic speeds.