Linear Sequence Predict, describe, find, test, verify- IB MYP
Summary
TLDRThis video explains the process of identifying and working with linear sequences, including how to determine if a sequence is linear, quadratic, or exponential. It focuses on linear sequences, demonstrating how to find the difference between terms, which reveals a constant difference and confirms the sequence as linear. The video walks through steps like predicting new values, describing patterns, writing the nth-term formula, and testing and verifying the formula using table values and beyond. The step-by-step approach ensures a thorough understanding of how to work with linear sequences, including the general rule for their nth-term formula.
Takeaways
- 😀 Start by finding the difference between consecutive terms to identify the type of sequence: linear, quadratic, or exponential.
- 😀 A sequence with a constant difference between terms is a linear sequence.
- 😀 For a linear sequence, the nth term formula is written as 'a_n = dn + a_0', where 'd' is the common difference and 'a_0' is the zeroth term.
- 😀 When describing a linear sequence, you can say the terms increase by a constant amount (e.g., 'The difference is 2').
- 😀 To find the nth term formula for a linear sequence, first identify the difference, then calculate the zeroth term by subtracting the difference from the first term.
- 😀 For a sequence like '1, 3, 5, 7, 9', the nth term formula is 'a_n = 2n - 1' after finding the difference of 2 and the zeroth term of -1.
- 😀 Always test the nth term formula using known values from the sequence to ensure its correctness.
- 😀 Verification involves testing the formula with values of 'n' that are not included in the original table or sequence to check its validity for future terms.
- 😀 Descriptions of linear sequences can be given in different ways, such as stating the terms form a sequence of odd numbers or increase by a constant value.
- 😀 The testing process involves plugging values of 'n' from the table into the nth term formula to check if the resulting values match the actual terms.
- 😀 When verifying, use values of 'n' beyond the table to confirm that the formula holds true for terms not originally given.
Please replace the link and try again.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video

SPtDV • Part 3: Cara Menentukan Pertidaksamaan Dua Variabel dari Grafik Daerah Penyelesaian
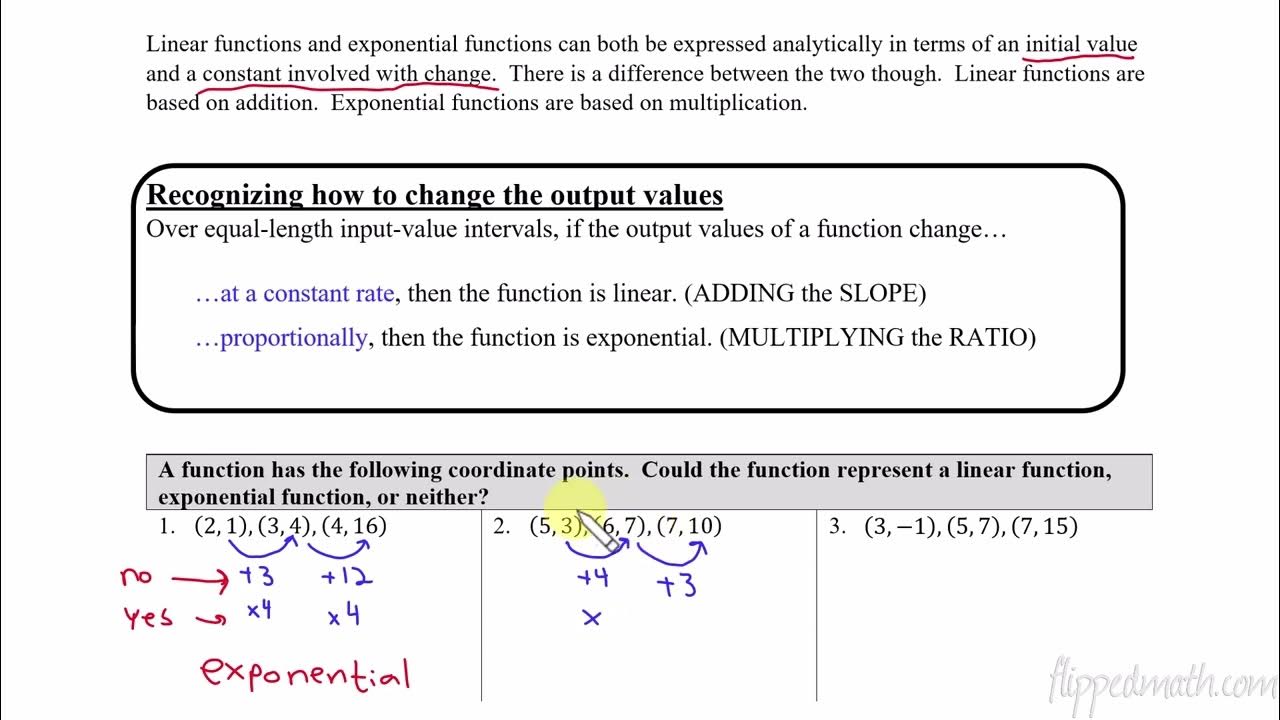
AP Precalculus – 2.2 Change in Linear and Exponential Functions
MATH 10 : DIFFERENTIATING GEOMETRIC SEQUENCE FROM AN ARITHMETIC SEQUENCE (Taglish)
SPtDV Matematika Kelas 10 • Part 1: Pertidaksamaan Linear & Kuadrat Dua Variabel

Introduction to Exponential Functions - Nerdstudy

Comparing Linear, Exponential, and Quadratic Functions
5.0 / 5 (0 votes)