DERIVADA DE UNA FUNCIÓN USANDO LA DEFINICIÓN - Ejercicio 2
Summary
Please replace the link and try again.
Takeaways
- 😀 The derivative of a function is defined as the limit of the difference quotient: (f(x + Δx) - f(x)) / Δx as Δx approaches 0.
- 😀 The definition of the derivative can use either Δx or h as the change in x, but here we use Δx.
- 😀 To evaluate the derivative, the function f(x) is expanded by substituting (x + Δx) and applying algebraic expansions.
- 😀 Algebraic skills are essential when working with derivatives, especially when expanding binomials and applying notable product formulas.
- 😀 A binomial squared is expanded using the formula: (a + b)^2 = a^2 + 2ab + b^2.
- 😀 The cube of a binomial is expanded using the formula: (a + b)^3 = a^3 + 3a^2b + 3ab^2 + b^3.
- 😀 Distributive property is used to multiply constants through the expanded expressions, simplifying the equation.
- 😀 Terms that cancel each other out (e.g., positive and negative versions of the same term) can be eliminated to simplify the expression.
- 😀 The limit form for the derivative produces an indeterminate form (0/0), which is resolved by factoring out Δx from the numerator.
- 😀 After factoring out Δx, the limit is evaluated as Δx approaches 0, eliminating the indeterminate form and simplifying the derivative expression.
- 😀 The final result for the derivative of the function is: f'(x) = 10x - 21x^2, computed using the limit definition of the derivative.
Please replace the link and try again.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video
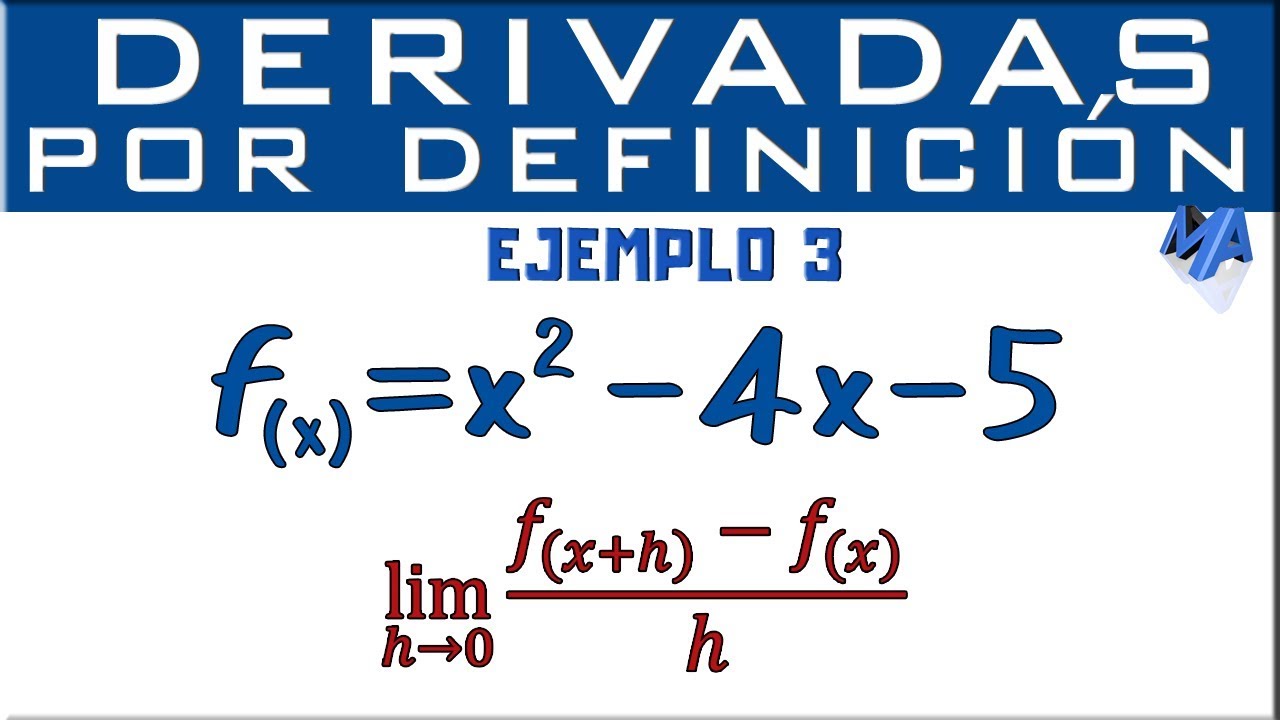
Derivada de una función usando la definición | Ejemplo 3

Derivada de una función usando la definición | Ejemplo 2

04. Maximum, minimum, growth and decrease: criterion of the first derivative

DERIVADA DE LOS 4 PASOS CON RAICES

Derivada de una función usando la definición | Ejemplo 4
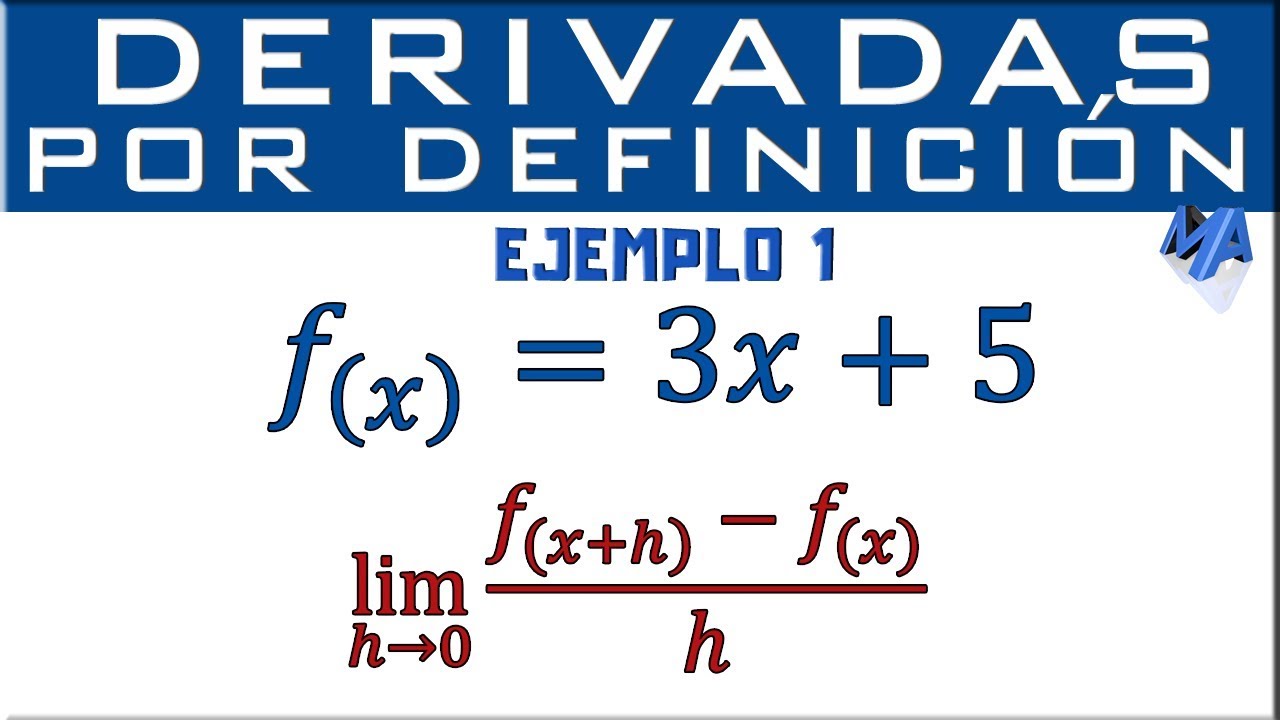
Derivada de una función usando la definición | Ejemplo 1

02. Derivative using definition as limit: Proof of formula
5.0 / 5 (0 votes)