Point Slope Form - Basic Introduction - Algebra
Summary
TLDRIn this video, the concept of point-slope form of linear equations is explored, detailing its formula and applications. The presenter explains how to identify the slope and a point from an equation in point-slope form, along with step-by-step examples. Viewers learn how to write linear equations using given slopes and points, as well as how to graph them effectively. The video concludes with a derivation of the point-slope formula from the standard slope formula, enhancing understanding of its origins and utility in graphing linear functions.
Takeaways
- 😀 The point-slope form of a linear equation is expressed as y - y1 = m(x - x1), where m is the slope and (x1, y1) is a point on the line.
- 😀 Linear equations can be represented in different forms, including standard form, slope-intercept form, and point-slope form.
- 😀 To write a linear equation in point-slope form, you need the slope and a point on the line.
- 😀 When extracting the slope and point from an equation in point-slope form, the slope is the coefficient in front of the parentheses.
- 😀 The signs of x1 and y1 in the point-slope equation are reversed compared to their appearance in the equation.
- 😀 To find the slope between two points, use the formula m = (y2 - y1) / (x2 - x1).
- 😀 When given a linear equation in point-slope form, you can identify the slope and convert the equation to standard or slope-intercept form if needed.
- 😀 Graphing a linear equation in point-slope form involves plotting a point and using the slope to find another point.
- 😀 The derivation of the point-slope formula stems from the slope formula and involves algebraic manipulation to arrive at the equation.
- 😀 Understanding point-slope form is essential for graphing linear equations and for connecting various forms of linear representation.
Q & A
What is the point-slope form of a linear equation?
-The point-slope form of a linear equation is expressed as y - y1 = m(x - x1), where m is the slope and (x1, y1) is a point on the line.
How do you identify the slope from a point-slope equation?
-The slope can be identified as the coefficient of x in the equation. For example, in the equation y - 2 = 3(x - 4), the slope is m = 3.
How can you find the point (x1, y1) from a point-slope equation?
-The point (x1, y1) can be determined by taking the opposite of the values next to x and y in the equation. For example, in y - 2 = 3(x - 4), x1 = 4 and y1 = 2.
What do you do if the equation includes negative signs?
-If the equation includes negative signs, you reverse the signs to find x1 and y1. For instance, in y - 2 = -4(x + 5), x1 becomes -5 and y1 becomes 2.
How do you write a point-slope equation from a given slope and point?
-To write a point-slope equation, substitute the slope (m) and the point (x1, y1) into the formula. For example, if the slope is -2 and the point is (-4, 5), the equation is y - 5 = -2(x + 4).
What formula do you use to find the slope when given two points?
-The slope can be found using the formula m = (y2 - y1) / (x2 - x1).
What steps should be taken to graph a linear equation in point-slope form?
-To graph a linear equation in point-slope form, first identify the slope and point, plot the point on the graph, use the slope to find a second point, and finally draw a line connecting the two points.
What is the significance of the point-slope formula in algebra?
-The point-slope formula is significant because it provides a method for writing and manipulating linear equations, making it easier to understand relationships between variables.
Can you derive the point-slope formula from the slope formula?
-Yes, the point-slope formula can be derived from the slope formula by rearranging the slope equation (m = (y2 - y1) / (x2 - x1)) into the form y - y1 = m(x - x1).
What is an example of using two points to write a point-slope equation?
-For points (2, 6) and (5, -3), calculate the slope as m = (-3 - 6) / (5 - 2) = -3. Using point (2, 6), the point-slope equation is y - 6 = -3(x - 2).
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video

2. PENGERTIAN GRADIEN - GRADIEN MELALUI 1 TITIK, 2 TITIK DAN DARI PERSAMAAN GARIS - PGL-KELAS 8 SMP

Standard Form - Lesson 6.3
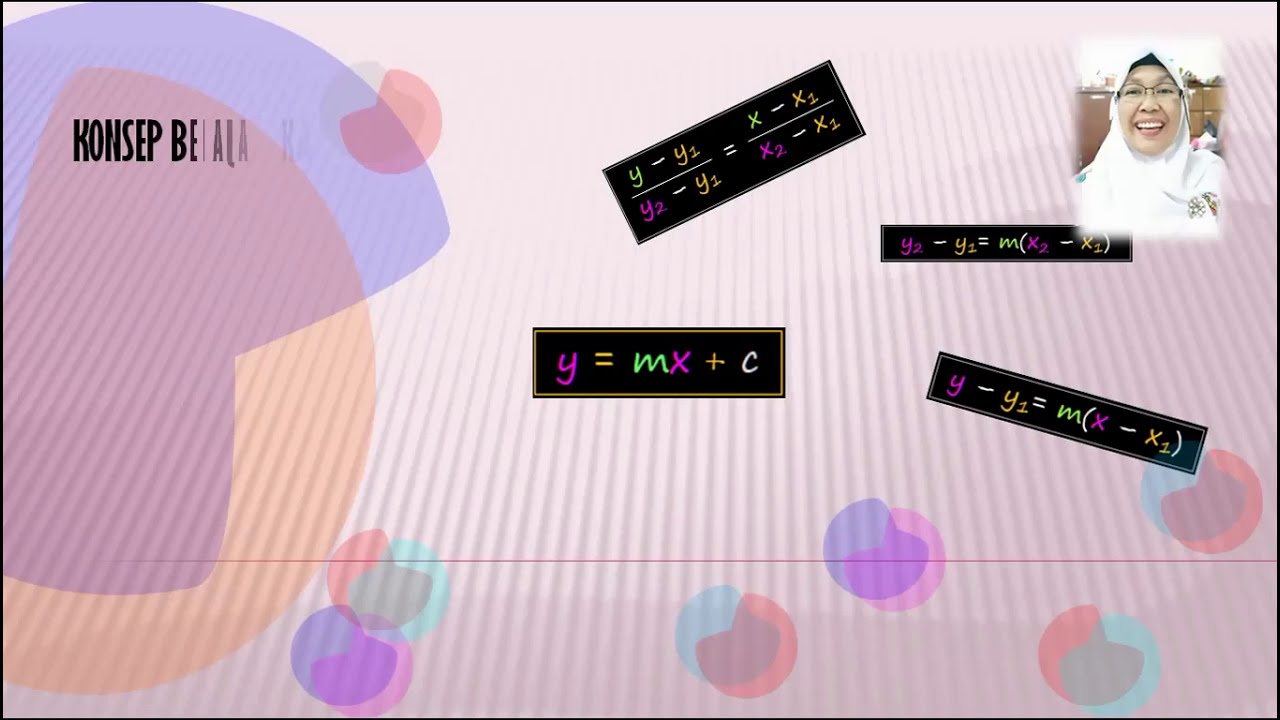
MATEMATIKA VIII Bab 4 Bagian 2 PERSAMAAN GARIS dengan KEMIRINGAN

Linear Functions

Grade 8 Lesson 2.2: Point Slope Form Short Video
Writing Equations of Parallel and Perpendicular Lines
5.0 / 5 (0 votes)