Determine the voltage VR(t) and Vc(t) for t≥0 when switch is moved from position 1 to 2.
Summary
TLDRThis video provides a detailed analysis of an electrical circuit's voltage behavior when a switch is toggled from position 1 to position 2. It begins by exploring the steady-state conditions before switching, where the capacitor behaves like an open circuit, yielding an initial voltage of 100 volts. After the switch is flipped, the circuit is analyzed using Laplace transforms to derive the current and voltage equations. The results reveal that the voltage across the capacitor remains constant at 100 volts, while the voltage across the resistor decays exponentially, showcasing the dynamic response of the circuit.
Please replace the link and try again.
Q & A
What is the main objective of the script?
-The main objective is to determine the voltages VR of the resistor and VC of the capacitor when a switch in a circuit is moved from position 1 to position 2.
What happens to the circuit when the switch is at position 1?
-When the switch is at position 1, the circuit is in a steady state, where the inductor acts like a short circuit, and the capacitor acts like an open circuit, resulting in no current flow.
What is the voltage VC of the capacitor at t = 0 minus?
-At t = 0 minus, the voltage VC of the capacitor is 100 volts since there is no current flowing in the circuit.
How is the voltage across the capacitor VC at t = 0 plus related to VC at t = 0 minus?
-VC at t = 0 plus is the same as VC at t = 0 minus, which is also 100 volts, due to the principle that the voltage across a capacitor cannot change instantaneously.
What transformation is applied to analyze the circuit after the switch moves to position 2?
-The Laplace transform is applied to represent the circuit in the s domain for easier analysis.
What does the circuit represent in the s domain when using the Laplace transform?
-In the s domain, the voltage source is represented as 50/s, and the capacitor is replaced by 1/(Cs), where C is the capacitance.
How is the current I of s derived in the s domain?
-Applying Kirchhoff's Voltage Law (KVL) leads to the equation 50/s - 100/s = 5000 * I(s) + (1/(1e-6*s)) * I(s), which is simplified to solve for I(s).
What is the expression for current I(t) after taking the inverse Laplace transform?
-The expression for current I(t) is -0.01 * e^(-200t), indicating an exponential decay with respect to time.
What is the final voltage across the resistor VR(t)?
-The final voltage across the resistor VR(t) is calculated as -50 * e^(-200t), showing how the voltage changes over time due to the current flowing through the resistor.
What does the result indicate about the behavior of the circuit after the switch is flipped?
-The results indicate that after the switch is flipped, the circuit exhibits exponential decay in current and voltage across the components, a typical response for RC circuits in transient analysis.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video

7.6 Step Response pada Rangkaian RC (3 dari 3)

RAF Modul6 ILB

Political System and Governance of Indonesia

RC snubber circuit design and calculations for inductive loads
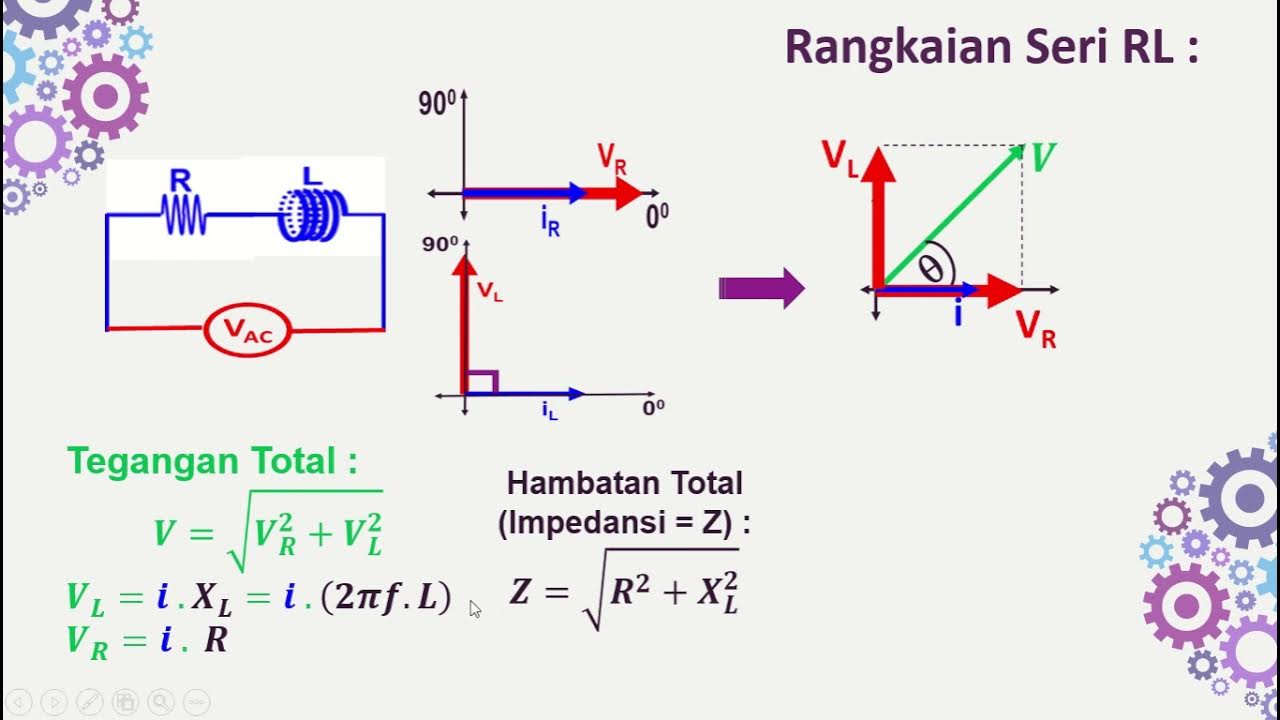
Rangkaian Seri RL-RC-LC dengan Sumber AC
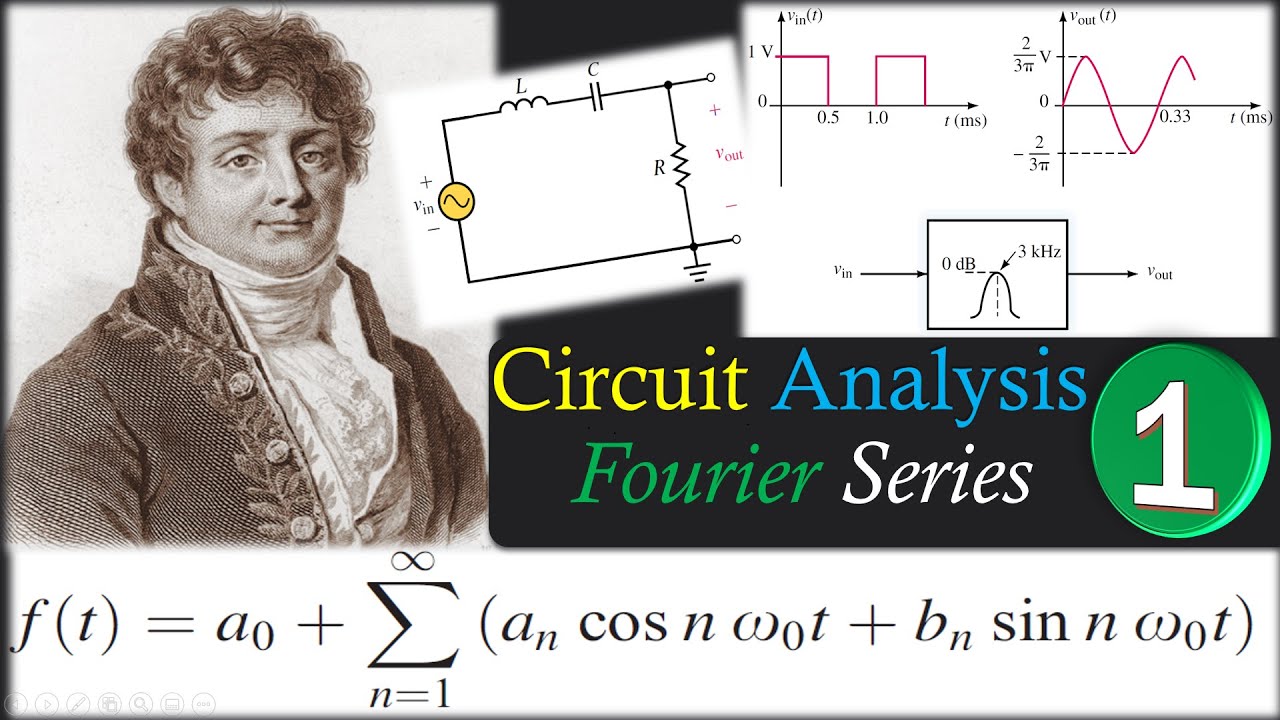
Circuit Analysis Using Fourier Series ⭐ RL Circuit Response - Nonsinusoidal Waveform ⭐ Example 1
5.0 / 5 (0 votes)