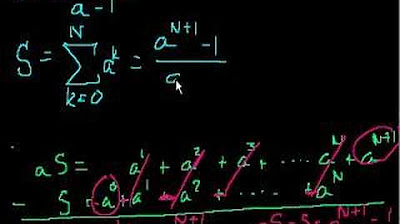Geometry of sums of powers of 1/5
Summary
TLDRThe video explains the geometric representation of the infinite sum of powers of one-fifth using a square. By dividing the square into five equal parts—four triangles and a smaller inner square—each piece represents one-fifth of the area. This process is repeated indefinitely within the inner square, illustrating the series 1/5 + 1/5² + 1/5³, and so on. When the shape is rotated four times, it fills the entire square, leading to the conclusion that the sum of the infinite series equals one-fourth.
Takeaways
- 🟢 The video begins by exploring the infinite sum of the powers of one-fifth.
- 🟩 A 1x1 square is used as the base to visualize the process.
- 🔺 Connecting the vertices to the midpoints divides the square into five equal parts.
- 📐 Four triangles and one inner square each represent one-fifth of the area.
- ♻️ The process can be repeated infinitely with the inner square, generating smaller and smaller areas.
- 🔄 Each iteration takes one-fifth of the previous inner square, spiraling down indefinitely.
- ➕ The shaded area represents a series: 1/5 + (1/5)^2 + (1/5)^3 and so on.
- 🔄 Rotating the shape three more times fills in the entire square with these areas.
- ✅ The sum of this infinite series of powers of 1/5 equals one-fourth.
- 📊 The geometric visualization demonstrates how the infinite sum converges to a finite value of 1/4.
Q & A
What does the initial one-by-one square represent in the context of the video?
-The one-by-one square represents the area that is progressively divided into smaller pieces to illustrate the sum of the powers of one-fifth.
How is the square divided initially?
-The square is divided into five equal pieces by connecting its vertices to the midpoints, resulting in four triangles around the outside and one inner square, each consisting of one-fifth of the area.
What is the area of the triangle formed by connecting the vertices to the midpoints?
-Each of the four triangles formed around the inner square has an area of one-fifth of the total square.
What happens to the inner square in the process described?
-The process is repeated on the inner square, dividing it into smaller sections, each time reducing its area by a factor of one-fifth.
What mathematical concept is illustrated by this division process?
-The division process illustrates an infinite geometric series, where each new section of the square represents a successive power of one-fifth.
What is the sum of the areas of the shaded regions?
-The sum of the areas of the shaded regions is represented by the infinite series 1/5 + (1/5)^2 + (1/5)^3, and so on.
Why is the infinite sum of powers of one-fifth equal to one-fourth?
-The sum is equal to one-fourth because when the shape is rotated three more times to fill the entire square, the total shaded area corresponds to the sum of the geometric series, which converges to 1/4.
How does rotating the shape help explain the sum of the series?
-By rotating the shape three more times, it demonstrates that the infinite sum of the areas covers the entire square, proving that the total sum is one-fourth of the square’s area.
What is the significance of the infinite geometric series in this context?
-The infinite geometric series represents the progressively smaller areas of the inner square, illustrating how the sum of these infinite fractions converges to a finite value (one-fourth).
How does the process demonstrate the concept of infinity in geometry?
-The process shows that even though the division of the square into smaller and smaller sections can continue indefinitely, the sum of these sections converges to a finite value, illustrating the idea of infinite sums in geometry.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade Now5.0 / 5 (0 votes)