Direct Variation - Basic Introduction | Algebra
Summary
TLDRThis video provides an introduction to direct variation, explaining the relationship between two variables, typically x and y, through a linear equation y = kx, where k is the constant of variation. The presenter demonstrates how to calculate k and use it to solve problems involving direct variation. Practical examples are given, such as calculating values for specific x and y pairs, determining if y varies directly with x in tables, and applying direct variation to real-world scenarios, such as calculating walking distances based on time.
Takeaways
- 📊 Direct variation describes a linear relationship between two variables, X (independent) and Y (dependent).
- 🔢 The equation for direct variation is y = kx, where k is the constant of variation.
- ✏️ To find k, divide y by x (k = y/x).
- 📈 As x increases, y increases at a proportional rate in direct variation.
- 📝 Example 1: If y = 8 when x = 2, k = 4, and the equation is y = 4x. When x = 7, y = 28.
- 🧮 Example 2: If y = 9 when x = -4, k = -9/4, and the equation is y = (-9/4)x. When x = 11, y = -99/4 or -24.75.
- 🔍 To determine if y varies directly with x in a table, check if k (y/x) remains constant for all values.
- ✔️ If k is constant across all pairs in the table, y varies directly with x. If not, it doesn't.
- 🚶 Example 3: If Karen walks 2 miles in 25 minutes, she can walk 4.8 miles in one hour (60 minutes), using the equation y = (2/25)x.
- 🔗 Direct variation problems can also include inverse and joint variations.
Q & A
What is direct variation?
-Direct variation is a relationship between two variables where one variable increases proportionally with the other. It is represented by the equation y = kx, where k is the constant of variation.
How do you calculate the constant of variation (k)?
-The constant of variation (k) is calculated by dividing the dependent variable (y) by the independent variable (x), so k = y/x.
What is the equation for direct variation?
-The equation for direct variation is y = kx, where k is the constant of variation and x is the independent variable.
If y = 8 when x = 2, how do you find y when x = 7?
-First, calculate the constant of variation k = y/x = 8/2 = 4. Then use the equation y = kx to find y when x = 7. y = 4 * 7 = 28.
If y = 9 when x = -4, what is y when x = 11?
-First, calculate the constant of variation k = y/x = 9/(-4) = -9/4. Then use the equation y = (-9/4) * x. When x = 11, y = (-9/4) * 11 = -99/4 or -24.75.
How can you determine if y varies directly with x from a table of values?
-To determine if y varies directly with x, calculate the constant of variation (k = y/x) for each pair of values. If k is the same for all pairs, then y varies directly with x.
In the first table example, does y vary directly with x?
-Yes, y varies directly with x in the first table because the constant of variation k is the same (2) for all pairs of x and y values.
Why does y not vary directly with x in the second table example?
-Y does not vary directly with x in the second table because the constant of variation (k) changes for different pairs of x and y values.
What is the relationship between distance and time in Karen’s walking example?
-The distance Karen walks varies directly with the amount of time she spends walking. This means the distance is proportional to the time.
If Karen walks 2 miles in 25 minutes, how far can she walk in one hour?
-First, calculate the constant of variation k = 2/25. Then use the equation y = (2/25) * 60 (since 1 hour = 60 minutes). The result is y = 4.8 miles.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video

Inverse Variation - Constant of Variation and Equation - Grade 9 Math Second Quarter
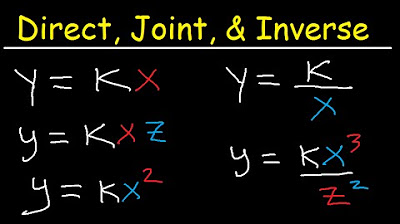
Direct Inverse and Joint Variation Word Problems
y=mx+b Ecuacíon de la recta, relación funcional
Direct and Inverse Proportion

Variación lineal: expresión algebraica, tabulación y gráfica.
Algebra - Proportional and Nonproportional Relationships
5.0 / 5 (0 votes)