Inverse of One-to-One Function | Grade 11- General Mathematics
Summary
TLDRThis educational video tutorial teaches viewers how to find the inverse of one-to-one functions. It covers three examples: solving for the inverse of a linear function (f(x) = 5x - 8), a rational function (f(x) = (x - 4)/(2x - 7)), and a cubic function (f(x) = 2x^3 - 5). The process involves changing f(x) to y, swapping x and y, solving for y, and simplifying. The video concludes with the inverse functions for each example, encouraging viewers to like and subscribe for more educational content.
Takeaways
- 📕 To find the inverse of a one-to-one function, you first replace f(x) with y.
- 📝 Interchange the variables x and y to reflect the inverse relationship.
- 💵 Solve for y to derive the inverse function.
- 💲 For a linear function like f(x) = 5x - 8, isolate y by transposing terms and dividing by the coefficient.
- 💳 The inverse of f(x) = 5x - 8 is y = (x + 8)/5.
- 📘 For rational expressions, cross-multiply to eliminate the fraction.
- 💴 Group terms with y on one side and terms without y on the other side to isolate y.
- 💹 The inverse of f(x) = (x - 4)/(2x - 7) is y = (7x - 4)/(2x - 1).
- 📗 For functions involving exponents, such as f(x) = 2x^3 - 5, solve for y by transposing and dividing to isolate the cube root.
- 💱 The inverse of f(x) = 2x^3 - 5 is y = √x + 5/2∛.
- 📝 The video provides a step-by-step guide on how to find the inverse of different types of functions, including linear, rational, and cubic functions.
Q & A
What is the first step in finding the inverse of a one-to-one function?
-The first step is to change f(x) to y, so that y equals the function expression.
How do you interchange variables to find the inverse function?
-You interchange x and y variables in the equation to express x in terms of y.
What is the inverse function of f(x) = 5x - 8?
-The inverse function of f(x) = 5x - 8 is x + 8/5.
How do you solve for y in the equation x = 5y - 8 after interchanging variables?
-You transpose -8 to the left side to get +8 and then divide both sides by 5 to isolate y.
What is the process for solving the inverse of a rational function like f(x) = (x - 4)/(2x - 7)?
-You interchange x and y, cross multiply to eliminate the fraction, and then group terms with y on one side and terms without y on the other side.
How do you handle the rational expression after interchanging x and y in f(x) = (x - 4)/(2x - 7)?
-You cross multiply to get rid of the fraction, then rearrange terms to isolate y.
What is the inverse function of f(x) = (x - 4)/(2x - 7)?
-The inverse function of f(x) = (x - 4)/(2x - 7) is 7x - 4/(2x - 1).
How do you solve for y in the equation x = y^3 - 5 after interchanging variables?
-You transpose -5 to the other side to get +5, then divide both sides by 2, and finally take the cube root of both sides.
What is the inverse function of f(x) = 2x^3 - 5?
-The inverse function of f(x) = 2x^3 - 5 is the cube root of (x + 5)/2.
Why is it necessary to cube root both sides after isolating y in the equation x = y^3 - 5?
-You cube root both sides to eliminate the power of 3 on the right side and isolate y.
What does it mean when the inverse function is represented with a negative exponent on y?
-A negative exponent on y indicates that the function is the inverse of the original function.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video

INVERSE OF ONE-TO-ONE FUNCTIONS || GRADE 11 GENERAL MATHEMATICS Q1

Functions

Lec 52 - Logarithmic Functions
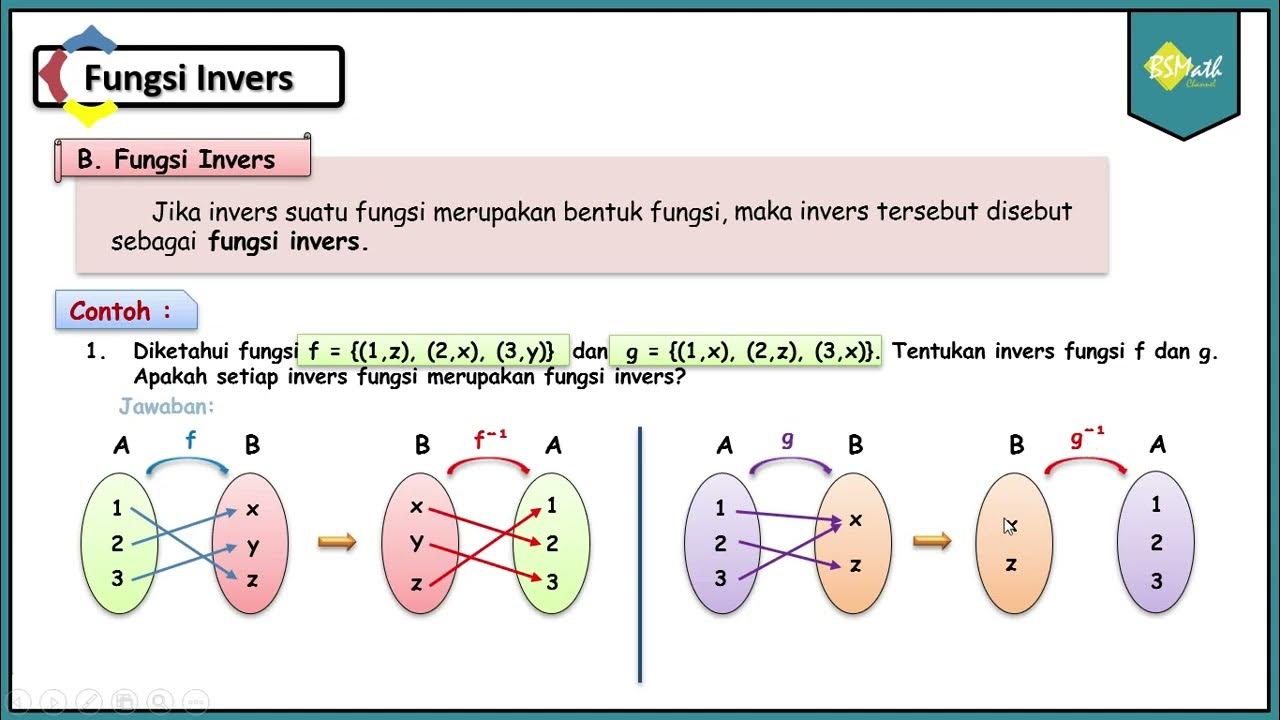
Fungsi Invers - Matematika SMA Kelas XI Kurikulum Merdeka
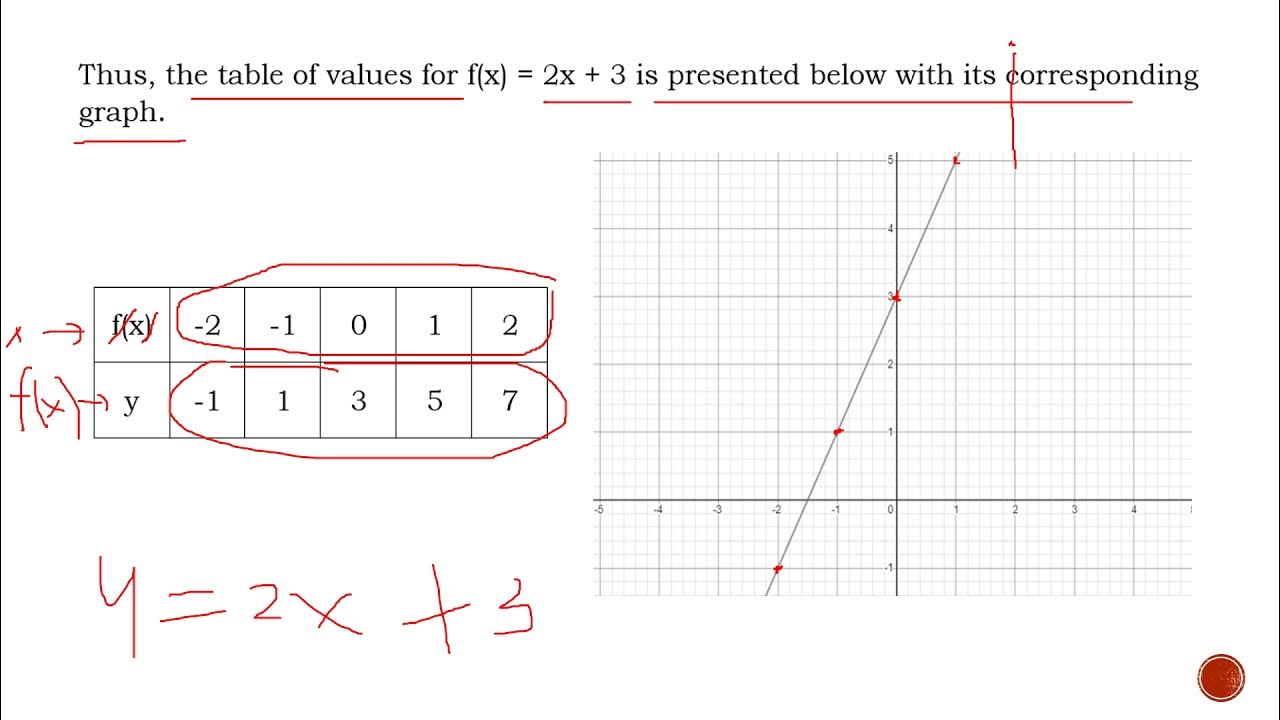
REPRESENTATIONS OF AN INVERSE FUNCTIONS | General Mathematics | Quarter 1 - Module 13

Komposisi Fungsi Part 3 - Fungsi invers dan Sifat-sifatnya [ Matematika Wajib Kelas X ]
5.0 / 5 (0 votes)