Introduction to the t Distribution (non-technical)

Summary

The video introduces the Student t-distribution, emphasizing its relevance when the population standard deviation is unknown. It explains that the t-distribution is used instead of the standard normal distribution when constructing confidence intervals for the population mean, with the degrees of freedom playing a crucial role in determining the t-value. The video illustrates how the t-distribution approaches the standard normal distribution as degrees of freedom increase, and cautions against disregarding the t-distribution even for large sample sizes, as it remains distinct from the standard normal distribution.

Takeaways

• 📚 The t-distribution, also known as Student's t-distribution, is used when the population standard deviation is unknown and needs to be estimated by the sample standard deviation.
• 🔢 The t-distribution is similar to the standard normal distribution (Z-distribution) but has more variability, with heavier tails and a lower peak.
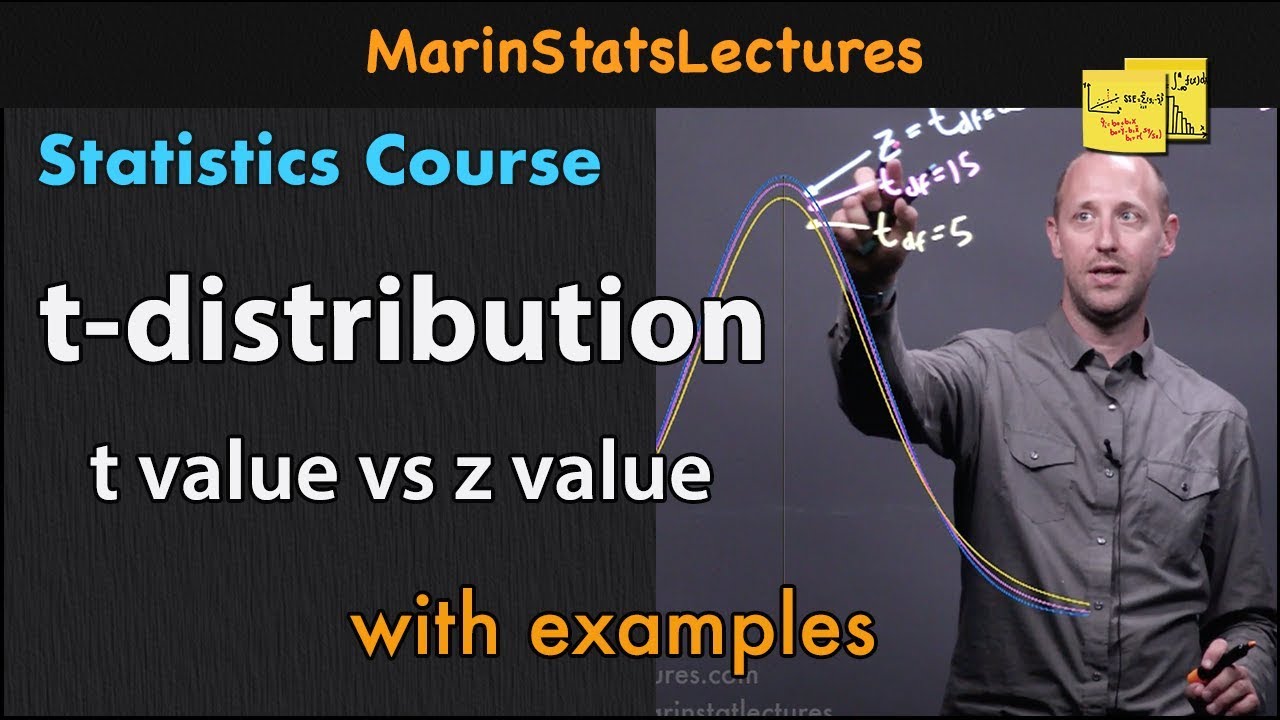
• 📈 As the degrees of freedom increase, the t-distribution approaches the standard normal distribution, becoming nearly identical at high degrees of freedom.
• 🌟 The degrees of freedom for the t-distribution is determined by the sample size (n-1), which is also the same concept used when calculating the sample variance (s^2 / (n-1)).
• 🔍 When constructing a confidence interval for the population mean (μ), if the population standard deviation (σ) is unknown, the sample standard deviation (s) must be used, and the t-distribution must be applied.
• 📊 A t-distribution with one degree of freedom is significantly different from the standard normal distribution, but as degrees of freedom increase, the differences diminish.
• 🧮 To find the appropriate t-value for a specific confidence interval and degrees of freedom, one must refer to a t-distribution table or statistical software.
• 🚫 The notion that the t-distribution can be ignored in favor of the standard normal distribution for sample sizes greater than 30 is incorrect and should not be followed.
• 📝 When using sample data to estimate a parameter, it is crucial to use values from the t-distribution rather than the standard normal distribution to avoid underestimating the margin of error.
• 📊 The shape of the t-distribution is influenced by the degrees of freedom, with higher degrees of freedom leading to a distribution shape that more closely resembles the standard normal distribution.
• 🔑 The key to statistical inference in situations where the population standard deviation is unknown lies in the correct application of the t-distribution, which accounts for the additional variability introduced by estimating σ with s.

Q & A

What is the Student t distribution?

The Student t distribution, often shortened to simply the t distribution, is a probability distribution that is used when the population standard deviation is unknown and is estimated by the sample standard deviation.

Why do we use the t distribution instead of the standard normal distribution in certain cases?

We use the t distribution instead of the standard normal distribution when the population standard deviation (sigma) is unknown and we have to estimate it using the sample standard deviation (s). This introduces more variability, and thus the t distribution has greater variance and heavier tails compared to the standard normal distribution.

How does the t distribution differ from the standard normal distribution?

The t distribution is similar to the standard normal distribution in that both are symmetric about zero and bell-shaped. However, the t distribution has heavier tails and a lower peak, which accounts for the additional variability when using the sample standard deviation as an estimate for the population standard deviation.

What are degrees of freedom in the context of the t distribution?

In the context of the t distribution, degrees of freedom refer to a parameter that influences the shape of the distribution. Specifically, in the formula for the t distribution, the degrees of freedom are equal to the sample size minus one (n-1).

How does the shape of the t distribution change with increasing degrees of freedom?

As the degrees of freedom increase, the t distribution tends to resemble the standard normal distribution more closely. With higher degrees of freedom, the t distribution's tails become lighter and its peak becomes higher, approaching the shape of the standard normal distribution.

What is the implication of the t distribution's shape for statistical inference?

The shape of the t distribution has implications for constructing confidence intervals. When the population standard deviation is unknown, and the sample standard deviation is used as an estimate, the critical values for constructing confidence intervals are taken from the t distribution rather than the standard normal distribution.

How do you determine the appropriate t value for a 95% confidence interval?

To determine the appropriate t value for a 95% confidence interval, you place 95% of the area in the middle of the distribution and split the remaining 5% evenly into the two tails. The t value that corresponds to an area to the right of 0.025 (the right tail) is used as the critical value for the confidence interval.

What happens to the t value as the degrees of freedom approach infinity?

As the degrees of freedom approach infinity, the t value for a 95% confidence interval converges to the z value of 1.96 from the standard normal distribution. This is because a t distribution with infinite degrees of freedom is effectively the same as the standard normal distribution.

Why should we not use the standard normal distribution values when the sample standard deviation is used as an estimate for the population standard deviation?

Using the standard normal distribution values when the sample standard deviation is used as an estimate for the population standard deviation can lead to an underestimation of the margin of error. The t distribution accounts for the additional variability introduced by estimating the population standard deviation from sample data, so it is more appropriate to use t distribution values in such cases.

What is the recommended approach for using the t distribution, regardless of the sample size?

The recommended approach is to use the t distribution for statistical inference when the population standard deviation is unknown and the sample standard deviation is used as an estimate, regardless of the sample size. This ensures that the confidence intervals and other statistical inferences are adjusted for the additional variability present in the estimation of the population parameter.

How can one find the appropriate t value for a given confidence interval and degrees of freedom?

The appropriate t value for a given confidence interval and degrees of freedom can be found using a t table or statistical software. These resources provide t values for various confidence levels and degrees of freedom, allowing for accurate construction of confidence intervals and other statistical analyses.

Outlines

00:00

📚 Introduction to the Student t Distribution

This paragraph introduces the concept of the Student t distribution, often referred to simply as the t distribution. It explains that the video will not delve deeply into the mathematical origins of the t distribution but will focus on its practical applications. The discussion begins with the premise of drawing a random sample from a normally distributed population and the standard normal distribution of the sample mean. It highlights the common issue of not knowing the population standard deviation (sigma) and the workaround of using the sample standard deviation instead. This leads to the definition of the t statistic and its distribution, which has n-1 degrees of freedom. The paragraph also touches on the concept of degrees of freedom and how the t distribution resembles the standard normal distribution but with more variability, resulting in heavier tails and a lower peak. The influence of degrees of freedom on the shape of the t distribution is emphasized, showing that as degrees of freedom increase, the t distribution approaches the standard normal distribution.

05:01

📊 Confidence Intervals and the t Distribution

This paragraph delves into the construction of confidence intervals for the population mean when the population standard deviation is unknown. It explains the standard approach using the Z statistic from the standard normal distribution and the associated value of 1.96 for a 95% confidence interval. However, when sigma is unknown, the paragraph clarifies that the t distribution must be used instead, leading to the determination of a t value for the confidence interval. The paragraph provides a detailed explanation of how to find the appropriate t value based on degrees of freedom and how this value changes with different sample sizes. It also addresses the common misconception that the t distribution can be ignored for large sample sizes, emphasizing the importance of using the t distribution when the sample standard deviation is used, regardless of the sample size.

Keywords

💡Student t distribution

The Student t distribution, often simply referred to as the t distribution, is a type of probability distribution that is used in statistics when the sample size is small or when the population standard deviation is unknown. It is particularly useful for constructing confidence intervals for the population mean. The video script discusses the t distribution in the context of estimating the population mean when the standard deviation is unknown, hence using the sample standard deviation and resulting in a t statistic.

💡Random sample

A random sample is a subset of a population in which every member of the population has an equal chance of being included. In the context of the video, a random sample of n observations is drawn from a normally distributed population to estimate the population mean. The use of a random sample is crucial for making valid statistical inferences about the population from the sample data.

💡Standard normal distribution

The standard normal distribution, also known as the Z distribution, is a special case of the normal distribution where the mean is 0 and the standard deviation is 1. It is used in statistics to standardize data and make comparisons across different datasets. In the video, the standard normal distribution is initially used to describe the quantity (X bar - mu) / (sigma / sqrt(n)), but when the population standard deviation is unknown, this quantity is instead described by the t distribution.

💡Population mean (mu)

The population mean, denoted by the Greek letter mu (μ), is the average value of a characteristic for an entire population. It is a key parameter in statistical analysis and is often the focus of inferential statistics. The video script discusses the process of estimating the population mean using the t distribution when the population standard deviation is unknown.

💡Sample standard deviation (s)

The sample standard deviation is a measure of the amount of variation or dispersion in a sample of data. It is an estimate of the population standard deviation and is calculated from the sample data. In the video, the use of the sample standard deviation in place of the population standard deviation leads to the use of the t distribution instead of the standard normal distribution for statistical analysis.

💡Degrees of freedom

Degrees of freedom in a statistical context refer to the number of independent observations that can vary without constraint in a calculation. In the context of the t distribution, the degrees of freedom are related to the sample size and are equal to n-1. The shape of the t distribution changes with the degrees of freedom, with more degrees of freedom resulting in a distribution that more closely resembles the standard normal distribution.

💡Confidence interval

A confidence interval is a range of values, derived from a statistical procedure, that is likely to contain the true value of an unknown parameter, such as the population mean. The level of confidence, typically expressed as a percentage (e.g., 95%), represents the degree of certainty that the interval contains the true parameter value. In the video, the concept of a confidence interval is used to estimate the population mean when the standard deviation is unknown.

💡t statistic

The t statistic is a measure used in hypothesis testing and the construction of confidence intervals when the population standard deviation is unknown. It is calculated as the difference between the sample mean (X bar) and the hypothesized population mean (mu), divided by the sample standard deviation (s) and the square root of the sample size (n). The t statistic follows a t distribution with n-1 degrees of freedom and is used to make inferences about the population mean.

💡Statistical inference

Statistical inference is the process of drawing conclusions about a population based on data collected from a sample. It involves using probability theory to make predictions about the population parameters. In the video, statistical inference is the overarching theme, with the focus on estimating the population mean using the t distribution when the population standard deviation is unknown.

💡Sample size (n)

The sample size, denoted by n, refers to the number of observations or individuals in a sample. It is an important factor in statistical analysis as it affects the precision of estimates and the power of hypothesis tests. In the context of the t distribution, the sample size directly influences the degrees of freedom and, consequently, the shape of the t distribution.

💡Margin of error

The margin of error is the difference between the estimated value of a parameter and the actual value of that parameter. It is used in statistical analysis to express the uncertainty associated with a point estimate. In the context of the video, the margin of error is related to the confidence interval for the population mean and is affected by the choice of distribution (t or standard normal) based on the known or unknown population standard deviation.

Highlights

Introduction to the Student t distribution, often shortened to simply the t distribution.

The t distribution arises when the population standard deviation is unknown and the sample standard deviation is used as an estimate.

The quantity X bar minus mu over sigma over the square root of n has the standard normal distribution when sigma is known.

In practice, we often don't know the population standard deviation sigma, so we use the sample standard deviation s to estimate it.

The statistic X bar minus mu over s over the square root of n has a t distribution with n-1 degrees of freedom when sigma is unknown.

The concept of degrees of freedom is important for the t distribution, and it is tied to the sample variance s squared divided by n-1.

The t distribution looks similar to the standard normal distribution but has greater variance, heavier tails, and a lower peak.

As the degrees of freedom increase, the t distribution tends toward the standard normal distribution.

The shape of the t distribution depends on the degrees of freedom, with higher degrees of freedom resulting in a distribution closer to the standard normal.

In statistical inference, when constructing a confidence interval, the appropriate values for the margin of error are derived from the t distribution, not the standard normal distribution, when sigma is unknown.

The t value for a 95% confidence interval changes based on the degrees of freedom and is greater than the z value of 1.96 from the standard normal distribution.

Even with large sample sizes, such as 30 or 100 degrees of freedom, the t value remains slightly higher than the standard normal z value of 1.96.

When using a sample standard deviation in normally distributed populations, values from the t distribution should be used for calculations, regardless of sample size.

The t distribution's practical applications include constructing confidence intervals when the population standard deviation is unknown.

The video provides a visual comparison of the standard normal distribution and the t distribution with one degree of freedom.

The t distribution's heavier tails and lower peak reflect the increased variability when estimating a parameter with a statistic.

The video explains the relationship between the sample size, degrees of freedom, and the t distribution's convergence to the standard normal distribution.

The video emphasizes the importance of using the correct distribution (t or standard normal) when calculating confidence intervals in statistical inference.

Transcripts

Let's look at an introduction to the Student t distribution,

often shortened to simply the t distribution.

This video is a little light on mathematical details,

so if you're looking for how the t distribution arises mathematically,

or its pdf, I go through that in another video.

Suppose we are about to draw a random sample of n observations

from a normally distributed population.

We've previously learned that the quantity X bar minus mu

over sigma over the square root of n has the standard normal distribution.

And we typically label that with the letter Z.

Previously, we've used this notion to construct a confidence interval

for the population mean mu.

But in practice we encounter a problem, and that problem is

that we don't know the value of the population standard deviation sigma.

Sigma is a parameter, the standard deviation for the entire population,

and we don't typically know its value, so we can't use that value in a formula.

So we do the next best thing, and instead of using the population standard deviation,

we're going to use our sample standard deviation to estimate it

and then we're going to have a statistic X bar minus mu

over s over the square root of n, where s is our sample standard deviation.

But something very fundamental has changed here.

Sigma is a constant but we don't know its value

so we use s, which is a statistic, and this statistic s has a sampling distribution,

and it would vary from sample to sample.

And so this quantity down here

would no longer have the standard normal distribution.

And we call this quantity or we label it as t

because it has a t distribution.

When we are sampling from a normally distributed population,

the quantity X bar minus mu over s over the square root of n

has the t distribution with n-1 degrees of freedom.

The concept of degrees of freedom can be a bit of a tricky one,

so I'm not going to get into the details here.

But the degrees of freedom for the t

and if you recall when we had our sample variance s squared, we divided by n-1.

those two notions are very much tied together.

What does the t distribution look like?

We'll look at that in a moment, but if we look at this statistic,

it looks very much like our Z statistic, which has the standard normal distribution,

Except we've replaced the population standard deviation

with the sample standard deviation.

We are estimating a parameter with a statistic

so there is greater variability. So our t distribution is going

to look a lot like the standard normal distribution, except with greater variance.

Here's a plot of the standard normal distribution in white

and a t distribution with one degree of freedom in red.

We can see that both distributions are symmetric about zero and bell-shaped,

but the t distribution has heavier tails and a lower peak.

The exact shape of the t distribution depends on the degrees of freedom.

A very fundamental point here is that as the degrees of freedom increase,

the t distribution tends toward the standard normal distribution.

So I'm going to let the degrees of freedom increase and let's see what happens.

as the degrees of freedom increase here

we see the red curve getting closer and closer and closer to the white curve.

or in other words, as the degrees of freedom increase

the t distribution is tending towards the standard normal distribution.

I've stopped it here at 20 degrees of freedom,

and the curves might look close, but if we look very closely we would see that

the t distribution still has slightly heavier tails and a slightly lower peak.

But if I let those degrees of freedom continue to increase,

the t distribution is going to get closer and closer and closer to the standard normal distribution.

This has some implications for us in statistical inference.

Here I'm going to look at constructing a 95% confidence interval,

but the same notion would hold in many other situations as well.

If we are sampling from a normally distributed population,

and we happen to know the value of the population standard deviation sigma,

then we've discussed previously that this is the appropriate formula for our confidence interval.

This 1.96 comes from the standard normal distribution.

And I've drawn in the standard normal distribution down here.

If we want a 95% confidence interval

then we put an area of 0.95 in the middle,

and we divide up the remaining area of 0.05

evenly into the two tails,

putting 0.025 in the right tail and 0.025 in the left tail.

We call the value here with an area to the right of 0.025

z_.025,

and that value is 1.96,

which we've encountered previously,

and we can find from the standard normal table or software.

But if sigma is not known,

then we can't use it in our confidence interval formula,

and we would have to replace it with the sample standard deviation.

But then we should no longer use 1.96,

we shouldn't use a value based on the standard normal distribution,

we need to use a value based on the t distribution.

So down here I've drawn in a t distribution,

and we use the same logic in that we want to put 95%

of the area in the middle and split up the remaining area evenly into the two tails.

And so what we want to find

is from this t distribution the t value

that gives an area to the right of 0.025.

Because the t distribution has greater area in the tails

and greater variability than the standard normal distribution,

How much greater?

Well that depends on the degrees of freedom,

because the shape of the t distribution depends on the degrees of freedom.

But let's look at a few values.

Here I have a table with the appropriate t value for various degrees of freedom.

This first column has the sample size n.

The second column has the degrees of freedom,

which are n-1 for the case we're discussing today.

And then the appropriate t value for a 95% confidence interval.

This can be found from a t table or software.

Take note that at infinite degrees of freedom we get our z value of 1.96,

that is our z_.025 value,

and that's because a t distribution with infinite degrees of freedom

is the same as the standard normal distribution.

But if we look up here with five degrees of freedom,

we see that the t value is 2.571,

which is quite a bit bigger than the 1.96 value from the standard normal distribution.

As the degrees of freedom increase,

the t distribution is getting closer and closer and closer to the standard normal distribution,

so these t values are getting closer and closer and closer and closer

to 1.96, the value from the standard normal distribution.

Some sources go so far as to say that if the sample size is greater than 30

just forget all about the t distribution and use the standard normal distribution.

But if you take statistics from me, forget you ever heard such a notion.

If we look here at 30 degrees of freedom

we see that the t value is 2.042,

which to me at least is quite a bit bigger than the z value of 1.96.

Even at 100 degrees of freedom the t value

still is a little bit different than the 1.96.

And so if we use this z value when we should be using the t value

our calculated margin of error will be smaller than it should be.

If we are sampling from a normally distributed population

and we are using a standard deviation that is based on our sample's data,

then we should be using values from the t distribution

and not the standard normal distribution,

regardless of the sample size.