Kirchhoff's current law | Circuit analysis | Electrical engineering | Khan Academy
Summary
TLDRThis educational video script explains Kirchhoff's Current Law (KCL) in the context of circuit analysis. It begins with a discussion of resistors and Ohm's Law, then introduces the concept of a node in a circuit. The script uses the principle of charge conservation to demonstrate that the total current entering a node equals the total current leaving it, leading to KCL's formulation: the sum of currents into a node equals the sum of currents out of the node. The explanation is illustrated with examples that show how to apply KCL to solve for unknown currents in various scenarios, emphasizing its fundamental role in circuit analysis.
Takeaways
- 🔌 Kirchhoff's Current Law states that the total current flowing into a node (junction) in a circuit is equal to the total current flowing out.
- 🌐 The law is based on the principle of charge conservation, meaning that charge does not accumulate at any point in a circuit.
- 📐 In a simple series circuit with resistors, the current through each resistor is the same because current cannot accumulate at a node.
- 🔄 When additional resistors are added to a node, the currents through each resistor may differ, but the sum of currents entering the node equals the sum of currents leaving it.
- 📉 Mathematically, Kirchhoff's Current Law can be expressed as the sum of currents entering a node (i_in) equals the sum of currents leaving the node (i_out).
- 📋 The law can be generalized to any node in a circuit, not just those with resistors, making it a fundamental principle in circuit analysis.
- 🔢 Currents can be defined as flowing into a node or out of a node; the choice is arbitrary, but the sum must equal zero for the node to be in equilibrium.
- ➡️ If all currents entering a node are known, Kirchhoff's Law can be used to calculate the unknown current flowing out of the node.
- ↪️ Conversely, if all currents leaving a node are known, the law can be used to determine the unknown current flowing into the node.
- 🔄 The law is applicable regardless of the direction of current flow chosen; it can be expressed with currents all entering or all leaving the node.
- 🛠️ Kirchhoff's Current Law is a cornerstone in circuit analysis and is used frequently to solve complex circuit problems.
Q & A
What is Kirchhoff's Current Law?
-Kirchhoff's Current Law states that the total current flowing into a node (or junction) in a circuit is equal to the total current flowing out of that node. It's based on the conservation of charge, meaning that charge cannot accumulate at any point in a circuit.
How is the conservation of charge related to Kirchhoff's Current Law?
-The conservation of charge is the principle that charge cannot be created or destroyed, and it must be conserved in any physical system. In the context of Kirchhoff's Current Law, this means that the total amount of charge entering a node must equal the total amount of charge leaving the node, as charge cannot pile up at the node.
What happens when you add another resistor to a node?
-When you add another resistor to a node, it introduces an additional current path. This means that the currents flowing into and out of the node are no longer necessarily the same, and the law can be expressed as the sum of currents entering the node equals the sum of currents leaving the node plus any currents entering through the new resistor.
How do you mathematically express Kirchhoff's Current Law?
-Mathematically, Kirchhoff's Current Law can be expressed as the summation of currents entering a node (Σi_in) equals the summation of currents leaving the node (Σi_out). Alternatively, it can be stated as the sum of currents entering a node equals zero if currents are defined as flowing out of the node.
What does it mean if the sum of currents entering a node equals zero?
-If the sum of currents entering a node equals zero, it means that for every current that enters the node, there is an equal and opposite current that leaves the node. This ensures that there is no net accumulation of charge at the node.
Can you give an example of how to apply Kirchhoff's Current Law?
-Sure, if you have a node with three currents of 1 amp each entering and one unknown current (i), applying Kirchhoff's Current Law would mean that 1 + 1 + 1 + i = 0. Solving for i gives you i = -3 amps, indicating that 3 amps must be leaving the node to balance the 3 amps entering.
What is the significance of defining currents as flowing into or out of a node?
-Defining currents as flowing into or out of a node is a matter of convention and can simplify calculations. By defining all currents as flowing into a node, any current that actually flows out can be represented as a negative value. Conversely, defining all currents as flowing out simplifies the calculation for currents flowing into the node.
How does Kirchhoff's Current Law relate to Ohm's Law?
-Kirchhoff's Current Law and Ohm's Law are separate concepts. Ohm's Law relates voltage, current, and resistance in a circuit, while Kirchhoff's Current Law deals with the conservation of charge at nodes. However, they are often used together in circuit analysis to solve for unknown currents and voltages.
Is there a similar law for voltage in circuits?
-Yes, Kirchhoff's Voltage Law (also known as Kirchhoff's Loop Rule) is the counterpart to Kirchhoff's Current Law. It states that the algebraic sum of the potential differences (voltages) in any closed loop or mesh in a network is zero.
Why is Kirchhoff's Current Law important in circuit analysis?
-Kirchhoff's Current Law is important in circuit analysis because it provides a fundamental principle that helps in determining the flow of current through various components in a circuit. It is one of the key tools used in solving complex circuits for unknown currents and voltages.
Can Kirchhoff's Current Law be applied to circuits with capacitors?
-Yes, Kirchhoff's Current Law can be applied to circuits with capacitors as well as resistors. The law is general and applies to any node in a circuit, regardless of the components connected to it.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video
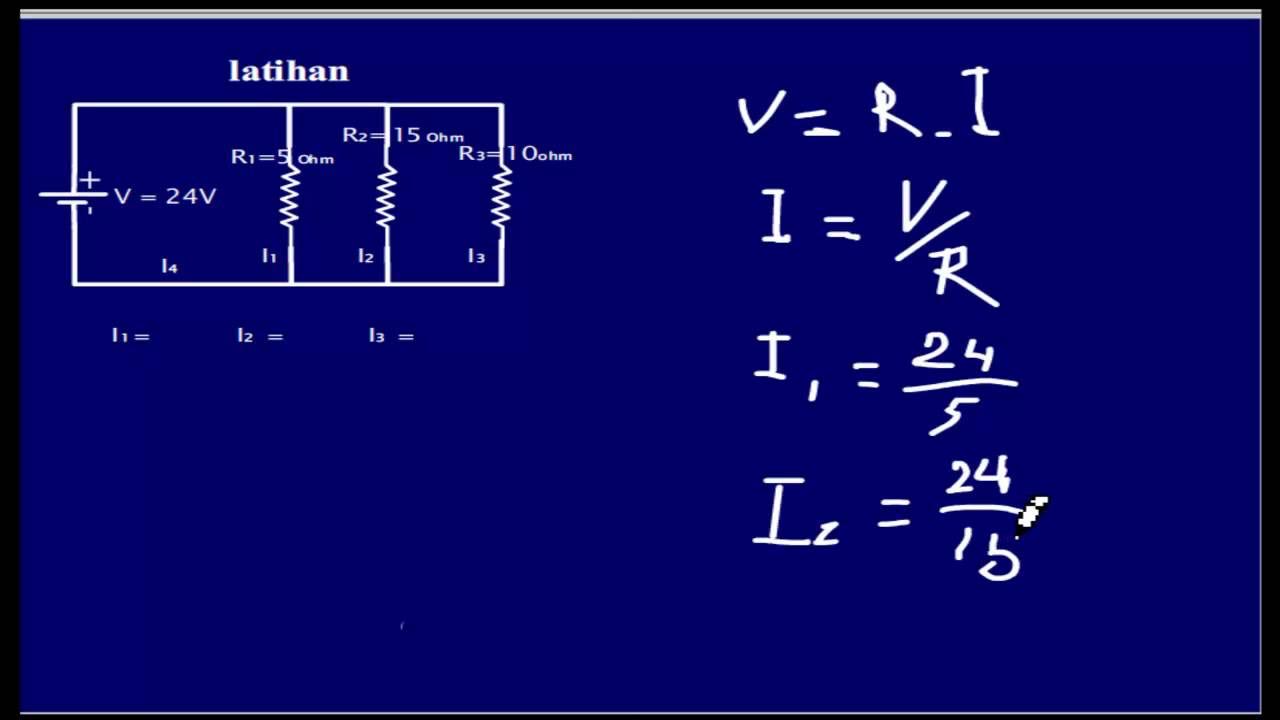
Elektronika Dasar 002 Resistor 02 Universitas Jember
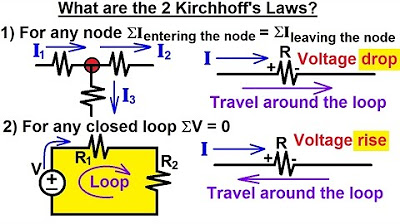
Electrical Engineering: Basic Laws (8 of 31) What Are Kirchhoff's Laws?

Analisis Node (Simpul)

Kirchhoff's Current Law (KCL) - How to Solve Complicated Circuits

Aula 2. Leis De Kirchhoff Exercício 1
7. Kirchhoff Current Law ( KCL ) - Theory, Sign Conventions, Example Problem |BEE|
5.0 / 5 (0 votes)