7. Kirchhoff Current Law ( KCL ) - Theory, Sign Conventions, Example Problem |BEE|

Trouble- Free

4 Jan 202411:38

Summary

TLDRIn this educational video, the host explains the concept of Kirchhoff's Current Law (KCL), which states that the algebraic sum of currents at a junction is zero. They use a simple circuit diagram to demonstrate how to apply KCL to find unknown currents, emphasizing the importance of understanding incoming and outgoing current directions. The video also offers tips for solving more complex circuits, highlighting the need to identify junctions and form equations accordingly.

Takeaways

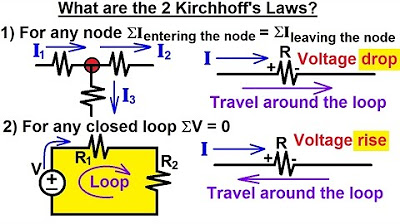
😀 The video discusses Kof's current law, also known as SKCL, which states that the algebraic sum of electric currents meeting at a junction is equal to zero.
🔌 A junction is defined as a point where three or more branches meet, and branches are parts of a circuit.
📈 Incoming currents at a junction are considered positive, while outgoing currents are considered negative.
🔄 The KCL can be referred to as the conservation of charge, emphasizing the balance of currents at a junction.
📚 The video provides an example to illustrate how to apply KCL in a circuit, involving currents labeled as i1, i2, i3, i4, and i5.
🔢 In the example, the KCL equation is formed by considering the sum of incoming currents (i1, i3, i4) equal to the sum of outgoing currents (i2, i5).
🔍 The video solves three sub-questions based on the circuit diagram, finding values for ix, iy, and iz under different conditions.
🤔 The video explains that in complex networks, you must identify all junctions, assume initial voltages, and form KCL equations for each junction.
🔋 The video mentions that in complex circuits, voltages and resistances will be involved, and Ohm's Law (V = IR) can be used to solve for currents and resistances.
📘 The video concludes by encouraging viewers to watch more videos on the channel for further understanding of complex circuits and the application of KCL.

Q & A

What is the main principle of Kirchhoff's Current Law (KCL)?
-Kirchhoff's Current Law states that the algebraic sum of the electric currents meeting at a junction or node is equal to zero. This means that the sum of incoming currents is equal to the sum of outgoing currents at any given node.
What is a junction in the context of electrical circuits?
-A junction is a point in an electrical circuit where three or more branches meet. It is the location where currents can either enter or leave the junction.
How are incoming and outgoing currents treated in KCL?
-In KCL, incoming currents are considered as positive, while outgoing currents are considered as negative. This helps in setting up the algebraic sum to zero for the currents meeting at a junction.
Can you explain the concept of conservation of charge in relation to KCL?
-The conservation of charge is a fundamental principle that states the total charge in an isolated system remains constant over time. In the context of KCL, this principle is reflected in the fact that the sum of currents at a junction must be zero, indicating that charge is neither created nor destroyed, just transferred between branches.
What is the significance of the direction of current in applying KCL?
-The direction of current is significant when applying KCL because it determines whether a current is considered incoming (positive) or outgoing (negative). If the direction is not given, it must be assumed for the purpose of setting up the KCL equations.
In the given example, how is the KCL equation set up for the circuit diagram?
-In the example, the KCL equation is set up by identifying the incoming and outgoing currents at the junction. The equation is formed by summing the incoming currents (5 amp, iZ, and iY) and setting it equal to the sum of the outgoing currents (IX and 3 amp).
What is the first condition given in the example for finding IX, and what is the result?
-The first condition given is when iY is equal to 5 amp and iZ is equal to 3 amp. Substituting these values into the KCL equation results in IX being equal to 10 amp.
How is the second condition in the example used to find the value of iY?
-The second condition states that IX is equal to 4 amp and iZ is equal to 4 times iY. By substituting these values into the KCL equation and solving for iY, it is found that iY equals 2 amp.
What does the third condition in the example tell us about the relationship between IX, iY, and iZ?
-The third condition states that IX is equal to iY, which is also equal to iZ. Using the KCL equation and substituting iZ for IX and iY, it is determined that iZ is equal to -2 amp, indicating that the current is flowing in the opposite direction.
What are some steps to follow when solving complex circuit problems using KCL?
-When solving complex circuits, one should first identify all junctions, assume initial voltages (VKN = 0), and unknown current directions. Then, form KCL equations for each junction. Remember sign conventions for adding or subtracting voltages, and use Ohm's Law (V = IR) to relate voltage, current, and resistance. These steps will help in solving for unknowns in more complicated circuits.

Outlines

00:00

📚 Introduction to Kirchhoff's Current Law (KCL)

This paragraph introduces the concept of Kirchhoff's Current Law (KCL), which is an essential principle in electrical circuit analysis. It explains that the algebraic sum of currents meeting at a junction or node equals zero, emphasizing the importance of understanding the terms 'junction' and 'branch'. The paragraph also illustrates how to apply KCL using an example with five currents (i1, i2, i3, i4, i5), where incoming currents are positive, and outgoing currents are negative. The example demonstrates the process of setting up and solving an equation based on KCL to find unknown currents in a circuit.

05:03

🔍 Applying KCL to Solve Circuit Problems

This paragraph continues the discussion on KCL by providing a practical example of a circuit diagram with three sub-questions to solve. It explains the process of identifying the junction and the currents entering and leaving it, using the law to set up equations for the currents (ix, iy, and iz). The paragraph demonstrates solving for ix when iy and iz are given, finding iy when ix and a multiple of iy are provided, and determining iz when ix, iy, and iz are all equal. It concludes with a reminder of the importance of understanding KCL for solving more complex circuits.

10:03

🛠️ Advanced Circuit Analysis Techniques

The final paragraph delves into the steps and conventions needed for analyzing more complex electrical circuits using KCL. It advises viewers to identify all junctions, assume initial voltages, and form KCL equations for each junction, even when current directions are not explicitly given. The paragraph also covers the rules for adding or subtracting voltages based on the connection of terminals and the fundamental principles of current flow from higher to lower potential. It wraps up with a mention of Ohm's Law and its variations, setting the stage for future videos that will tackle more complicated circuits and their analysis.

Mindmap

Keywords

💡Kirchhoff's Current Law (KCL)

Kirchhoff's Current Law (KCL), also known as Kirchhoff's first law or the node rule, states that the algebraic sum of currents entering a junction is equal to zero. This law is based on the principle of conservation of charge, which implies that the total amount of electric charge entering a junction must equal the total amount leaving it. In the video, the presenter explains how KCL applies to electrical circuits, illustrating that incoming currents are considered positive and outgoing currents are negative, resulting in the equation: sum of incoming currents = sum of outgoing currents.

💡Junction

A junction in an electrical circuit is a point where three or more conductors meet. It is crucial for applying Kirchhoff's Current Law, as KCL is concerned with the currents at these junctions. The video describes a junction as a node where currents converge, and the presenter uses it to demonstrate the concept of KCL, showing how the sum of currents at a junction should equal zero.

💡Branch

A branch in a circuit is a path that connects various components in a circuit. It is significant in analyzing circuits using KCL and KVL (Kirchhoff's Voltage Law), as it helps in understanding how current flows through different parts of the circuit.

Highlights

Introduction to the concept of Kirchhoff's Current Law (KCL).

Explanation that KCL states the algebraic sum of electric currents at a junction is zero.

Definition of a junction as a point where three or more branches meet.

Clarification that incoming currents are considered positive and outgoing currents are negative.

Introduction of an example to illustrate KCL with five currents meeting at a junction.

Equation setup for KCL: i1 + I3 + I4 = I2 + I5.

Description of a circuit diagram and the task to find currents ix, iy, and iz.

Application of KCL to a junction in a circuit diagram, balancing incoming and outgoing currents.

Solution for finding IX when iy equals 5A and iz equals 3A, resulting in IX = 10A.

Second condition: finding iy if IX equals 4A and iz equals 4 times iy, leading to iy = 2A.

Third condition: finding iz if IX equals iy equals iz, resulting in iz = -2A.

Discussion on solving complex networks using KCL, emphasizing the importance of identifying junctions.

Instructions on assuming initial voltage VKN equal to zero and unknown current direction.

Explanation of sign conventions for adding or subtracting voltages based on connection polarity.

Introduction of Ohm's Law (V = IR) and its implications for solving complex circuits.

Promise of future videos explaining how to apply KCL in more complex circuits.

Invitation for viewers to ask questions in the comments and request more videos.