How to Solve Advanced Cubic Equations: Step-by-Step Tutorial
Summary
TLDRIn this tutorial video, the presenter guides viewers through solving a cubic equation step by step. They begin by checking if x=1 and x=-1 are solutions using a rule involving the coefficients. After finding they are not solutions, they explore other potential solutions using synthetic division. The presenter divides the leading coefficient by the constant term and tests the resulting quotients. They find x=2 is a solution and then use synthetic division to derive a quadratic equation, solving it to find x=-3 and x=1/2. Finally, they verify the solutions by checking if they satisfy the original equation's conditions and conclude with a graph showing the cubic function's intercepts.
Takeaways
- 🔢 The original cubic equation is presented in a standard form, with coefficients a=2, b=1, c=-13, and d=6.
- 🔍 The tutorial first checks if x=1 and x=-1 are solutions to the equation using specific rules based on the coefficients.
- ❌ It is determined that x=1 is not a solution because the sum of the coefficients a+b+c+d does not equal zero.
- ❎ Similarly, x=-1 is also not a solution as the alternate coefficients do not sum to the same value.
- 🔑 The leading coefficient is labeled as Q and the constant term as P, with divisors of P and Q being considered for potential solutions.
- 🔄 A synthetic division process is used to test x=2 as a potential solution, which is found to be correct as the remainder is zero.
- 🔄 The synthetic division also helps in simplifying the cubic equation into a quadratic equation: 2x^2 + 5x - 3 = 0.
- 📐 The quadratic equation is factored into (x+3) and (2x-1), leading to additional solutions x=-3 and x=1/2.
- 🔍 The tutorial concludes by verifying the solutions (2, -3, 1/2) by checking if they satisfy the original equation's condition of -b/a.
- 📊 The final step includes a visual representation of the cubic function's graph, confirming the three x-intercepts as the solutions.
Q & A
What is the standard form of a cubic equation as mentioned in the video?
-The standard form of a cubic equation mentioned in the video is ax^3 + bx^2 + cx + d = 0.
What are the values of a, b, c, and d in the given cubic equation?
-In the given cubic equation, a is 2, b is 1, c is -13, and d is 6.
Why does the video suggest checking if x = 1 and x = -1 are solutions to the equation?
-The video suggests checking if x = 1 and x = -1 are solutions because they are easy to find and can be quickly verified using specific rules.
What rule is used to check if x = 1 is a solution to the cubic equation?
-The rule used to check if x = 1 is a solution is to add a + b + c + d and see if the result is 0. If it is, then x = 1 is a solution.
How does the video determine that x = 1 is not a solution?
-The video determines that x = 1 is not a solution by adding the coefficients (2 + 1 - 13 + 6) which equals -4, not 0, so x = 1 is not a solution.
What is the rule for checking if x = -1 is a solution to the cubic equation?
-The rule for checking if x = -1 is a solution involves adding the alternate coefficients of the equation. If the sum is 0, then x = -1 is a solution.
How does the video find the divisors of P and Q?
-The video finds the divisors of P (which is 6) and Q (which is 2) by listing all the positive and negative integer divisors for each.
What is synthetic division and how is it used in the video?
-Synthetic division is a method used to find the roots of a polynomial. In the video, it is used to test if x = 2 is a solution to the cubic equation.
How does the video derive the quadratic equation from the synthetic division results?
-After finding that x = 2 is a solution, the video uses the remaining coefficients from the synthetic division to form a quadratic equation, which is then solved to find the other solutions.
What are the final solutions to the cubic equation as determined in the video?
-The final solutions to the cubic equation are x = 2, x = -3, and x = 1/2.
How does the video verify the solutions found?
-The video verifies the solutions by adding them together and comparing the sum to -b/a from the original cubic equation. If they match, the solutions are confirmed.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video

Natural Cubic Spline Interpolation Example | Numerical Methods

MATRIKS RUANG VEKTOR | NILAI EIGEN MATRIKS 2x2 DAN 3x3
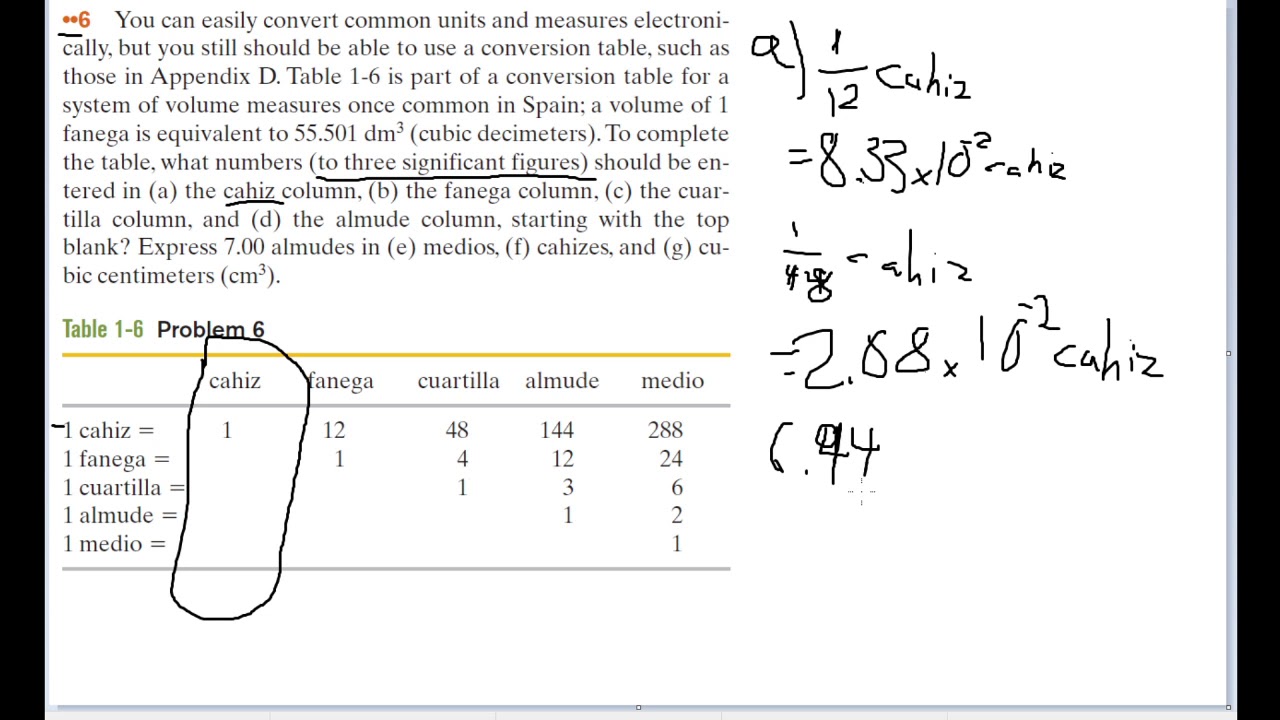
You can easily convert common units and measures electronically
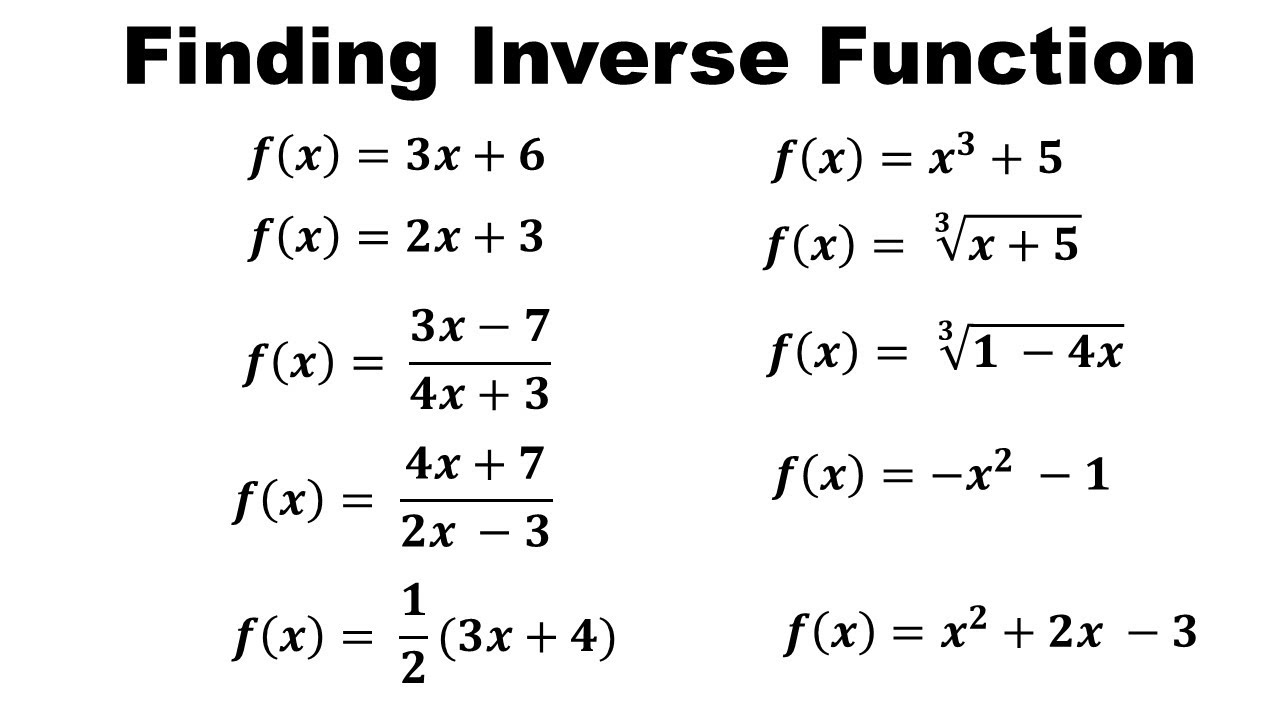
INVERSE FUNCTION // GENERAL MATHEMATICS // TAGALOG

Ecuaciones

Laju Reaksi • Part 6: Contoh Soal Persamaan Laju Reaksi dan Orde Reaksi
5.0 / 5 (0 votes)