Operation on Functions | Addition, Subtraction, Multiplication and Division of Functions

Summary

In this educational video, the host, referred to as 'teacher,' explains the operations and functions of rational expressions. The script covers adding, subtracting, and dividing functions, specifically focusing on two functions, f(x) and g(x). Through step-by-step examples, the teacher demonstrates how to combine and simplify these functions, including function composition and evaluation at a specific point. The video aims to clarify these mathematical concepts for beginners, encouraging viewers to engage with the material and simplify their answers for clarity.

Takeaways

• 📚 The video is an educational tutorial focused on operations and functions in mathematics.
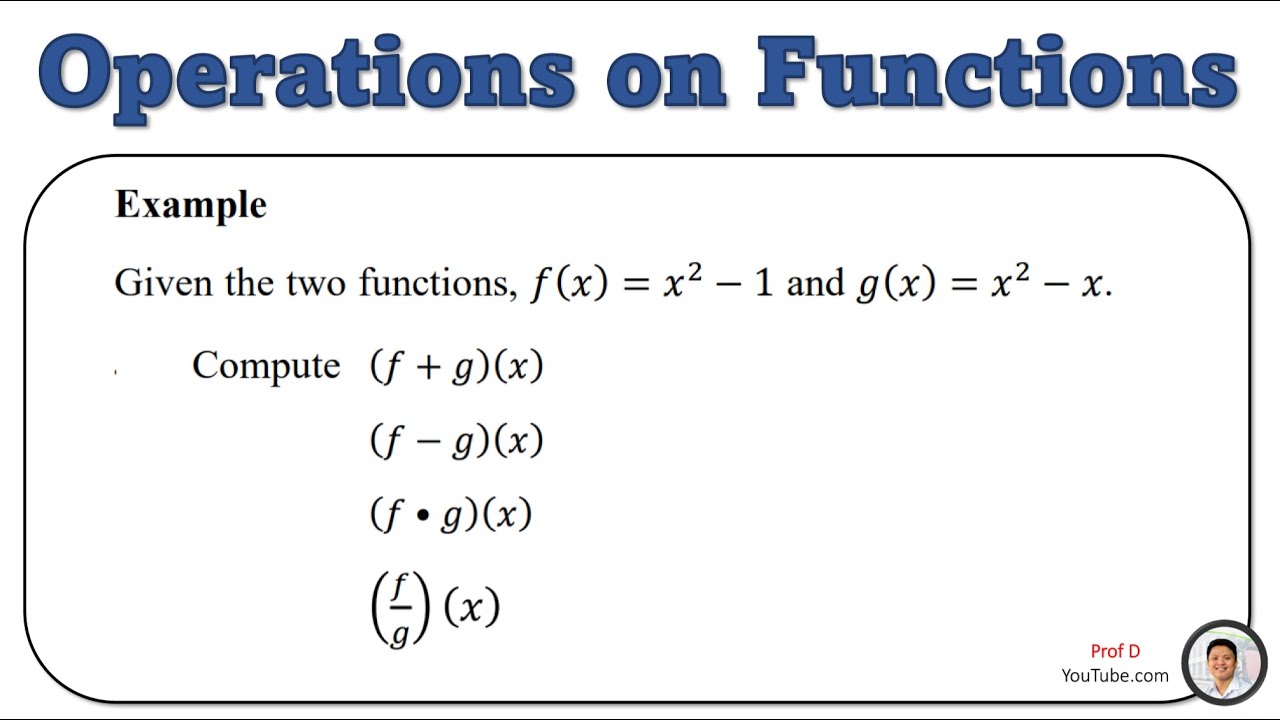
• 🔢 The script introduces two functions: f(x) = (x + 5) / (x - 7) and g(x) = 3 / (x - 7).
• ➕ The first operation discussed is the addition of functions f and g, resulting in f(x) + g(x) = (x + 8) / (x - 7).
• ➖ The second operation is the subtraction of g from f, leading to f(x) - g(x) = (x + 2) / (x - 7).
• 🔄 The third operation is the composition of functions, g(f(x)), which simplifies to 3(x + 5).
• 📉 The fourth operation involves evaluating g(f(x)) at a specific value, x = 2, resulting in g(f(2)) = 3/7.
• 📝 The importance of simplifying mathematical expressions is emphasized throughout the script.
• 👨‍🏫 The presenter, referred to as 'teacher', guides viewers through each step of the operations.
• 📹 The video script is repetitive, likely due to the nature of video recording, to ensure clarity.
• 📚 The script is designed for beginners in math classes, aiming to teach new techniques.
• 🔑 The video provides a step-by-step approach to combining and simplifying rational functions.
• 🌐 The video ends with a call to action for viewers to like, subscribe, and turn on notifications for more content.

Q & A

What is the main topic of the video?

The main topic of the video is the operation and function of mathematical expressions, specifically focusing on adding, subtracting, and dividing rational functions.

What are the two functions given in the video?

The two functions given are f(x) = (x + 5) / (x - 7) and g(x) = 3 / (x - 7).

What operation is performed in the first item of the video?

In the first item, the operation performed is the addition of the two given functions, f(x) and g(x).

How is the addition of f(x) and g(x) simplified in the video?

The addition is simplified by combining the numerators (x + 5 + 3) over the common denominator (x - 7), resulting in (x + 8) / (x - 7).

What is the result of f(x) + g(x)?

The result of f(x) + g(x) is (x + 8) / (x - 7).

What operation is performed in the second item of the video?

In the second item, the operation performed is the subtraction of g(x) from f(x).

How is the subtraction of g(x) from f(x) simplified in the video?

The subtraction is simplified by combining the numerators (x + 5 - 3) over the common denominator (x - 7), resulting in (x + 2) / (x - 7).

What is the result of f(x) - g(x)?

The result of f(x) - g(x) is (x + 2) / (x - 7).

What operation is performed in the third item of the video?

In the third item, the operation performed is the division of g(x) by f(x), which is also known as function composition.

How is the division of g(x) by f(x) simplified in the video?

The division is simplified by multiplying g(x) by the reciprocal of f(x), resulting in 3 * (x + 5) / (x - 7), which simplifies to 3x + 15.

What is the result of g(f(x))?

The result of g(f(x)) is 3x + 15.

What is the value of g(f(2)) as calculated in the video?

The value of g(f(2)) is calculated by first finding f(2) = -7/5 and then applying g(x) to this result, which gives g(f(2)) = 3/7.

What technique is used to simplify the final answer in the video?

The technique used to simplify the final answer in the video is to cancel out common factors in the numerator and denominator and to evaluate the functions at specific values when necessary.