What's a Tensor?

Summary

In this video, Dan Fleisch explains the concept of tensors using simple, relatable household items like children's blocks and arrows. He starts by reinforcing the understanding of vectors and their components, using basic examples like projecting vectors onto coordinate axes. He then explores how vectors can be considered tensors of rank one and moves into higher-dimensional tensors, demonstrating how tensors represent complex physical quantities such as forces within a solid object. Ultimately, Fleisch highlights the power of tensors in transforming consistently across reference frames, making them essential for understanding universal physical laws.

Takeaways

• 😀 Tensors are often misunderstood, and this video aims to explain them through simple household objects like blocks, arrows, cardboard, and a stick.
• 😀 Understanding vectors is key to understanding tensors. Vectors have both magnitude and direction, which represent various physical quantities.
• 😀 Vectors can represent more than just forces; they can also represent areas by adjusting the length and direction of the vector.
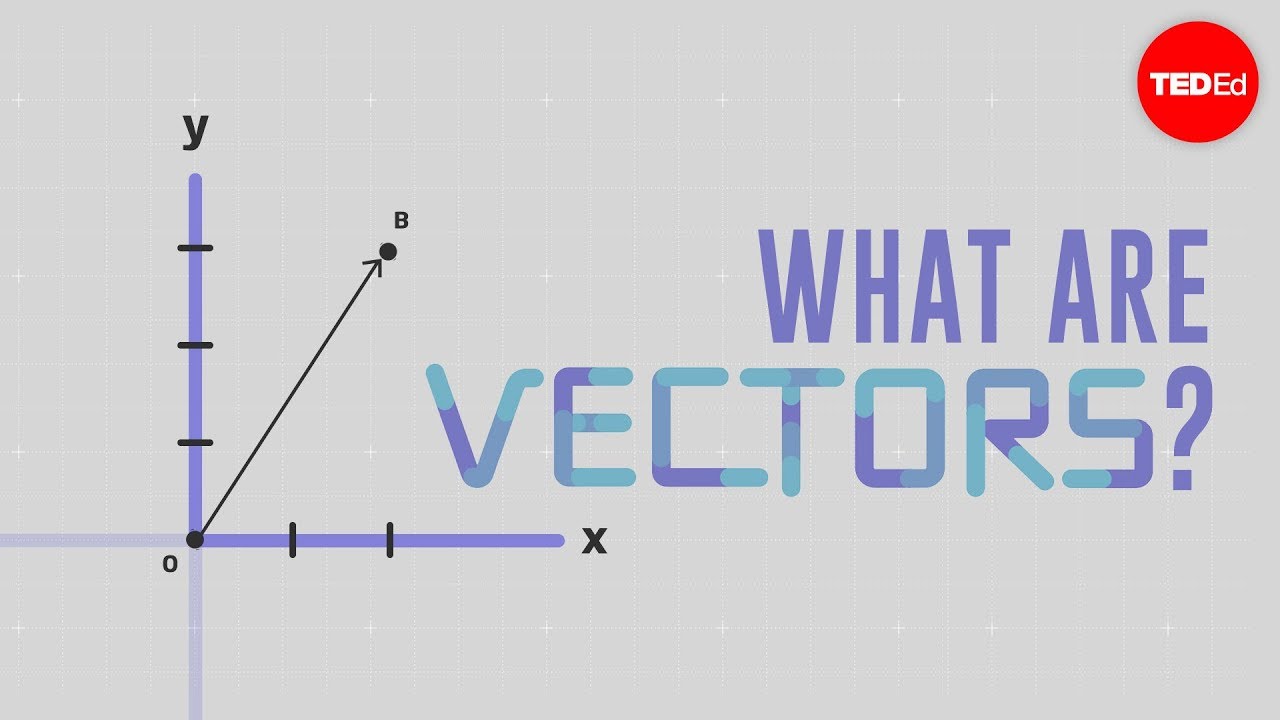
• 😀 A coordinate system, such as the Cartesian system, is essential to understanding vector components. It consists of the x, y, and z axes.
• 😀 Basis vectors (or unit vectors) represent the direction of the coordinate axes and are fundamental for finding vector components.
• 😀 Vector components are obtained by projecting a vector onto the axes of the coordinate system. This allows you to determine how much the vector lies along each axis.
• 😀 By breaking down a vector into its components, you can visualize it as a combination of basis vectors, each with a corresponding scalar coefficient.
• 😀 Vectors are considered tensors of rank one, as they require one index for each component (e.g., Ax, Ay, Az).
• 😀 Scalars are tensors of rank zero, as they have no directional components and are represented by a single value.
• 😀 Higher-rank tensors (e.g., rank-two and rank-three tensors) involve multiple indices and components, which are necessary to represent more complex physical quantities like forces inside a solid object.
• 😀 Tensors are powerful because the combination of components and basis vectors remains the same across all reference frames. This property makes tensors invariant and universally applicable in physics.

Q & A

What is the main goal of this video?

The main goal of the video is to explain what a tensor is, using simple, everyday objects like children's blocks, small arrows, cardboard, and a pointed stick, without relying on complex mathematical equations.

How does the video define a vector?

A vector is defined as an arrow that represents a quantity with both magnitude and direction. The length of the arrow is proportional to the magnitude, and the direction of the arrow indicates the direction of the quantity.

How can a vector represent an area?

A vector can represent an area by making its length proportional to the size of the area (in square meters) and orienting the arrow perpendicular to the surface of the area.

What are coordinate systems and how are they relevant to vectors?

Coordinate systems, like the Cartesian coordinate system, provide a reference framework where vectors are represented. These systems come with basis vectors (unit vectors) that point in the direction of the coordinate axes, which help in defining the components of vectors.

What is the role of unit vectors in vector representation?

Unit vectors, such as x-hat, y-hat, and z-hat, have a length of one and represent the direction of the coordinate axes in a given system. They are used to determine the components of a vector along the respective axes.

How can the components of a vector be found using projections?

The components of a vector can be found by projecting the vector onto the coordinate axes. The shadow cast by the vector onto each axis gives the respective component (x-component or y-component).

What does the process of finding vector components involve?

To find the components of a vector, you project the vector onto each axis (e.g., the x-axis and y-axis) and measure the length of the projections. This gives you the scalar values that represent the vector's components along each axis.

What does the video mean by vectors being tensors of rank one?

Vectors are considered tensors of rank one because they have one index for each of their components (e.g., A_x, A_y, and A_z for a 3D vector). This indicates they belong to a class of objects with directional indicators.

What is the significance of tensors having components and basis vectors?

The combination of components and basis vectors allows tensors to represent physical quantities in a way that is independent of the coordinate system, which makes them useful for transforming between different reference frames while maintaining consistency.

What is a rank-two tensor, and how is it represented?

A rank-two tensor in three-dimensional space has 9 components and 9 sets of two basis vectors. It can represent things like forces acting on different surfaces within a solid object, where each component refers to the force in a specific direction on a specific surface.

How are higher-rank tensors represented in three-dimensional space?

Higher-rank tensors, such as a rank-three tensor, have multiple components and more indices. In 3D space, a rank-three tensor has 27 components, each associated with 3 basis vectors, and its components are indexed with three indices.

What makes tensors powerful, according to the video?

Tensors are powerful because their combination of components and basis vectors remains consistent across different reference frames. This allows all observers to agree on the physical quantities described by the tensor, regardless of their individual perspectives.

Why are tensors referred to as 'the facts of the universe'?

Tensors are called 'the facts of the universe' because they transform in a way that preserves their physical meaning across different reference frames. This makes them a fundamental tool for understanding and describing the physical world in a consistent manner.